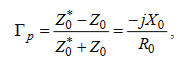The problem you have is that you are applying all the results of traveling waves on transmission lines with real characteristic impedance to a problem where the base impedance is complex. The normal reflection coefficient when \$Z_0\$ is real:
\$\Gamma_L = \frac{b}{a} = \frac{Z_L-Z_0}{Z_L+Z_0}\$ eqn(1)
represents the ratio of the reflected to forward waves and \$|\Gamma|^2\$ is their power ratio.
When \$Z_0\$ is complex, this is no longer true. Read, for example:
S. Llorente-Romano, A. Garca-Lampérez, T. K. Sarkar and M. Salazar-Palma, "An Exposition on the Choice of the Proper S Parameters in Characterizing Devices Including Transmission Lines with Complex Reference Impedances and a General Methodology for Computing Them," in IEEE Antennas and Propagation Magazine, vol. 55, no. 4, pp. 94-112, Aug. 2013, doi: 10.1109/MAP.2013.6645145.
It's complicated, but it's easy to see the problem, as at conjugate match with the normal definition of reflection coefficient,
which is not zero if \$X_0\$ is not zero. You need to work through the maths to see that when the base impedance is complex, the reflection coefficient defined in eqn(1) does NOT represent the ratio of reflected power to forward power. In the paper above, then define something they call power wave scattering parameters, and these are much the same except that the reflection coefficient is defined as:
\$\Gamma = \frac{Z_L-Z_0^*}{Z_L+Z_0}\$
and they show that this has similar characteristics as the one we know well when \$Z_0\$ is real. Clearly when the load is conjugately matched then \$\Gamma=0\$ and it behaves as we expect.
Read the paper, it's too involved to reproduce here.