A thermal relief pad is essentially a pad which has fewer copper connections to a plane (such as a ground plane).
A normal pad would simply be connected in all directions, with the solder mask exposing the area to be soldered. However the copper plane then serves as a giant heatsink which can make soldering difficult, because it requires that you keep the iron on the pad longer and risk damaging the component.
By reducing the copper connections, you limit the amount of heat transmission to the plane. It follows of course, that with reduced copper conduction paths, you also have greater electrical resistance. The increase in resistance is marginal compared to the reduction in thermal conductivity.
This should not be a concern unless the pad is carrying high current such that the four traces (on a standard thermal relief) together are insufficient to carry the current; or if it is for high frequency signals where the thermal relief may cause unwanted inductance.
Just to show a visual on normal vs thermal relief pads:
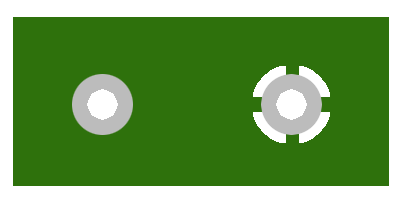
The pad at left is connected to the copper plane (green) in all directions whereas the pad at right has had copper etched away such that only four "traces" connect it to the plane.
Just for fun, I used a trace resistance calculator to estimate what the electrical resistance difference might actually be.
Consider the thermal relief pad. If we assume the four "traces" to be 10 mil wide (0.010") and approximately 10 mil in length from the pad to the plane, then each of them has a resistance of about 486μΩ.
The four "resistors" in parallel would give us a total resistance of :
$$R_{total} = \frac{1}{\frac{1}{486\mu\Omega} \cdot 4} = \frac{486\mu\Omega}{4} = 121.5 \mu \Omega$$
If we approximate the empty space created by the thermal relief to have the equivalent of about three such traces, giving us 16 in total:
$$R_{total} = \frac{486\mu\Omega}{16} = 30.375 \mu \Omega$$
Remember these values are micro ohms or \$0.0001215\$ and \$0.000030375\$ ohms, respectively. So by rough estimate, the difference in electrical resistance between our two hypothetical pads is a mere 91.125μΩ.
The thermal properties, on the other hand, are significantly different. I don't know thermal conductivity formulas very well, so I won't try to calculate it. But I can tell you from experience that soldering one versus the other is highly noticeable.
Values calculated assuming a 1 oz copper layer.
