Here is my task:
Periodical signal is given (only one period of signal is shown):
[![enter image description here][1]][1]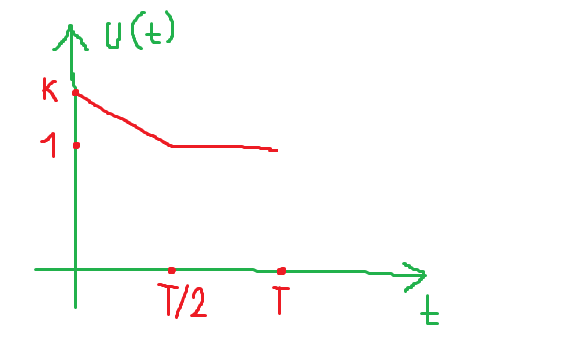
Calculate value of voltage K so that average power of this signal equals 1.5W.
Power of signal can be calculated as $$P=\int_{0}^{T}u^{2}(t)dt$$.
My idea is to express u(t) and solve equation $$\int_{0}^{T}u^{2}(t)dt=1.5$$ (after solving integral I will get some function of k, f(k), and solve f(k)=1.5).
But u(t) is piecewise defined, it is k+2*(1-k)*t/T in interval 0 to T/2 and 1 in interval T/2 to T. What to do here? [1]: https://i.sstatic.net/sO5gs.png
