Vin1 = -Vin2 = var (differential mode). If we vary both input voltages in an opposite direction with the same rate, the local voltages inside the resistor will gradually decrease from the higher to the lower voltage. Because the two voltages are of opposite polarity, a point of zero voltage will appear inside the middle of the resistor; this is the famous "virtual ground". So the input is grounded with its middle point. Twice as much voltage is applied to the resistor, the current is twice as much, and the resistance "seen" by each of the input sources is half the total resistance of the resistor.

I have set an offset (input common-mode) voltage of 5 V and AC (differential) voltage of 100 mV. The phase of Vin2 is 180° (inverted). The output common mode voltage is 9 V.

Conclusion

Vin1 = -Vin2 = var (differential mode). If we vary both input voltages in an opposite direction with the same rate, the local voltages inside the resistor will gradually decrease from the higher to the lower voltage. Because the two voltages are of opposite polarity, a point of zero voltage will appear inside the middle of the resistor; this is the famous "virtual ground". So the input is virtually grounded with its middle point. Twice as much voltage is applied to the resistor, the current is twice as much, and the resistance "seen" by each of the input sources is half the total resistance of the resistor.

I have set an offset (input common-mode) voltage of 5 V and AC (differential) voltage of 100 mV. The phase of Vin2 is 180° (inverted). The output common mode voltage is 9 V.

An experiment with a real ground

If, after the written above, you are not convinced of the need for a "movable ground", I suggest you check it experimentally by connecting the common emitter point E to the real ground.

Differential mode

First explore the original (ungrounded) differential pair by setting the voltage of the common emitter point E equal to zero. To do this, set the offset of both input voltage sources to about 0.8 V (I have adusted it to 0.758 V).

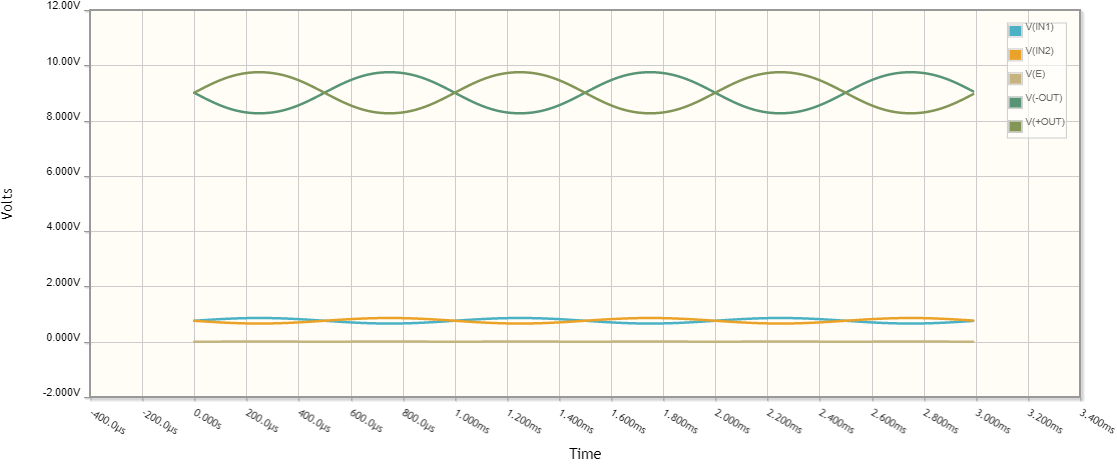
Then connect the point E to the real ground.

^{simulate this circuit}

As you can see there is no difference between the two graphs. The two transistors work as common emitter stages with really grounded emitters. The conclusion is that the pure differential mode allows grounding of the emitter point E.

Differential + common mode

Now set 5 V offset (common mode) voltage to both input voltage sources. The result is obvious - because of the excessive base-emitter voltages both transistors are saturated. The conclusion is that the common mode does not allow grounding of the emitter point E; it needs a "flexible" ground.

Conclusion

The idea of ​​the differential input is to drive it simultaneously on both sides using two grounded (single-ended) voltage sources. Actually, they are connected (through the ground) in series; so their voltages are summed (subtracted) according to KVL and the total voltage is applied to the resistor as in the first picture. From another perspective, you can think of the floating input source in the first figure above as being split into two "sub-sources".

So in a differential stage (which is the op-amp input stage) there is no fixed ground. All three cases are possible - there is no ground at all, there is a real ground (zero) or it is shifted (in a positive or negative direction).

Above we drove a grounded device input by a grounded input voltage source. But what do we do if the input is floating and we still want to drive it with a grounded input source? How do we drive a floating input with a grounded source?

We can apply another clever trick - we can "split" the floating source in the first figure above and ground the midpoint between the two "sub-sources".

Thus we arrive at the idea of ​​differential input - to drive the input simultaneously on both sides using two grounded (single-ended) voltage sources. Actually, they are connected (through the ground) in series; so their voltages are summed (subtracted) according to KVL and the total voltage is applied to the resistor as in the first picture.

In a differential stage (which is the op-amp input stage) there is no fixed ground. All three cases are possible - there is no ground at all, there is a real ground (zero) or it is shifted (in a positive or negative direction).