Differential vs static resistance
The zero differential resistance Rdiff = dV/dI of voltage sources and the infinite differential resistance of current sources are intrinsic properties, unaffected by whether the circuit is undergoing small-signal or large-signal analysis. These properties are always exhibited as long as there is a change in the input signal; hence the name "differential". At the same time, they have some "static" resistance R = V/I.
Experiments
This can be experimented in a simple way by connecting two different sources - one voltage and the other current - to each other. By varying the quantity produced by one of them, we can determine the differential resistance of the other.
Current source
Let's first supply the load through a current source...
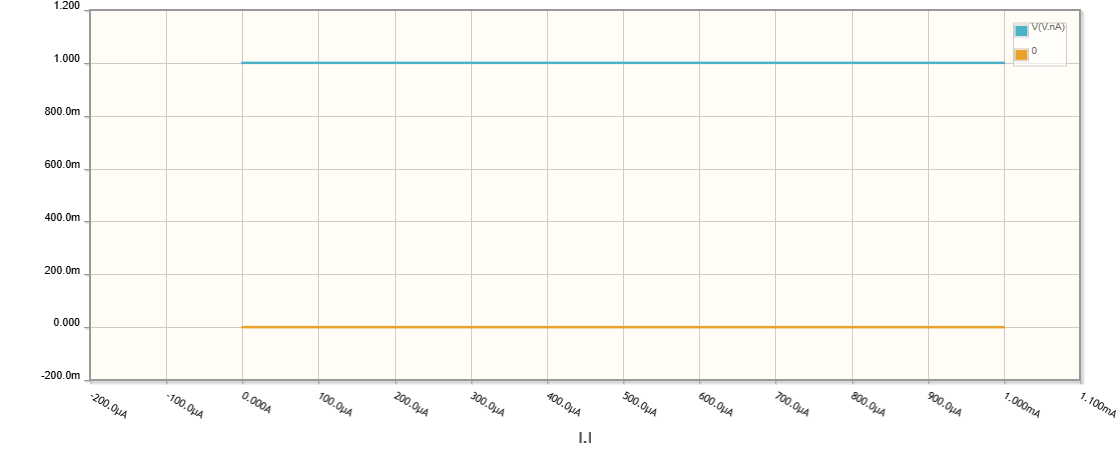
Voltage source as a load: ... and let the load be a voltage source. The static resistance is R = V/I = 1/1 = 1 kΩ. Figuratively speaking, the current source "sees" a 1 kΩ "resistor".
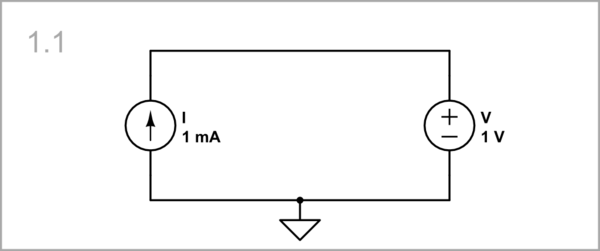
simulate this circuit – Schematic created using CircuitLab
Because the voltage does not change when the current changes, the differential resistance Rdiff = dV/dI = 0/1 = 0. The current source "sees" an AC short circuit.
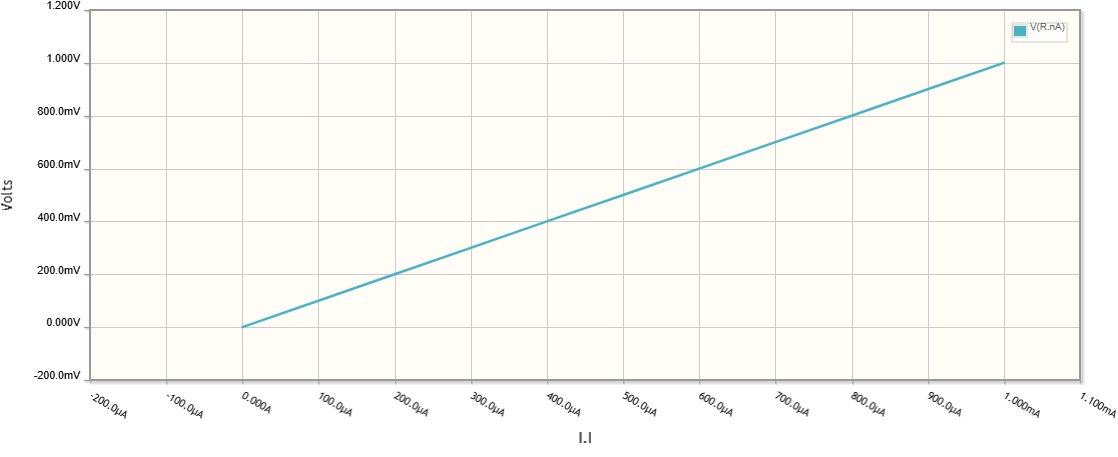
Resistor load: In this case, the differential resistance is identical to the static resistance - Rdiff = dV/dI = R = V/I = 1 kΩ. The voltage source "sees" a 1 kΩ resistor.
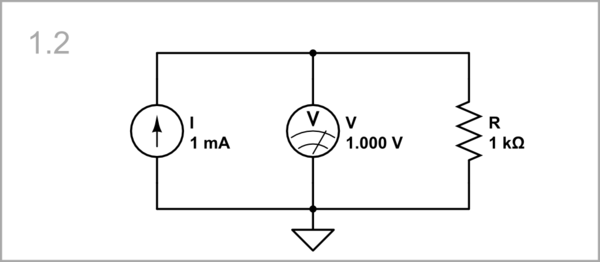
The voltage is proportional to current (Ohm's law).
Voltage source
Now let's supply the load through a voltage source...
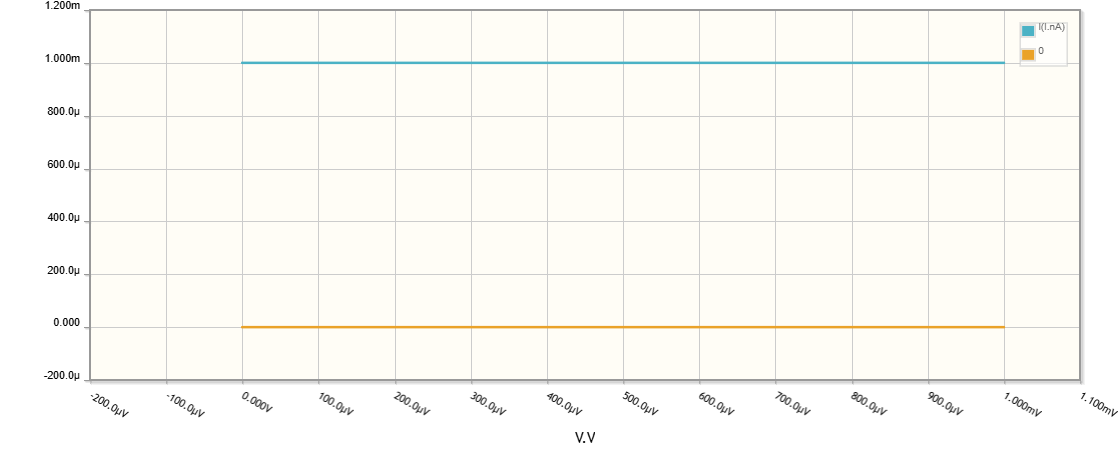
Current source as a load ... and let the load be a current source. The static resistance is again R = V/I = 1/1 = 1 kΩ. The voltage source "sees" a 1 kΩ "resistor".
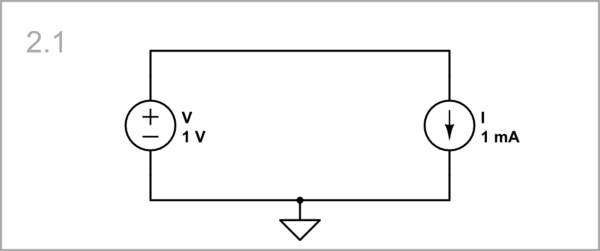
Because the current does not change when the voltage changes, the differential resistance Rdiff = dV/dI = 1/0 -> ∞. The voltage source "sees" an AC open circuit.
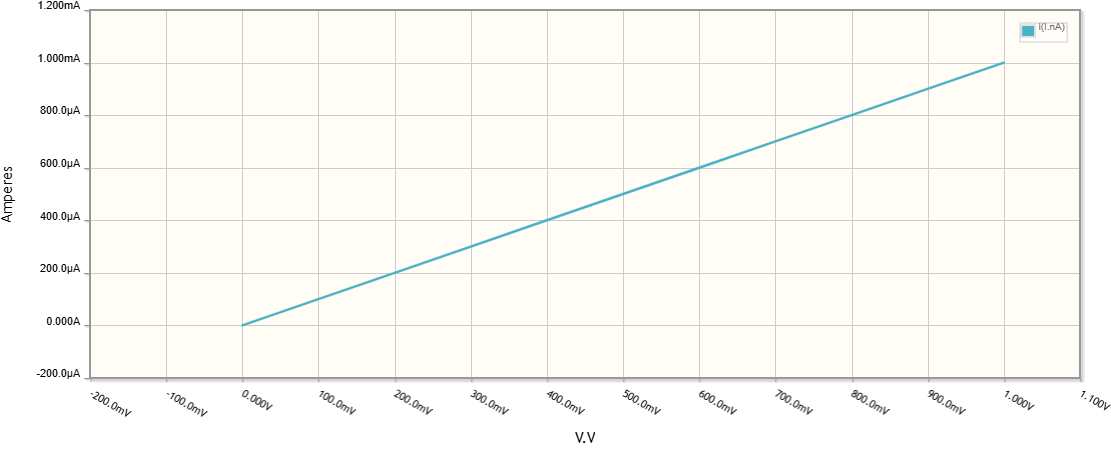
Resistor load: As above, in this case, the differential resistance is identical to the static resistance - Rdiff = dV/dI = R = V/R = 1 kΩ. The voltage source "sees" a 1 kΩ resistor.
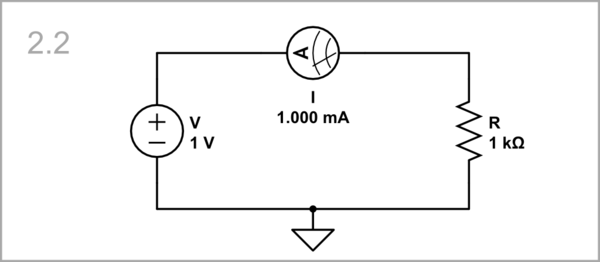
The current is proportional to voltage (Ohm's law).