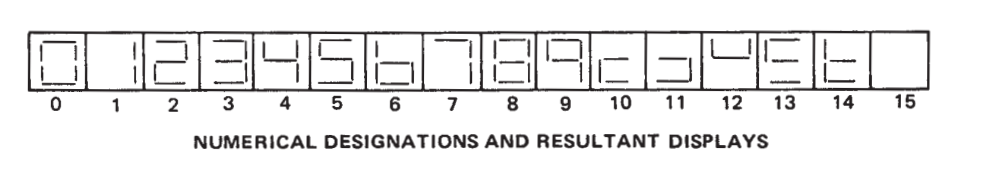
TyeThe 7447 and 7448 are BCD-to-seven-segment display drivers. They are not fully decoded (saving transistors was important in the ~1960s), so invalid inputs provide oddball outputs rather than blanking or showing some semblance of a hex character. Valid BCD inputs are 0x00 .. 0x09, and 0x0A to 0x0F are invalid. From this datasheet:
The display for each input code is unique (assuming zero blanking is not used) so you can actually read the binary number directly, although it's not very user-friendly until you memorize the top 6 symbols including blank. But I doubt that's the point of the exercise.
If you intend to show the result in hex format you would need to make or find a HEX-to-seven-segment display driver or decoder. If you intend to show the result in decimal digits (from 0 to 225 in this case) you would need to interpose a logic circuit between the multiplier output and the BCD display drivers to convert 8 bit binary to 3 digit BCD. I suspect the object of your assignment is the latter.
One such method is the 'add 6' algorithm.