I'm working in my Electrical Circuits homeworks. One of the exercises says: "Use the Fourier Transform method to calculate $v_0(t)$"\$v_0(t)\$" The circuit is this:
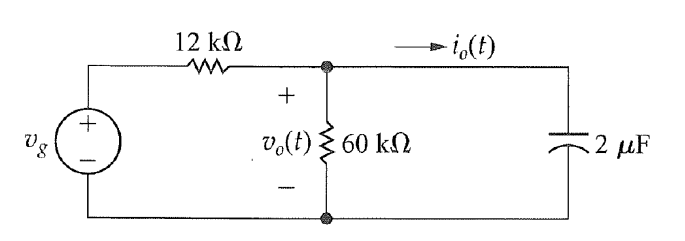
where $v_g=36sgn(t)$\$v_g=36sgn(t)\$, and $sgn(t)$\$sgn(t)\$ is the sign of $t$\$t\$ (it returns 1, -1 or 0). Well, my problem is the following. I consulted my table of Fourier Transforms and I found that $$V_g(\omega)=36\cdot \dfrac{2}{j\omega}$$ However, I don't know how to calculate $\omega$\$\omega\$. I need it in order to calculate the $V_g$\$V_g\$ and also $Z_C$\$Z_C\$. I know that, if, for example $v_g(t)=A\sin(kt)$\$v_g(t)=A\sin(kt)\$ then $k=\omega$\$k=\omega\$. But in this case I'm completely lost.
How can I do it? Thanks in advance!
