1
\$\begingroup\$

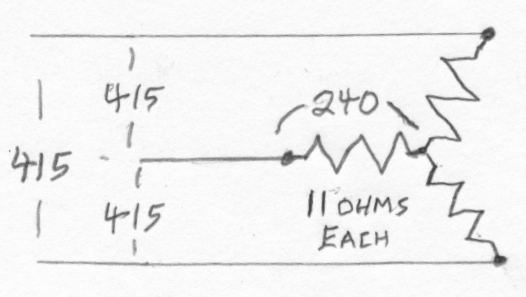
Is there a simple way of calculating the power (kW) of a 3 phase heating element?

I have 3 elements mounted in a pack and inserted into a tube and there are 12 tubes, so 36 elements in total. They are connected in delta. I have 22ohms between L1+L2, L2+L3 and L3+L1.

\$\endgroup\$
4
  • 2
    \$\begingroup\$ Welcome to EE.SE! Did you measure 22 ohm between L1 and L2 while other elements was still connected between L2 and L3 and L3 and L1? In that case you have 33 ohm between each phase. Also, what is your phase to phase voltage? \$\endgroup\$
    – winny
    Commented May 19, 2020 at 15:18
  • \$\begingroup\$ Hi Winny. I measured between L1 and L2 etc with the rest all disconnected. These are approximately 6000mm long and running 415v delta. \$\endgroup\$
    – Can you smell burning
    Commented May 19, 2020 at 15:36
  • \$\begingroup\$ You should try to explain more clearly how the elements and tubes are connected to get the final L1, L2 and L3 connection. If a delta has been created, I can not picture how you could have measured resistance between L1 and L2 etc. with the rest disconnected. As a result, you seem to have one assumption on that as a comment and two more as answers. \$\endgroup\$
    – user80875
    Commented May 19, 2020 at 17:10
  • \$\begingroup\$ Ok. Then disregard 33 ohm. \$\endgroup\$
    – winny
    Commented May 19, 2020 at 17:10

2 Answers 2

Reset to default
1
\$\begingroup\$

They are connected in delta

That's a 22 ohm resistor connected to each line voltage.

I measured between L1 and L2 etc with the rest all disconnected. These are approximately 6000mm long and running 415v delta

The power for each 22 ohm resistor is \$V_{LINE}^2/R\$. This equals 7.828 kW.

The total power is therefore 3 x 7.828 kW = 23.485 kW

\$\endgroup\$
3
  • \$\begingroup\$ Thank you all for your comments. My understanding of mathematics and equations is shameful. I'm trying to understand the equation above. The answer makes sense and tallys with what i was expecting to see, just not sure how we got there. So V2Line is 22ohms squared? then divide by the resistance? Might need to be walked through this if you have the patience? \$\endgroup\$
    – Can you smell burning
    Commented May 20, 2020 at 7:04
  • \$\begingroup\$ The power per phase is \$\dfrac{V^2}{R}\$ hence 415*415/22 = 7828 watts. Then. because there are three phases, the total power is 3*7828 watts. \$\endgroup\$
    – Andy aka
    Commented May 20, 2020 at 8:05
  • \$\begingroup\$ That makes sense, thank you. \$\endgroup\$
    – Can you smell burning
    Commented May 20, 2020 at 10:34
0
\$\begingroup\$

Once you have all of the resistors connected in a balanced load, you don't really need to know how they are really connected, you can assume they are wye connected. If you measure 22 ohms from terminal to terminal and apply balanced, 3-phase, 415 volts from terminal to terminal, you have the equivalent of 240 volts across each of three, 11-ohm resistors. Using the voltage squared divided by the resistance, you have 5236 watts in each of three resistors for a total of 15,708 watts.

enter image description here

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.