How to understand EIRP, PSD for a given bandwidth?

Question

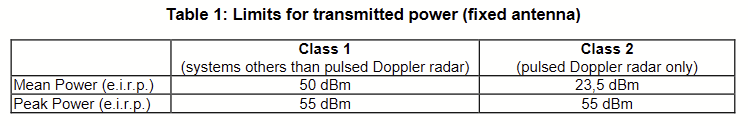
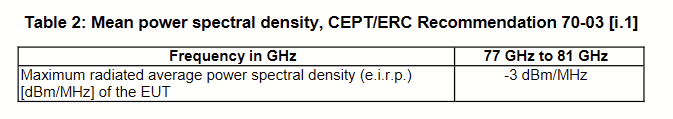
I have troubles to understanding what is the meaning of parameters for transmitting power of RF-signal.

So for RF signal in the same frequency band and same application, on one hand I see the max. allowed mean power is e.g 50dBm. On the other hand I see another max. allowed power spectral density is e.g -3dBm/MHz. This really confuses me.

So the questions are:

1. What is the relation between mean power and power spectral density? How to convert one to the other ?

2. For e.g. if my device uses a 2GHz bandwidth, how do I know how much power the device is allowed to transmit ? How to calculate it ?

Thanks very much.

BL

Answer 1

If your Power Spectral Density is -0.3 dBm / MHz that's a real power of 0.5 milliwatts per MHz hence, if your bandwidth is 2000 MHz, that's a total permissible power of 1 watt across the whole width of the spectrum you are allowed to use.

If you are allowed to use a signal of 50 dBm (100 watts) AND, you must simultaneously ensure that it doesn't exceed the PSD of -0.3 dBm / MHz, you have to consider the signal bandwidth. If the bandwidth is 5 Hz, that's 100 watts in 5 Hz and, equivalent to 0.5 milliwatt spread over 1 MHz.

Answer 2

What is the relation between mean power and power spectral density? How to convert one to the other ?

The integral over the PSD is the power. That's it.

For e.g. if my device uses a 2GHz bandwidth, how do I know how much power the device is allowed to transmit ? How to calculate it ?

2 GHz · -3 dBm/MHz = 33 dB + -3 dBm = 30 dBm = 1 W.

It's forbidden, because you mustn't transmit more than -50 dBm on average. So, you're bound by both limits, and either one might kick in.

You might, however, transmit a 1 ns pulse with a power of 1W, then be silent for -50 dBm - 30 dBm = -80 dB of a nanosecond, so -10 dBs = 0.1s

More realistically, your PSD won't be flat – you're doing anything but a pulsed radar, after all. In that case, assigning of power might be implicit e.g. through transmitter symbol pulse shaping, or explicit, e.g. through subcarrier power allocations according to subcarrier SNR, for example employing the waterfilling algorithm.