0
\$\begingroup\$

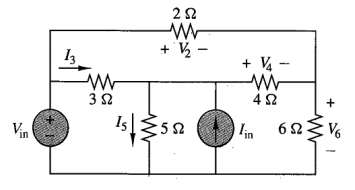
enter image description here

Given the power absorbed by each resistor, and the resistance value - do I have enough information to solve the circuit?

I found the Voltage Supply value by \$v^2=pr\$ but I'm not sure how to find the currents for \$V_{in}\$, or the branch currents... I created 4 mesh equations - the three along the bottom, and one along the edge . but since I dont know \$V_{in}\$ I can't create my matrix to solve for \$V_{k}\$.

EDIT: I am very aware of \$p=iv\$ and \$v=ir\$

I am able to find individual Voltages and Currents but not what the values of \$V_{in}\$ and \$I_{in}\$ are. I'm not sure how to tell what the direction of these currents are. I'm trying to find out if the \$I_{in}\$ absorbing or delivering power - to dot that, do I need to know the directions? To find \$V_{in}\$ can I sum the individual currents? \$v=\sqrt{p_{1} r_{1} + p_{2} r_{2} \cdots p_{n} r_{n}}\$. What do I do with the current source?

\$\endgroup\$
4
  • \$\begingroup\$ If you know the power and resistance for each resistor then you know the voltage across and the current through each resistor. That's plenty of information. \$\endgroup\$
    – Pete Becker
    Commented Sep 25, 2015 at 19:46
  • \$\begingroup\$ Yeah! Power and resistance for each resistor is enough. I hope you know that here: P = I^2 R = (V^2)/R = V*I \$\endgroup\$
    – Rahul Behl
    Commented Sep 25, 2015 at 19:52
  • \$\begingroup\$ Now what is that really complicated theory.... oh yeah ohms law. You can even solve it by not knowing the powers dissipated by each resistor. \$\endgroup\$
    – Andy aka
    Commented Sep 25, 2015 at 20:24
  • \$\begingroup\$ I'm a tiny bit confused. What other information were you given? Were you actually told what the power dissipated in each resistor was? If so, why isn't that information part of your question? \$\endgroup\$
    – Dwayne Reid
    Commented Sep 25, 2015 at 23:11

1 Answer 1

Reset to default
1
\$\begingroup\$

Using the mesh or node equations, you should be able to determine each of the currents and voltages in the circuit in terms of \$V_{in}\$ and \$I_{in}\$. This will give you 10 equations of the form

$$V_j = f_j\left(V_{in},I_{in}\right)$$ and $$I_k = g_k\left(V_{in},I_{in}\right)$$

Now you say you are given the power consumed by each resistor, which means a set of equations of the form

$$V_nI_n=P_n$$

where the \$P_n\$ are known values.

This will be more than enough equations to be able to determine \$V_{in}\$ and \$I_{in}\$. All you need to do is pick the most convenient couple of equations and do some algebra.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.