From my EM theory book:
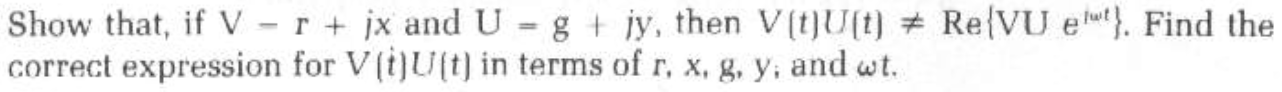
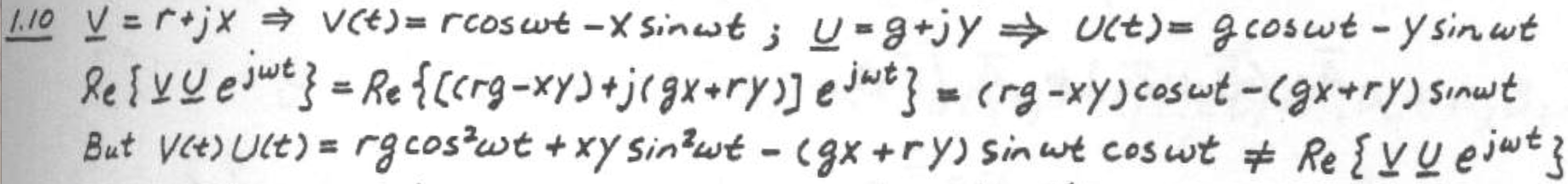
I have the solution for this problem, but I don't even understand the first step. How does \$V = r + jx \implies v(t) = rcos(wt) - xsin(wt) \$??
The formula to convert from the phasor representation \$V=r+jx\$ to its time-domain equivalent is this:
\$ V(t) = Re\{V \, e^{\,j\omega t}\} \$
Using the complex identity:
\$ e^{\,j\omega t} = \cos(\omega t) + j\,\sin(\omega t) \$
You obtain:
\begin{align*} V(t) &= Re\{V \, e^{\,j\omega t}\} = Re\{ (r+jx) \cdot [\cos(\omega t) + j\,\sin(\omega t)] \} = \\[1em] &= Re\{ r \cos(\omega t) - x \sin(\omega t) + j[...] \} = \\[1 em] &= r \cos(\omega t) - x \sin(\omega t) \end{align*}