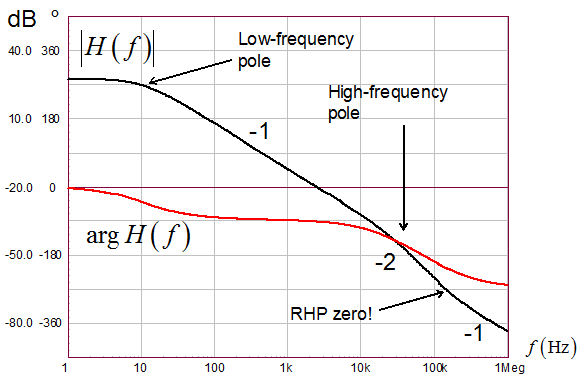
I may be a bit off here, but I think you are trying to find out where the definition for pole/zero match with the 20dB/dec and phase shift.
The answer is actually that the position of the pole heavily influences the transfer function. Poles can be anywhere on the complex plane. And if you get close to those poles (on the complex plane), then you will indeed see that you will get to infinity there.
However, a bode plot, transfer function or anything similar doesn't cover that whole complex plane. It will stay on the imaginary axis! And so unless your poles are exactly on the imaginary axis, you won't see any point going to infinity.
The following figure illustrates this with the transfer function:
\$TF(s)=\frac{1}{1+s+s^2}\$
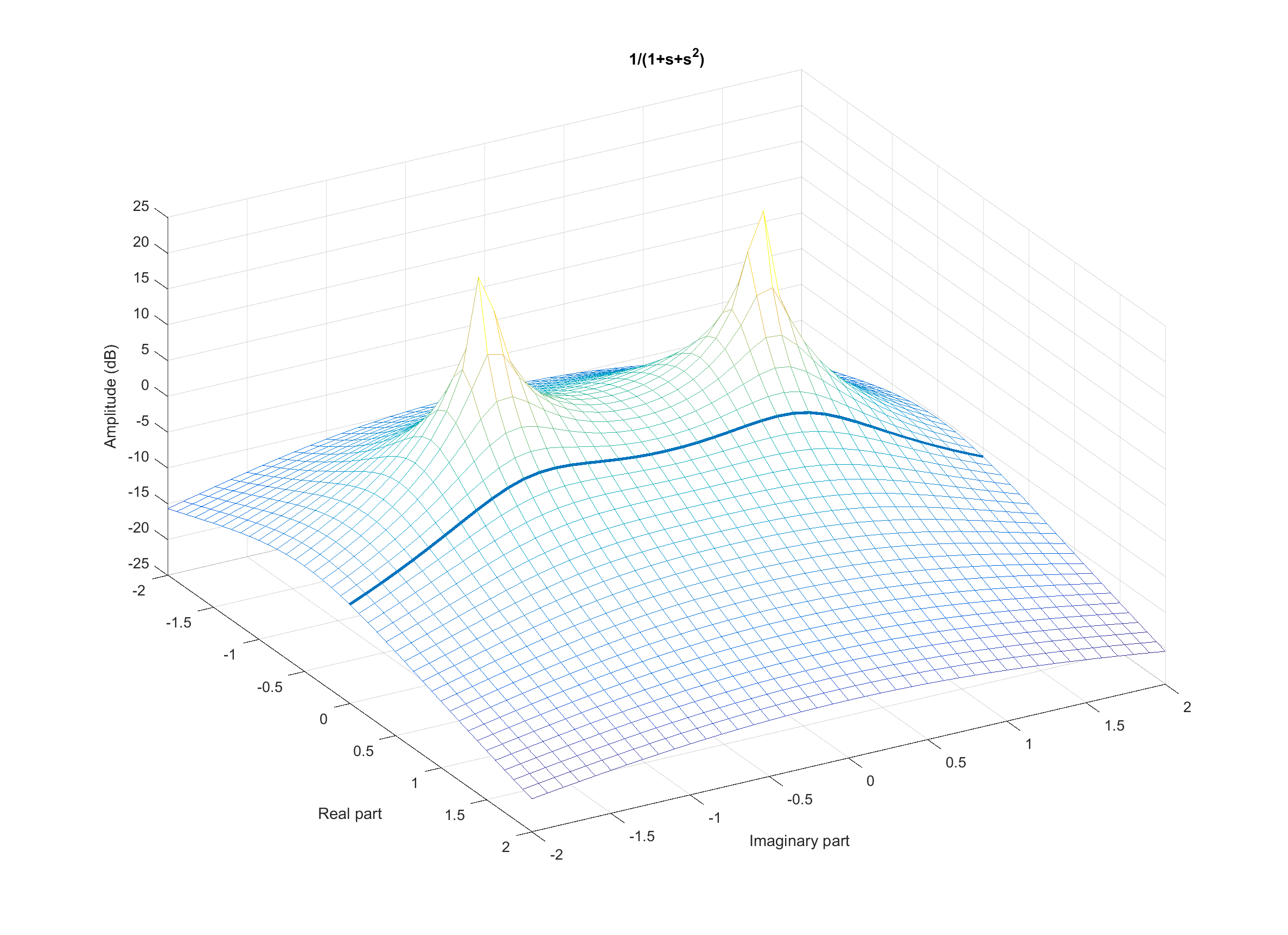
The bold line is the frequency axis that you use for bode plots or transfer functions. But you see that it "misses" the poles completely.
What is possible though, is to describe behavior far away from the poles when going along the frequency axis. Let's take a simple transfer function 1/(1+s) and follow it along the frequency axis:
\$\left|\frac{1}{1+j\omega}\right|=\frac{1}{\sqrt{1+\omega^2}}\$
If \$\omega\$ becomes much larger than the pole frequency, then
\$\frac{1}{\sqrt{1+\omega^2}} \overset{\omega\rightarrow +\infty}{\approx} \frac{1}{\omega}\$
If \$\omega\$ is multiplied by 10, then the amplitude will decrease by a factor of 10, which is 20dB, and so you get -20dB/decade. You can make the same approximation for the phase of the transfer function. A Left-Half Plane pole will add 90 degrees phase shift as you increase \$\omega\$ beyond the pole frequency.
The same reasoning applies to LHP zeros (eg. \$TF(s)=1+s\$), where you will see that, as \$\omega\$ increases, similar things happen to the amplitude and phase, albeit in another direction (+20dB/decade rather than -20dB/decade, and -90 degrees rather than +90 degrees).
Doing the same analysis for RHP poles and zeros will lead to the same conclusions for the amplitude, but they lead to inverted phase shifts.