0
\$\begingroup\$

I'm doing some homework for my circuitry class and I'm stuck.

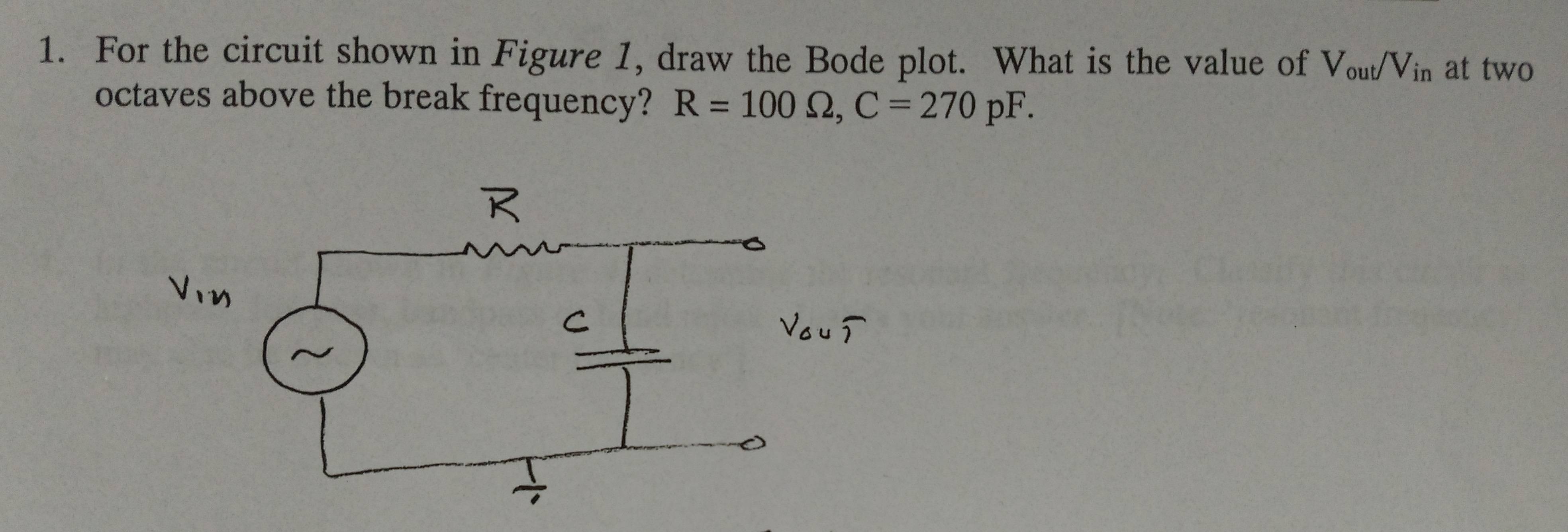
I have to find the value \$\frac{V_{in}}{V_{out}}\$ of a low pass filter, the schematic of which I have posted below. enter image description here

I got the break frequency, which I have shown below, but I do not know how to find the value of \$V_{in}\$ or \$V_{out}\$. I am also confused by what an octave is, but I think it is adding the same frequency per octave.

enter image description here

Any help would be appreciated!

\$\endgroup\$
1
  • \$\begingroup\$ * you know the voltage ratio of a 2 resistor divider? substitute the impedance of the cap(Zc=1/ωC) for R. The calc. method is exactly the same. \$\endgroup\$
    – D.A.S.
    Commented Dec 4, 2017 at 1:14

1 Answer 1

Reset to default
1
\$\begingroup\$

An octave is twice (or half) the frequency away from a given point. Eg an octave higher than 100Hz is 200Hz.

You do not need to know the exact value of \$V_{out}\$ or \$V_{in}\$, just the ratio and the transfer function can determine this.

  1. Derive the transfer function.
    This is a simple 1st order filter \$\frac{V_{out}}{V_{in}} = \frac{\omega_o}{s + \omega_o} \$

  2. Determine the "break frequency" a.k.a. cutoff frequency.
    This is straight forward for simple RC low pass filters & you have posted this \$ f_{cuttoff} = \frac{1}{2 \pi R C}\$.

  3. determine the frequency of interest.
    This is "two octave's above the cuttoff". Remembering 1 octave is x2.

  4. Determine the output ratio at this frequency.
    Using the transfer function from #1. and replacing s with \$j\omega \$ & then determining the magnitude

\$\endgroup\$
5
  • \$\begingroup\$ So does that mean that two octaves would be (Break Frequency x 3) or would it be (Break frequency + Break Frequency + Break Frequency)? \$\endgroup\$
    – John
    Commented Dec 3, 2017 at 20:50
  • \$\begingroup\$ it would be break x4. \$\endgroup\$
    – user16222
    Commented Dec 3, 2017 at 21:00
  • \$\begingroup\$ So, I've found that the interest frequency is about 23578.508 Khz and the break frequency is 5894.627 Khz. I can't find what the ratio is though, my book only tells me that the output voltage is 70.7% of the input. So would it just be 100/70.7? I really don't know what value I am looking for \$\endgroup\$
    – John
    Commented Dec 3, 2017 at 21:16
  • \$\begingroup\$ Think about the transfer function. If you replace "s" with "jw" \$\endgroup\$
    – user16222
    Commented Dec 3, 2017 at 21:44
  • \$\begingroup\$ ^^^ In other words, turn this circuit into the Laplace domain. This will help you with your bode plot. \$\endgroup\$
    – user103380
    Commented Dec 3, 2017 at 22:55

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.