Series RL current equation

Question

I am trying to derive a inductor current equation for \$i(t)\$ in a series RL circuit when the switch is closed at \$t=0\$, as seen below:

I have posted my attempt so far but I'm having trouble getting past a certain point which I have labelled equation (7) on my attached workings page.

I am unsure how to take the integral of the RHS of equation (7). I know the integral is the natural log, but I'm unsure of what happens inside the brackets.

I know what the answer should be and it can be seen circled at the bottom of my attempt page.

Answer 1

Everything up to step 7 is correct so starting from there: $${\int_0^t \frac{dt}{L\mathbin{/}R}=\int_0^i\frac{di}{V\mathbin{/}R - i}}$$ Multiplying both sides by \$-1\$ $${\int_0^t \frac{-dt}{L\mathbin{/}R}=\int_0^i\frac{di}{i - V\mathbin{/}R}}$$ You already have the integral of the LHS: $${\frac{-t}{L\mathbin{/}R}}$$ To solve the right hand side, we can use integration by substitution (Wikipedia): $${let\ \ u=i-V\mathbin{/}R}$$ $${thus \ du=di}$$ Pluging \$u\$ back into the RHS of equation 7 yields: $${\int_0^i \frac{du}{u}}=ln|u| \Big|_0^i$$ substituting \$u=i-V\mathbin{/}R\$ $${ln|i-V\mathbin{/}R|\Big|_0^i=ln|i-V\mathbin{/}R|-ln|0-V\mathbin{/}R|}$$ $${\rightarrow \ ln\Big|\frac{i-V\mathbin{/}R}{-V\mathbin{/}R}\Big|}$$ Equating both sides: $${\frac{-t}{L\mathbin{/}R}=ln\Big| \frac{i-V\mathbin{/}R}{-V\mathbin{/}R}\Big|}$$ Taking both sides as a power of \$e\$: $${e^{-t\frac{R}{L}}=-\frac{iR}{V}+1}$$ Solving for \$i\$ $${i=\frac{V}{R}\left(1-e^{-t\frac{R}{L}}\right)}$$

What if we didn't multiply by -1

So we start again after step 7: $${\int_0^t \frac{dt}{L\mathbin{/}R}=\int_0^i\frac{di}{V\mathbin{/}R - i}}$$ You already have the integral of the LHS: $${\frac{t}{L\mathbin{/}R}}$$ To solve the right hand side, we can use integration by substitution (Wikipedia): $${let\ \ u=V\mathbin{/}R - i}$$ $${thus \ du=-di}$$ Pluging \$u\$ back into the RHS of equation 7 yields: $${\int_0^i \frac{-du}{u}}=-ln|u| \Big|_0^i$$ substituting \$u=V\mathbin{/}R - i\$ $${-ln|V\mathbin{/}R - i|\Big|_0^i=-\left(ln|V\mathbin{/}R - i|-ln|V\mathbin{/}R - 0|\right)}$$ $${\rightarrow \ -ln\Big|\frac{V\mathbin{/}R - i}{V\mathbin{/}R}\Big|}$$ By law of logarithms: $${aln(x) = ln(x^a)}$$ Thus: $${-ln\Big|\frac{V\mathbin{/}R - i}{V\mathbin{/}R}\Big| = ln\Big|\frac{V\mathbin{/}R}{V\mathbin{/}R - i}\Big|}$$ Equating both sides: $${\frac{t}{L\mathbin{/}R}=ln\Big|\frac{V\mathbin{/}R}{V\mathbin{/}R - i}\Big|}$$ Taking both sides as a power of \$e\$: $${e^{t\frac{R}{L}}=\frac{V\mathbin{/}R}{V\mathbin{/}R - i}}$$ Solving for \$i\$ $${\frac{V\mathbin{/}R - i}{V\mathbin{/}R} = \frac{1}{e^{t\frac{R}{L}}}}$$ $${1 - i\frac{R}{V} = e^{-t\frac{R}{L}}}$$ Rearranging: $${i=\frac{V}{R}\left(1-e^{-t\frac{R}{L}}\right)}$$

Answer 2

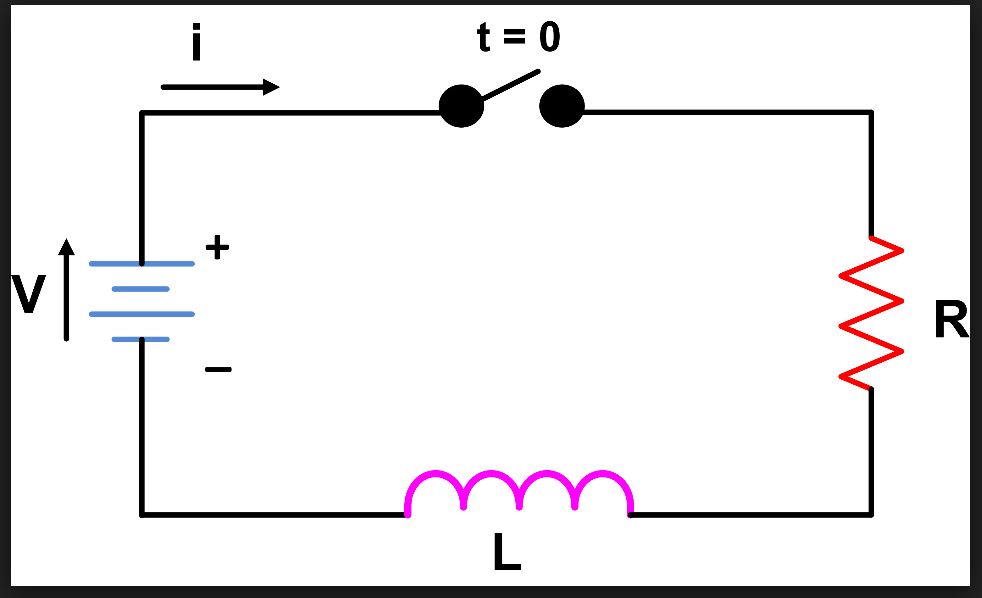
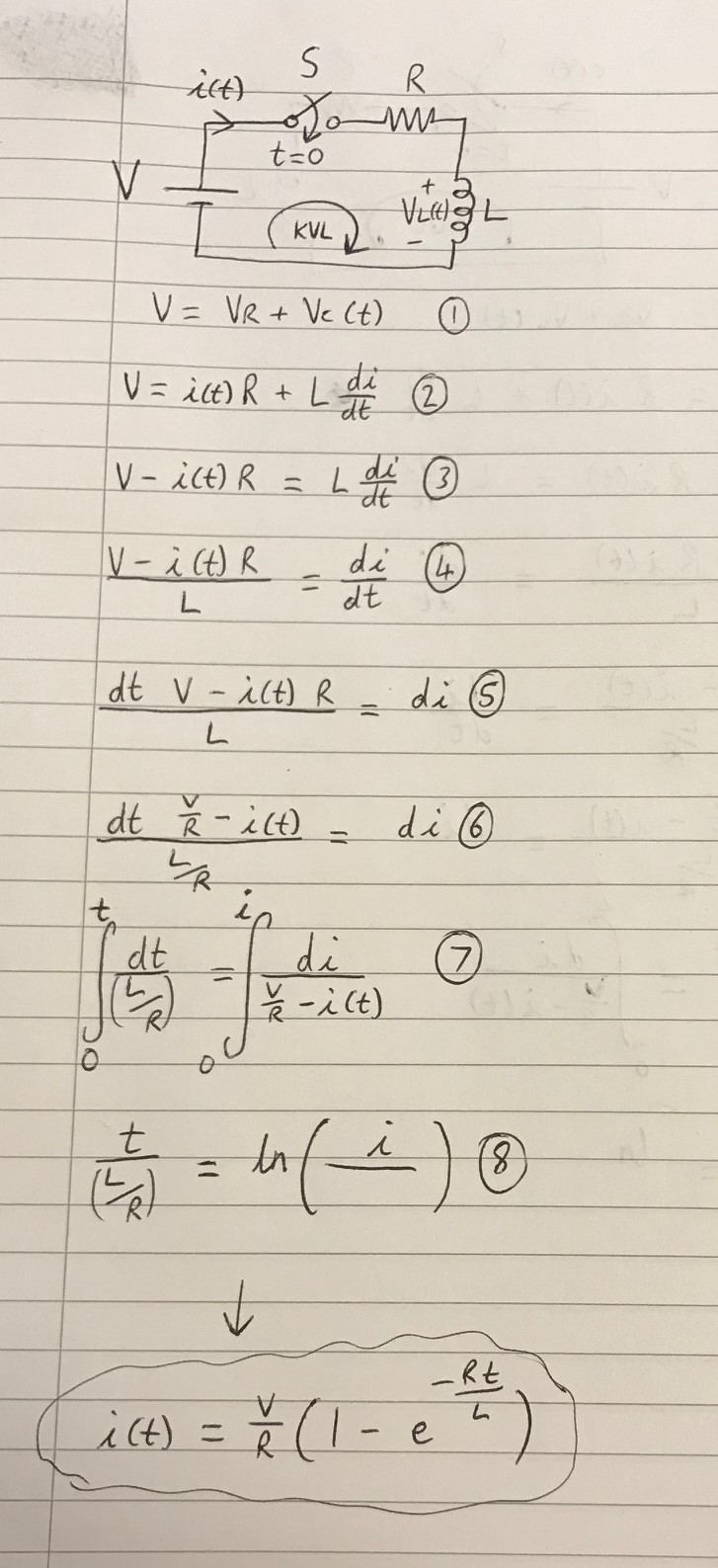To solve in the time domain, first re-arrange equation 2 in the form

\$ \frac{di}{dt} + \frac{R}{L}\,i= \frac{V}{L} \$

This is a linear first order differential equation. This class of equations can be solved by multiplying through by an integrating factor. In this case the integrating factor is

\$ e^{\small\frac{^R}{L}\normalsize t} \$

Multiplying through we get

\$ e^{\small\frac{^R}{L}\normalsize t}\, \frac{di}{dt} + \frac{R}{L} \, e^{\small\frac{^R}{L}\normalsize t} \,i = \frac{V}{L} \, e^{\small\frac{^R}{L}\normalsize t} \$

By the product rule, the left side becomes

\$ \frac{d}{dt} \left( e^{\small\frac{^R}{L}\normalsize t}\,i \right) = \frac{V}{L} \, e^{\small\frac{^R}{L}\normalsize t} \$

Integrate both sides with respect to t

\$ \int\frac{d}{dt}\left(e^{\small\frac{^R}{L}\normalsize t}\,i \right)\,dt = \frac{V}{L}\int e^{\small\frac{^R}{L}\normalsize t} \, dt \$

and we get

\$ e^{\small\frac{^R}{L}\normalsize t}\,i = \frac{V}{L}\,\frac{L}{R} \,e^{\small\frac{^R}{L}\normalsize t} + c\$, where c is a constant. Dividing through by \$ \,e^{\small\frac{^R}{L}\normalsize t} \$ we get

\$ i = \frac{V}{R} \, + ce^{-\small\frac{^R}{L}\normalsize t}\$

Solve for \$c\$ by setting initial conditions \$t=0\$ and \$\, i _{(t=0)} = 0\$ to obtain

\$c = - \frac{V}{R}\$

Substitute for c to obtain the solution

\$ i = \frac{V}{R} \,\left(1 - e^{-\small\frac{^R}{L} \normalsize t} \right)\$