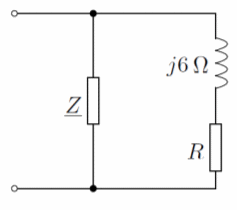
Here are two ways of solving it. I know it's not the best to give you the answer straight away, but I'm very rusty anyways so I wanted to go through with it from start to end. Plus, you've shown your attempt, and that means the world to me and that you should be able to absorb my answer.
Just looking at the apparent power, power factor and voltage.
\$
\begin{align}
U &= |(3+6i)|\sqrt{\frac{666}{3}} &\approx 99.95\text{ V}
\\P &= 3370\times0.937 &\approx 3157.7 \text{ W}
\\Q &= 3370\sqrt{1-0.937^2} &\approx 1177.3 \text{ VAr}
\\Q &= \text{Due to being capacitive} & -1177.3 \text{ VAr}
\end{align}
\$
With these values we can set up another equation that we know
$$
\begin{align}
P+Q&=\frac{U^2}{Z||(3+6i)}
\\\\\frac{U^2}{P+Q}&=Z||(3+6i)
\\\\\frac{U^2}{P+Q}&=\frac{Z(3+6i)}{Z+3+6i}
\\\\(Z+3+6i)\frac{U^2}{P+Q}&=Z(3+6i)
\\\\(3+6i)\frac{U^2}{P+Q}&=Z(3+6i-\frac{U^2}{P+Q^*})
\\\\Z = \frac{(3+6i)\frac{U^2}{P+Q^*}}{(3+6i-\frac{U^2}{P+Q^*})}&= \frac{(3+6i)\frac{99.95^2}{3157.7+1177.3i}}{(3+6i-\frac{99.95^2}{3157.7+1177.3i})} \approx 2-2i \text{ ohm}
\end{align}
$$
I am a little bit unsure about where the conjugate of Q comes in. I know that it does, just not 100% sure when. If I did this at an exam I would try to get rid of a complex denominator because it complicates things.
Or you can do it like this, closer to what you tried.
\$
\begin{align}
I_{RL} &= \sqrt{\frac{666}{3}} &\approx 14.9\text{ A}
\\U_{RL} & = |3+6i|I_{RL} &\approx 99.95\text{ V}
\\P &= 3370\times0.937 &\approx 3157.7 \text{ W}
\\Q &= 3370\sqrt{1-0.937^2} &\approx 1177.3 \text{ VAr}
\\Q &= \text{Due to being capacitive} & -1177.3 \text{ VAr}
\end{align}
\$
And then we plug them in into similar ways that you were doing
$$
\begin{align}
P_Z&=P-3I_{RL}^2=3157.7-666= 2491.7 \text{ W}
\\\\Q_Z&=Q-i6I_{RL}^2=-1177.3i-1332i= -2509.3 \text{ VAr}
\\\\I_Z&=\frac{|P_Z+Q_Z|}{U_{RL}} = 35.4 \text{ A}
\\\\Z &= \frac{P_Z+Q_Z}{I_Z^2} = \frac{2491.7-2509.3i}{35.4^2} \approx 2-2i \text{ ohm}
\end{align}
$$
There are more ways, but I don't want to spend too much time on this answer. I hope this gave you some insight into how to deal with these complex circuits.