I have to find yn/xn ratio.
From the circuit analysis i found out transfer function Uout//Uin =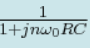 . I am having hard time trying to isolate two coefficients of two complex fourier Series. Any help is highly appreciated. Thank you.
. I am having hard time trying to isolate two coefficients of two complex fourier Series. Any help is highly appreciated. Thank you.
For LTI systems, use the linearity property. $$ \begin{align} H(s) \cdot \left(\sum X_i (s)\right) \mapsto{} & \sum H(s)\cdot X_i (s) & {}={} & \sum Y_i(s) \\ h(t) \circledast \cdot \left(\sum x_i (t)\right) \mapsto{} & \sum h(t)\circledast x_i (t) & {}={} & \sum y_i(t) \end{align} $$
From the above, \$\frac{y_i}{x_i}\$ or \$\frac{Y_i}{X_i}\$ can be found by comparing like terms.
U_inis the sum of certain signals. Can you take it from here? \$\endgroup\$