alternating current in a RC circuit

Question

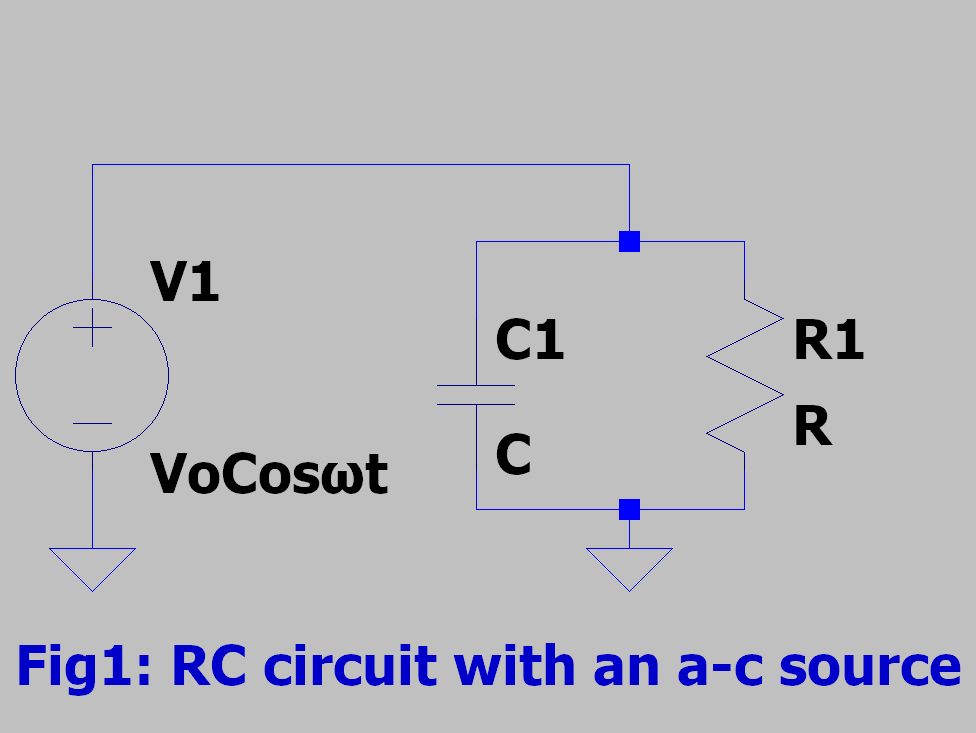
This is a problem from a book.

Consider a parallel arrangement of a capacitance C and a resistor R. An external voltage \$V(t) = V_0coswt\$ is applied to this arrangement. Show that the total current i(t) is given by:

$$i(t) = \frac{V_o}{R}cos(ωt) - CωV_osin(ωt) $$

Solution:

Since C and R are in parallel, their collective admittance is \$Y = Y_C + Y_R\$

\$Y_C = jωC\$

\$Y_R = \frac{1}{R} \$

\$Y = jωC +\frac{1}{R} = \frac {1+jωCR}{R} \$

Current \$ i(t) = VY \$

After solving, I got the current as :

\$i(t) = \frac{V_o}{R}cos(ωt) +jCωV_ocos(ωt) \$

What was the error in the step by step solution? How is jcosωt = -sinωt?

Reference: Electrical engineering materials, A.J. Dekker, page 77, PHI/Pearson.

Answer 1

What was the error in the step by step solution?

There was no error.

How is jcosωt = -sinωt

They are the same when recognizing Euler identities for sin and cos.

Further reading on Euler identities and formulas from Newcastle University.

Note that the link uses "i" as the complex operator and not "j".