I have this simple circuit. And from what I calculated the output impedance seen from the OUT node comes to about \$138.765 \Omega\$.
$$ X_c = \frac{1}{2\pi * 890*10^3 * 10^{-9}} = 178.8257\Omega $$
$$ Z_{out} = R2||X_c = \frac{220 * 178.8257}{\sqrt{220^2 + 178.8257^2}} = 138.765\Omega$$
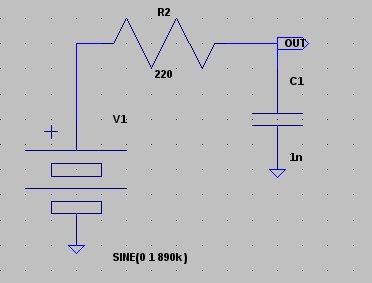
With an input of 890K Hz sine wave of 2v pk-pk, the voltage at OUT node comes to is about 1.2615v pk-pk.
If I connect a resister of the same value of \$138.765 \Omega\$, the voltage at OUT node should be half ed.
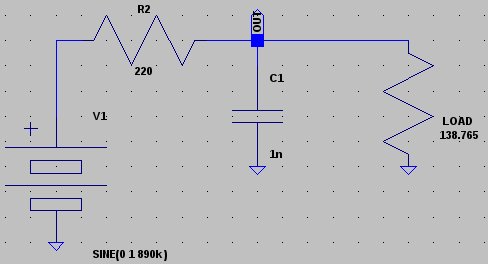
But I see a voltage of 697.843mV instead of 630mV. What is the problem, am I calculating the output impedance wrongly?
