How do KCL and KVL equations look like in a circuit with a switch and two voltages sources?

Question

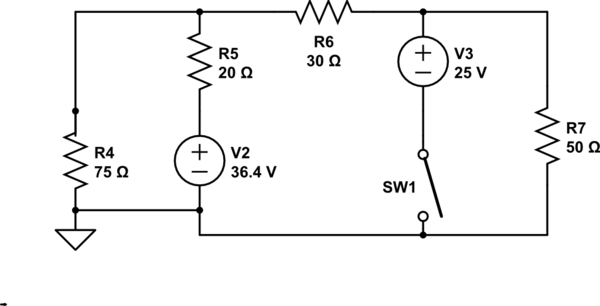
Let I1 be the current through R6, V3, and R7 Let I2 be the current through R5, and V2 Let I3 be the current through R4

Suppose I did not know V2 = 36.4V, but I know the voltage across R7 is 15V.

How do I figure out V2 ?

Here is my KCL =

I2 = I1 + I3

KVL

30I1+25 + 50I1-V2 + 20I2 = 0

-20I2 + V2 -75I3 = 0

When I solve the above 3 equations I get V2 = 25, not 36.4. What am I doing wrong?

^{simulate this circuit – Schematic created using CircuitLab}

Answer 1

Your equations are wrong. The \$V_3\$ branch is not considered since the switch is open. If the switch was open, you would then have 3 loops in total, and \$I_1\$ would be split.

Following your assumptions about the currents:

$$I_2 = I_1 + I_3$$

\$I_1\$ can be gotten right away since you somehow know that the voltage across \$R_7\$ (top to bottom) is \$15 V \$.

$$I_1 = 15/50 = 0.3 A$$ $$I_2 = 0.3 + I_3 ............(1)$$

The equation for the first loop is:

$$-20I_2 - V_2 - 75I_3 = 0$$ $$20I_2 + V_2 = -75I_3..........(2)$$

The equation for the second loop is:

$$80I_1 + V_2 + 20I_2 = 0$$ $$24 + V_2 + 20I_2 = 0$$ $$20I_2 + V_2 = -24............(3)$$

Equating (2) and (3) gives:

$$-24 = -75I_3$$ $$I_3 = 0.32 A$$

From (1):

$$I_2 = 0.3 + 0.32 = 0.62 A$$

From (3):

$$V_2 = -24 - 20I_2 = -24 - 12.4 = -36.4 V$$

Meaning, the polarity of \$V_2\$ in your schematic is the other way round.