Currently I am learning control systems. I specifically find the mathematics very hard to understand.
For my exam I am practicing on this question: "Determine the transfer function of this circuit in the S-domain."
I came to here:
I don't know if it's correct and I don't know how to simplify this. Could you please explain me in steps how to solve this mathematically?
Thank you in advance for your help!
Edit: In order to address your question more completely, the step you have missed in completing the transfer function is to substitute in the circuit element impedances for the components in the circuit. Also, you you did calculate the parallel impedance of \$R_2\$ and \$C\$ incorrectly.
In order to find the transfer function \$H(s)\$ of this circuit we use the voltage divider rule, that is: \begin{equation} H(s) = \frac{U_{out}}{U_{in}}(s) = \frac{Z_2}{Z_1 + Z_2} \end{equation} where, \begin{equation} Z_2 = R_2||\frac{1}{sC} \qquad Z_1 = R_1 \end{equation} so we now have, \begin{equation} Z_2 = \left(\frac{1}{R_2} + sC\right)^{-1} = \left(\frac{sCR_2 + 1}{R_2}\right)^{-1} = \frac{R_2}{sCR_2 + 1}. \end{equation}
From here we can derive the transfer function using the previously stated voltage divider rule, substituting in the impedances for \$Z_1\$ and \$Z_2\$, \begin{equation} \begin{split} H(s) &= \frac{\frac{R_2}{sCR_2 + 1}}{\frac{R_2}{sCR_2 + 1} + R_1} = \frac{R_2}{R_2 + R_1 + sCR_1R_2} \\ &=\frac{\frac{1}{R_1C}}{s + \frac{R_1 + R_2}{R_1R_2C}}= \frac{\frac{1}{R_1C}}{s + \frac{1}{R_1||R_2C}}. \end{split} \end{equation} Note how the denominator of the transfer function is normalized to the coefficient of the largest power of \$s\$. From this transfer function if we look at the limiting behavior as \$s \rightarrow 0\$ or \$s \rightarrow \infty\$ we see that the circuit exhibits a "low-pass" behavior.
Since often in controls the impulse response \$H(s)\$ is not what you will be interested in, to find the time domain response to say a step (Heaviside) function you would multiply the impulse response function by the Laplace transform of the time domain voltage input, \begin{equation} U_{out}(s) = H(s)U_{in}(s), \end{equation} and taking the inverse Laplace transform of the result you arrive at the time domain response of your circuit to the given input. \begin{equation} U_{out}(t) = \scr{L}^{-1}\lbrace H(s) U_{in}(s) \rbrace \end{equation}
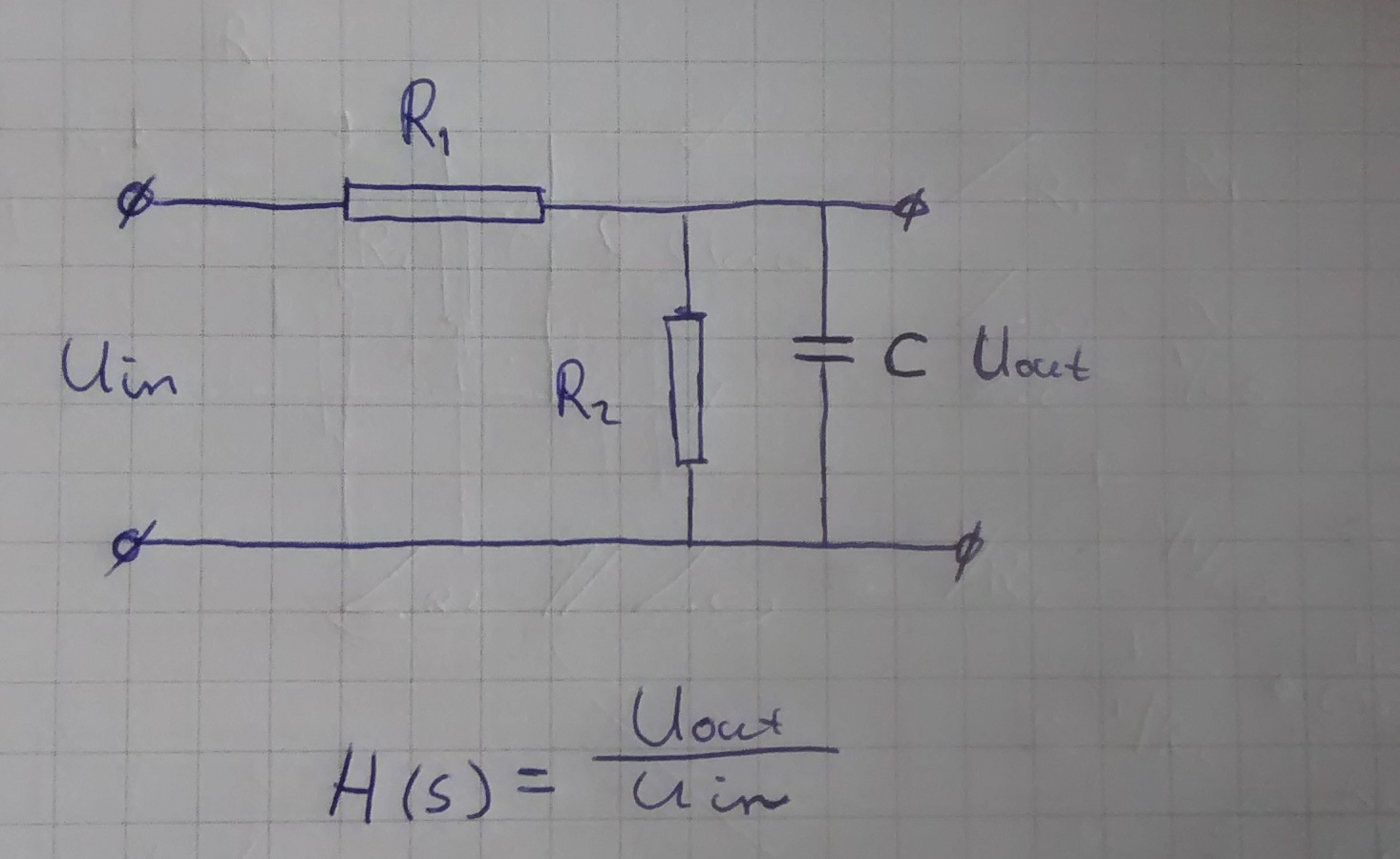
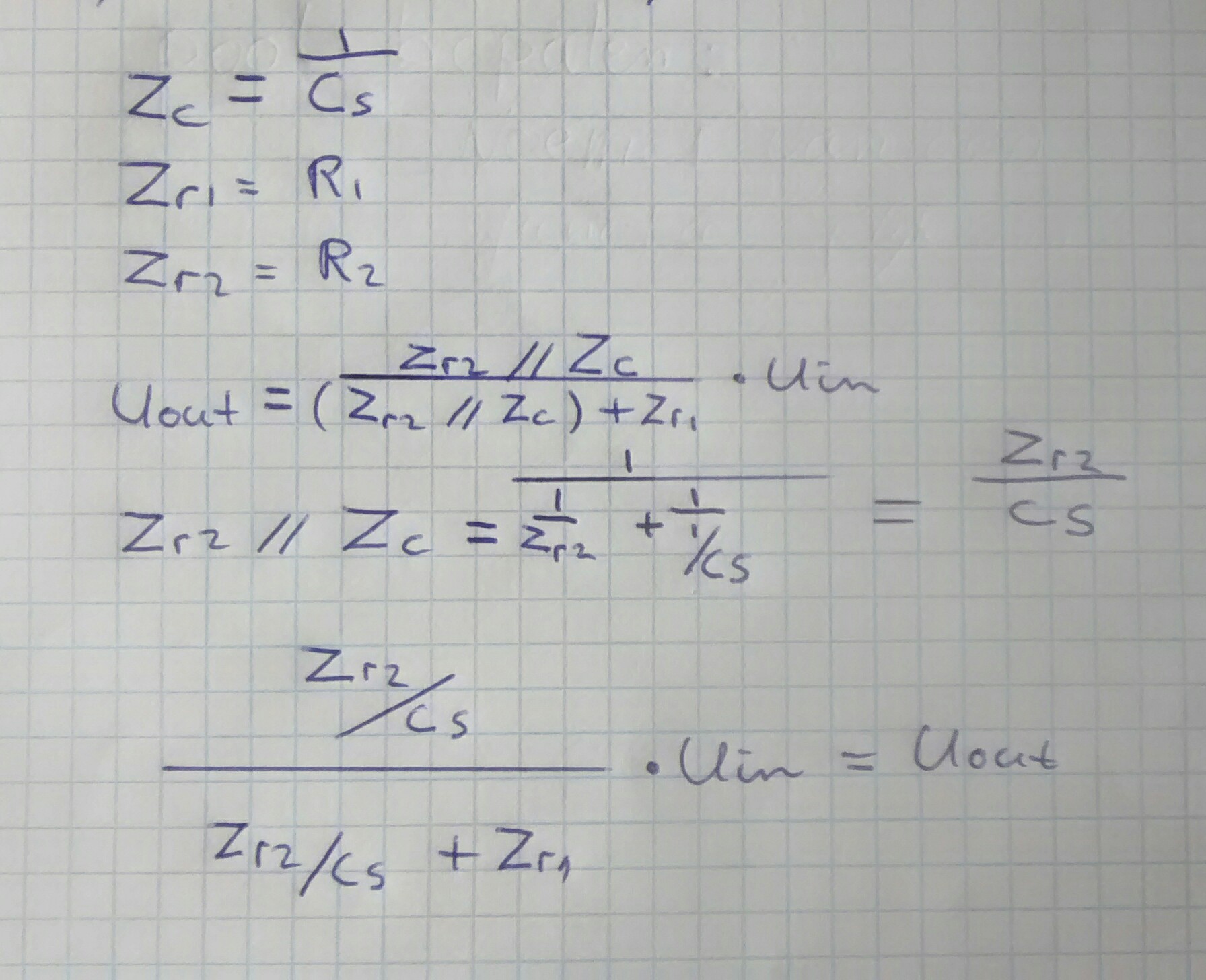A slightly different approach here and there is nothing I can see wrong with the other answer is to first find the impedance of \$ C \$ in parallel with \$ R_2 \$ from "product over sum" formula for two impedances in parallel.
$$Z_{bot} =\dfrac{R_2 \cdot \dfrac{1}{s \cdot C}}{R_2 + \dfrac{1}{s \cdot C}} = \dfrac{R_2}{1+ s \cdot C \cdot R_2}$$
Now we note that the circuit is a potential divider so
$$H(s) = \dfrac{U_{out}}{U_{in}} = \dfrac{Z{bot}}{Z{top} + Z{bot}}$$
Where \$ Z_{top} \$ is just \$ R_1 \$ and \$ Z_{bot} \$ is the parallel impedance we just calculated.
$$H(s) = \dfrac{\dfrac{R_2}{1+ s \cdot C \cdot R_2}}{R_1 + \dfrac{R_2}{1+ s \cdot C \cdot R_2}} = \dfrac{R_2}{R_1 + R_2} \cdot \dfrac{1}{1 + s \cdot C \cdot \dfrac{R_1 \cdot R_2}{R1+R2}}$$
Writing the transfer function is this way we can easily see by inspection that we have a gain at low frequencies of
$$\dfrac{R_2}{R_1 + R_2}$$
and a single pole making this a low pass filter. The pole is located where
$$\left| s \cdot C \cdot \dfrac{R_1 \cdot R_2}{R1+R2} \right| = 1$$
