I'm really confused in the following question,
The flux linkage (\$\lambda\$) and current (\$i\$) relation for an electromagnetic system is \$ \lambda = (\sqrt i / g\$ ) . When \$i = 2 A\$ and \$g\$ (air-gap length) = 10 cm, the magnitude of mechanical force on the moving part, in \$N\$, is?
My approach, $$f = i \frac{d \lambda}{d x}$$ $$ |f| = \frac{i \sqrt i}{g^2} = 282.84 $$ But the answer is in the range 186-190 N
The following curve shows the magnetic-field energy and co-energy.
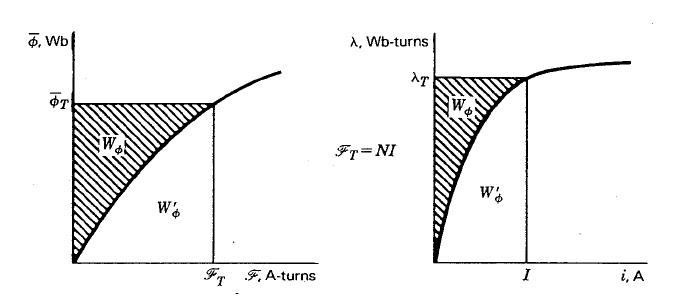On moving a small distance \$ dx \$, the current remains constant but the flux increases by an amount \$ d \lambda\$
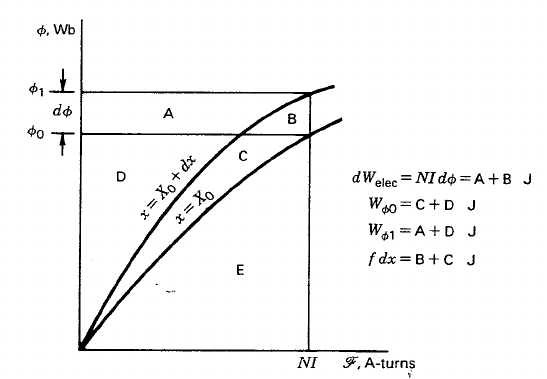
The curve between \$ \lambda \$ and \$ i \$ follows the same pattern. Since, the current remains constant, the differential energy \$ d W_\text{elec} \$ taken from the system is half stored in magnetic field as \$ d W_{\phi} \$ and the rest is used in doing virtual work \$ f\; dx\$, so on balancing the energy we get $$ 2\; d W_\phi = d W_{\phi} + f\; dx $$ $$ f = \frac{d W_{\phi}}{dx} \quad \text N $$ Since the question does not specify about the direction of motion,i.e., \$ x\$ let us assume that the force decreases as \$ x \$ increases and displacement \$ g \$ decreases as \$ x \$ increases. So, the force can be calculated as $$ f = - \frac{d W_\phi}{dx} = \frac{d W_\phi^{'}}{dx} $$ As, co-energy \$ W_\phi^{'} \$ increases when \$ d W_\phi \$ decreases. $$ W_\phi^{'} = \int_0^2 \lambda \; di = \int_0^2 \frac{\sqrt{i}}{g}\; di $$ $$ f = \frac{d W_\phi^{'}}{dx} = -\frac{d W_\phi^{'}}{dg} = -\frac{d}{dg} \int_0^2 \frac{\sqrt{i}}{g}\; di = -\int_0^2 \frac{\partial}{\partial g} \left( \frac{\sqrt{i}}{g} \right)\; di$$ $$ f = \int_0^2 \frac{\sqrt{i}}{g^2}\; di = \frac{2}{3} \left[ \frac{i^{3/2}}{g^2} \right]_0^2 = 188.56 \; N$$