So I need help with the following exercise on op-amps (picture). I know how basic op-amps work but here I dont know how they got to the formula (circled in red). Do you need to use KVL or KCL ? I know that the gain on a normale inverting op-amp is V0=-Zf/Zi*Vi.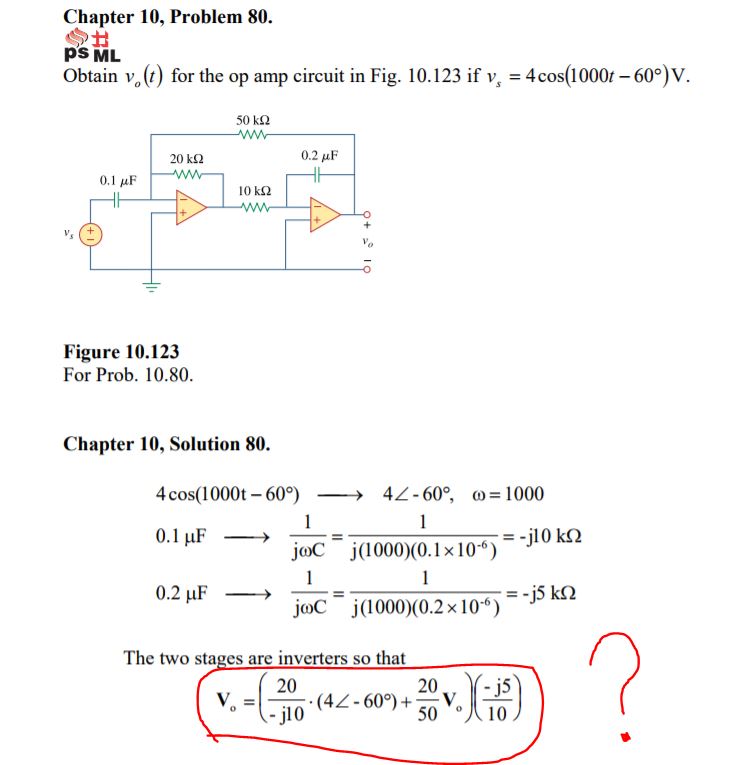
Ok so now I applied KCL at the two nodes and this is what I got (picture 2). But after rewriting it and replacing Vs by its value, it still isn't the same equation as the answer given, what did I do wrong ?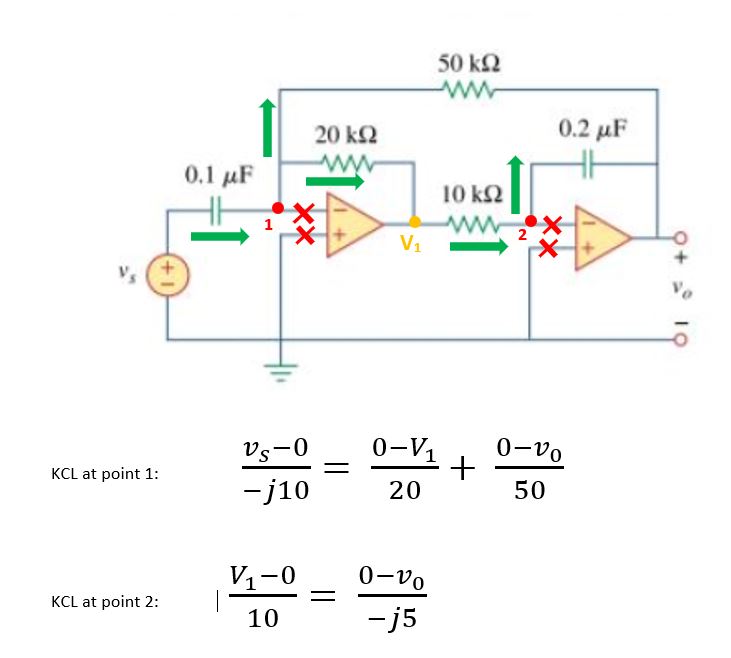
\$\begingroup\$
\$\endgroup\$
1
-
3\$\begingroup\$ The question is flawed with positive feedback and an output stage that will quickly integrate and Vo(t) will saturate the output to the supply rail because of this. Complain to Prof , that it is unrealistic. \$\endgroup\$– D.A.S.Commented Jan 5, 2018 at 20:09
Add a comment
|
2 Answers
\$\begingroup\$
\$\endgroup\$
As Tony Stewart commented, the circuit as shown will not work. Vo will be saturated, and V1 will be 0.4 the level of V2, but with the opposite polarity. Two inverters in a row produce positive feedback, not negative.
As a starting point, let's say Vs is set at zero, and Vo is 1 volt. Then V1 will be be -.4, and Vo will integrate even higher. The process only stops when Vo saturates. This is classic positive feedback, and there will be no reliable response to an AC Vs.
In order to get the circuit to work, the + and - inputs of one of the op amps (preferably the second) must be swapped.
answered Jan 6, 2018 at 2:30
\$\begingroup\$
\$\endgroup\$
The op amps are ideal, and they have negative feedback ,so it is true, that the voltages of the inverting, and non-inverting inputs are the same. In this case, both inverting inputs are virtual ground (has zero potential).
Now you can write two KCL equation at these nodes (assuming no current flowing in the amplifier beacause an ideal amplifier has infinite resistence at the inputs). From these equations you should get the transfer function, and the answer.
