How to find peak to peak and rms current for square wave AC circuit?

Question

I have a circuit with AC square wave at 10V and 4 Hz. There is only a single resistor of 1000 ohm in the circuit. I would like to calculate the rms current and also the peak to peak current of this circuit.

Answer 1

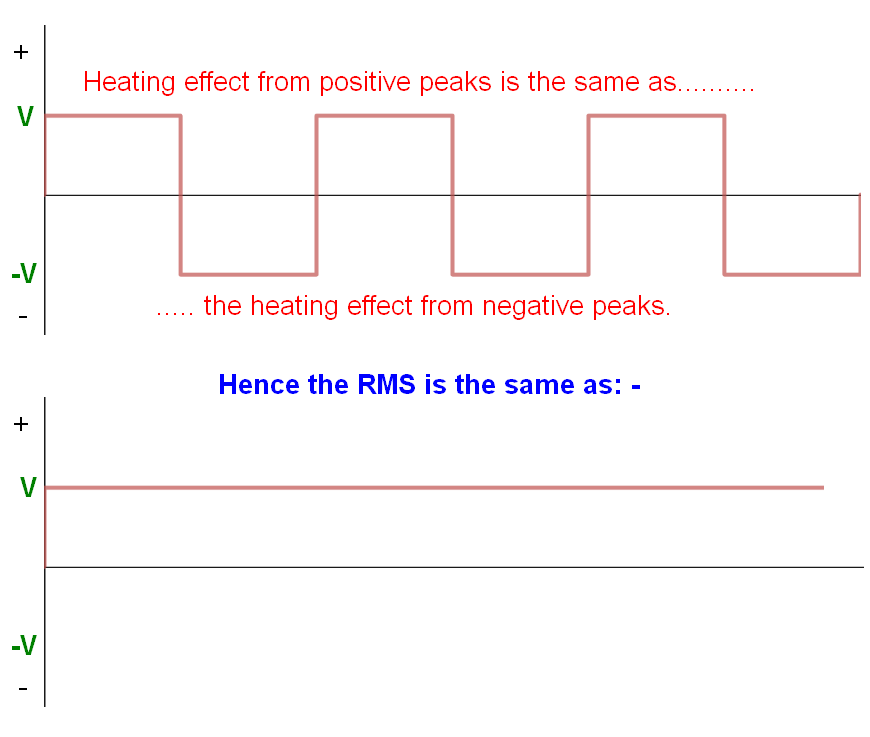
I have a circuit with AC square wave at 10V and 4 Hz. There is only a single resistor of 1000 ohm in the circuit. I would like to calculate the rms current and also the peak to peak current of this circuit.

• So calculate RMS current based on the peak voltage of 10 volts and 1000 ohms.
• The peak current is also the same as the RMS current
• The peak to peak current is twice the peak current.

Answer 2

The peak can be found by using:

$$\text{V}_\text{R}=\text{V}_\text{in}=\text{I}_\text{R}\cdot\text{R}=\text{I}_\text{in}\cdot\text{R}\tag1$$

And the RMS can be found by finding:

$$\frac{1}{100}=\overline{\text{I}}_\text{R}=\overline{\text{I}}_\text{in}=\sqrt{\frac{1}{\frac{1}{4}-0}\int_0^\frac{1}{4}\text{I}_\text{R}^2\space\text{d}x}=\sqrt{\frac{1}{\frac{1}{4}-0}\int_0^\frac{1}{4}\text{I}_\text{in}^2\space\text{d}x}=$$ $$\sqrt{\frac{1}{\frac{1}{4}-0}\int_0^\frac{1}{4}\left(\frac{\text{V}_\text{R}}{\text{R}}\right)^2\space\text{d}x}=\sqrt{\frac{1}{\frac{1}{4}-0}\int_0^\frac{1}{4}\left(\frac{\text{V}_\text{in}}{\text{R}}\right)^2\space\text{d}x}\tag2$$