"The effective value of an alternating current is that current which will produce the same heating effect as an equivalent direct current. The effective value is called root mean square RMS value."
What is that "heating effect" stated in the sentence?
The heating effect refers to the power that would be dissipated if that current flowed in a pure resistor. Such a resistor would convert all of its dissipated power into heat. The RMS value of a current is equal to a DC current that produces the same amount of heat in that resistor.
Generally, currents we want to know the effect of the current. For example, how much power or heating does it provide. The standard method for doing this is to calculate or measure the true RMS (root mean square) of the current waveform. If you like, the resultant value gives you the equivalent DC current that would have the same effect as stated in your definition.
simulate this circuit – Schematic created using CircuitLab
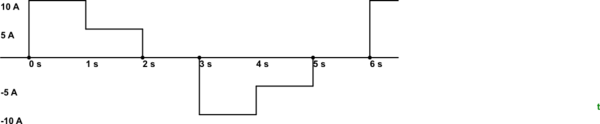
Figure 1. A strange AC waveform.
A simple example may help. In Figure 1 we have an AC waveform whose geometric average or integral will be zero. Clearly the power delivered is non-zero so let's calculate the effective current.
That's the squared part of RMS done. Now get the mean.
Now get the root.
For the negative half-cycle the result will be the same due to the squaring.
Note that if we just calculated the "average" current for one half-cycle (the the positive one, for example) we would have got \$ I_{AVG} = \frac {10 + 5 + 0}{3} = 5 \; A \$. The RMS value is much higher because the \$ 10^2 \$ term has a large effect.
This waveform with a 10 A peak current would heat a resistor by the same amount a 6.5 A steady DC current would.
For a sinewave the RMS value is \$ \frac {1}{\sqrt 2} V_{peak}\$.
In a DC circuit, if the supply voltage is 10 volts and it was placed across a 5 ohm resistor it would generate 20 watts (\$V^2/R\$). That is the "heating effect".
A sinewave that produces the same power (and "heating effect") in the same resistor has an RMS value also of 10 volts. The peak of that sine wave will be \$\sqrt2\$ higher at 14.14 volts.
You have two different systems.
In the Direct Current system, current and voltage are constant with reference to (wrt) time.
In the Alternating Current system, current and voltage are NOT constant wrt time. \$ v = V_M \ sin \ (\omega t)\ V\$
To do calculations or comparisons, we need an effective value for ac which is equivalent to dc.
Average of a sinewave is 0. Can't use that.
\$V_M\$ only occurs twice per cycle. Ditto.
So boil water. Vary the ac voltage until it boils the same amount of water using the same resistor in the same time. The power must be the same.
Then work the math. $$ \begin{align} P_{DC} & = P_{{AVG}_{ac}} \\P_{DC} & = \frac {P_M} {2} \\ I^2 R & = \frac {I_M^2 \ R} {2} \\ I^2 & = \frac {I_M^2} {2} \\ I & = \frac {I_M} {\sqrt{2}} \\ I & = 0.707 I_M \end{align} $$
The RMS value of an AC sinewave (current or voltage) is the equivalent or effective value which produces the same heat as a DC battery. If they do the same work, they must be equivalent.
