How do multiple mixing stages work in a spectrum analyzer?

Question

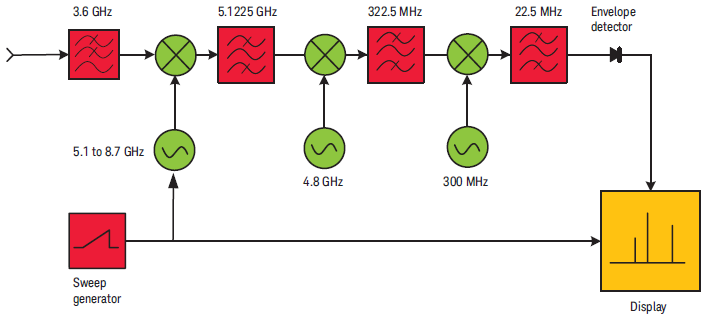
I'm reading about spectrum analyzers from Keysight's AN 150. On page 12 they explain how multiple mixing stages are used to obtain narrower IF filters. The block diagram offered is the following one:

They state that the tuning equation would then be:

$$f_{sig}=f_{LO1}-(f_{LO2}+f_{LO3}+f_{final \ IF})$$

And that

$$f_{LO2}+f_{LO3}+f_{final \ IF} = f_{first \ IF}$$

So they say:

Simplifying the tuning equation by using just the first IF leads us to the same answers.

My doubts

I tried to deduce the first equation, but I'm getting a sign wrong. My reasoning was the following. I have a sinusoid of frequency \$f_{sig}\$. After the first mixing stage, I get two components at \$f_{LO1} + f_{sig}\$ and \$f_{LO1} - f_{sig}\$. The first one will be filtered out, so after the second mixing stage we have \$f_{LO2} - f_{LO1} + f_{sig}\$ and \$f_{LO2} + f_{LO1} - f_{sig}\$. Again, the first one is filtered out and after the third and last mixing stage, and after the filtering too, we have that the following equality must be satisfied to generate a response on the display: $$f_{LO3}-f_{LO2} + f_{LO1} - f_{sig}=f_{final \ IF}$$ Reordering: $$f_{sig}=f_{LO1} -(f_{LO2}-f_{LO3}+ f_{final \ IF})$$ So in my equation, \$f_{LO3}\$ appears with a negative sign, but on the AN it doesn't. Where did I make the mistake?

The other question I have is regarding the second equation shown above: \$f_{LO2}+f_{LO3}+f_{final \ IF} = f_{first \ IF}\$. Is this a design requirement? I mean, must this equality be satisfied in order for the spectrum analyzer to work properly? Could the frequencies of each mixing stage be any combination so that the equality holds?

Answer 1

A true multiplicative mixer produces components at both the sum and difference of the frequencies (real mixers will usually produce components at other frequencies too but we can ignore that for now)). Generally filters will be arranged so that only one of those components passes through to the next stage.

Frequencies (in this context) are always positive, so difference here means absoloute value of the difference.

In this case the filters are not shown, but from the frequencies labeled on the diagram they are clearly always taking the difference and filtering out the sum.

So writing this out as equations.

$$f_\mathrm{if1} = |f_\mathrm{sig}-f_\mathrm{lo1}|$$

$$f_\mathrm{if2} = |f_\mathrm{if1}-f_\mathrm{lo2}|$$

$$f_\mathrm{if3} = |f_\mathrm{if2}-f_\mathrm{lo3}|$$

If we combined these equations together and tried to solve them we would end up with 8 possible soloutions for \$f_\mathrm{sig}\$ in terms of \$f_\mathrm{if3}\$. Which of those 8 soloutions is correct? well we need to go back to our diagram.

$$f_\mathrm{sig} < f_\mathrm{lo1} \rightarrow f_\mathrm{if1} = f_\mathrm{lo1} - f_\mathrm{sig}$$

$$f_\mathrm{if1} > f_\mathrm{lo2} \rightarrow f_\mathrm{if2} = f_\mathrm{if1} - f_\mathrm{lo2}$$

$$f_\mathrm{if2} > F_\mathrm{lo3} \rightarrow f_\mathrm{if3} = f_\mathrm{if2} - f_\mathrm{lo3}$$

Combing these equations.

$$ f_\mathrm{if3} = ((f_\mathrm{lo1} - f_\mathrm{sig}) - f_\mathrm{lo2})- f_\mathrm{lo3} $$

Getting rid of the brackets.

$$ f_\mathrm{if3} = f_\mathrm{lo1} - f_\mathrm{sig} - f_\mathrm{lo2}- f_\mathrm{lo3} $$

Rearrange to make \$f_\mathrm{sig}\$ the subject.

$$ f_\mathrm{sig} = f_\mathrm{lo1} - f_\mathrm{lo2}- f_\mathrm{lo3} - f_\mathrm{if3}$$

Which is equvilent to

$$ f_\mathrm{sig} = f_\mathrm{lo1} - (f_\mathrm{lo2} + f_\mathrm{lo3} + f_\mathrm{if3})$$

P.S curiously the diagram labels the first Lo as 5.1 to 8.7 GHz but to get the frequencies shown in the rest of the diagram would require a first LO of 8.7225 GHz.

Answer 2

Looks like you didn't properly select the mixer outputs. You also have to make sure the frequency is always positive. The output of the mixer is both the sum and the difference, but the difference is really the absolute value of the difference or the higher frequency minus the lower frequency. Here's how it works:

At the input, you have \$f_{sig}\$. This is low pass filtered at 3.6 GHz and mixed with \$f_{LO1}\$. The result is \$f_{LO1} + f_{sig}\$ and \$f_{LO1} - f_{sig}\$. This is bandpass filtered at 5.1225 GHz. Since \$f_{LO1}\$ ranges from 5.1 to 8.7 GHz, the output of the filter must correspond to \$f_{LO1} - f_{sig}\$.

At the second mixer, \$f_{LO1} - f_{sig}\$ is mixed with a fixed \$f_{LO2}\$ of 4.8 GHz. The result is \$f_{LO1} - f_{sig} + f_{LO2}\$ and \$f_{LO1} - f_{sig} - f_{LO2}\$. This is bandpass filtered at 322.5 MHz, so only \$f_{LO1} - f_{sig} - f_{LO2}\$ passes through the filter.

At the third mixer, \$f_{LO1} - f_{sig} - f_{LO2}\$ is mixed with a fixed \$f_{LO3}\$ of 300 MHz. The result is \$f_{LO1} - f_{sig} - f_{LO2} + f_{LO3}\$ and \$f_{LO1} - f_{sig} - f_{LO2} - f_{LO3}\$. This is bandpass filtered at 22.5 MHz, so only \$f_{LO1} - f_{sig} - f_{LO2} - f_{LO3}\$ passes through the filter. So \$f_{finalIF} = f_{LO1} - f_{sig} - f_{LO2} - f_{LO3}\$.

Reordering that results in \$f_{sig} = f_{LO1} - (f_{LO2} + f_{LO3} + f_{finalIF})\$, which matches the documentation.

As for your second question, the first IF is just the signal after the first mixer. \$f_{firstIF} = f_{LO1} - f_{sig}\$. If you substitute that, you get \$f_{finalIF} = f_{firstIF} - f_{LO2} - f_{LO3}\$, which rearranges to \$f_{firstIF} = f_{finalIF} + f_{LO2} + f_{LO3}\$, which also matches the documentation.