You can estimate the internal resistance (hence the shourt-circuit current), without using an ammeter. All you need is a single external resistor and a voltmeter.
First, a battery can be modeled as a series of an ideal voltage source of \$V_{BATT}\$ volts and a resistor of \$R_{BATT}\$ ohms. \$R_{BATT}\$ is the battery's internal resistance. This model is not perfect, because \$R_{BATT}\$ actually varies with charge, load, temperature, etc. But it's close enough, so a typical rechargeable AA / AAA battery may look like this:
\$V_{BATT}\$ is easily measured with a voltmeter - it's the open-circuit voltage of the battery. Now, to calculate \$R_{BATT}\$, we need that extra resistor of \$R_{LOAD}\$ ohms:
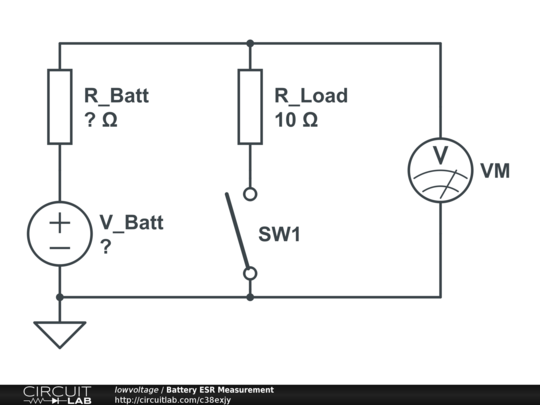
When the \$R_{LOAD}\$ resistor closes the circuit, it forms a voltage divider with \$R_{BATT}\$. If the voltage across \$R_{LOAD}\$ is \$V_{LOAD}\$, then:
$$
V_{LOAD} = V_{BATT} * \frac {R_{LOAD} }{ R_{BATT} + R_{LOAD}}
$$
or, by rearranging:
$$
R_{BATT} = R_{LOAD} * ( \frac{V_{BATT}}{V_{LOAD}} - 1)
$$
All values on the right-hand side can be measured directly.
Note: On the schematic above, when SW1 is open (or \$R_{LOAD}\$ is absent), VM measures \$V_{BATT}\$; when SW1 is closed (i.e. \$R_{LOAD}\$ completes the circuit), VM measures \$V_{LOAD}\$
Note: There are a couple of things to consider, when choosing the value of the load resistor:
- It has to be low enough, so that the \$V_{LOAD}\$ measurement yields a useful result. With an internal resistance typically in the range of 0.05 ~ 1 ohm, a 100 ohm load resistor would drop 99+% of the voltage and would introduce a lot of error in calculation.
- It has to be high enough, so that the discharge current is not too high - batteries don't like that and may be permanently damaged if (near-)short-circuited for too long. I can't provide exact values, but anything under 1A should be OK.
- It has to be high enough, for the specific resistor package / wattage, so that the resistor itself does not catch fire in the process. The power dissipated in the resistor is approximately \$V_{BATT}^2/R_{LOAD}\$
A common 10 ohm 1/4W resistor should be fine for a quick test of AA/AAA batteries: Discharge current is 0.12 ~ 0.15A, dissipated power is 0.144 ~ 0.225W. To be on the safe side, a 1/2W resistor would be a better choice.


