0
\$\begingroup\$

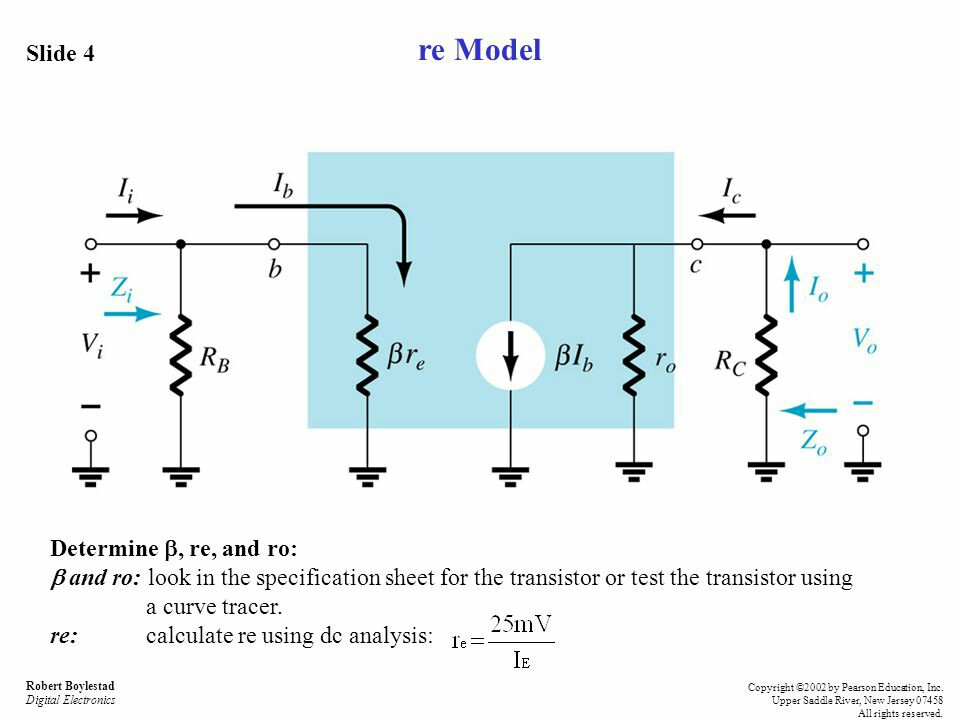
enter image description here

This is a picture of a Common Emitter Voltage Divider bias and it is been modelled using a CCCS and a diode resistance using the r_e model. Now my doubt is that I get problem in finding the input impedance/current and output impedance/current for the above model. How should I approach and how should I think and apply which techniques? What should I do so that I don't need to memorize and model the transistor myself for different configurations?

\$\endgroup\$
25
  • 1
    \$\begingroup\$ Well, I'm confused about the question. Perhaps others aren't. I can't speak for them. But you've flummoxed me up to this point. \$\endgroup\$
    – jonk
    Commented Nov 30, 2018 at 5:41
  • 1
    \$\begingroup\$ Are you aware that you are only using the linearized hybrid-\$\pi\$ model? You need a DC operating point. That is, in some sense, specified by the value of \$r_\pi=\beta\:r_e\$ (or else \$r_e\$.) That's an input to analyzing with the model. Not an output of it, as I understand things. \$\beta\$ is another such input. \$\endgroup\$
    – jonk
    Commented Nov 30, 2018 at 5:58
  • 1
    \$\begingroup\$ The words don't really matter and I'm not a linguist nor an expert on electronic terminology. You could know the model in every language in the world and still know nothing at all about the model, itself. So words aren't important. Just images, pictures, concepts, and derived mathematical results. Perhaps we are beginning to go in circles now. If so, I'll wait until there is a break in the circling. The bottom line is that I don't know what you are asking about. Others may differ in that. But I think we are wasting time on etymology now. \$\endgroup\$
    – jonk
    Commented Nov 30, 2018 at 6:18
  • 1
    \$\begingroup\$ As jonk says - words don`t really matter, but I think, it is important to avoid mis-interpretations of words and terms. Therefore: The models under discussion are NOT the so-called r_e model. In the r_e model (which I do not like at all, because it does not reflect the physical reality) the most important transistor parameter (transconductance gm) is modelled as a dynamic/differential "resistor r_e=1/gm". \$\endgroup\$
    – LvW
    Commented Nov 30, 2018 at 8:12
  • 1
    \$\begingroup\$ Continued: But this model gives not the correct picture and causes confusion (this is my teaching experience!), because the inverse transconductance (1/gm) is not a resistance at all (it only has the unit "Volt/Ampere" because it connects the B-E voltage with a current that does not exist between B and E but between E and C. \$\endgroup\$
    – LvW
    Commented Nov 30, 2018 at 8:13

2 Answers 2

Reset to default
0
\$\begingroup\$

You have an unloaded CE amplifier.

DC Biasing gives you \$I_E\$, which allows you to determine \$r_e\$.

You would probably be given a Table for input impedance \$Z_i\$, output impedance \$Z_o\$, voltage gain \$A_V\$ and current gain \$A_I\$ for the variety of biasing circuits your instructor expects you to cover.

In the CE \$r_e\$ model, there is no connection between input and output. So Input impedance is: $$Z_i = R_B \parallel \beta r_e$$

Output impedance looks back into circuit with 0 input.

$$Z_o = r_o \parallel R_C$$

If \$r_o\$ >> \$R_C\$ (by a factor of 10+) then output impedance can be simplified to:

$$Z_o \approx R_C$$

This simplification can be made because the Q-Point will vary with \$\beta\$, temperature, tolerances.

Voltage Gain (from a formula sheet).

$$ A_v = - \frac {r_o \parallel R_C} {r_e} $$ $$ A_v \approx - \frac {R_C} {r_e} ,\ if\ r_o >> R_C$$

You can use \$Z_i\$, \$Z_o\$, and \$A_V\$ to form the Generalized Model to simplify solving currents (but that is not your question).

Use current division with the \$r_e\$ model to solve for currents.

$$ I_b = I_i\ \frac {R_B} {R_B + \beta r_e} $$

That gives you \$\beta I_b\$.

\$I_o \approx \beta I_b\$ or:

$$ I_o = \beta I_b\ \frac {r_o} {R_C + r_o} $$

\$\endgroup\$
3
  • \$\begingroup\$ Sir, is I_o=β. I_b and approximation when r_o is 10 times larger than Rc. \$\endgroup\$
    – John Cena
    Commented Dec 1, 2018 at 16:52
  • \$\begingroup\$ Yes, but if \$R_C\$ << \$r_o\$, the difference is <10%. Given variance in \$\beta\$, this approximation is more than valid, since Q-Point will move. No theoretical calculation with transistors will every be met by an actual circuit. \$\endgroup\$
    – StainlessSteelRat
    Commented Dec 1, 2018 at 17:53
  • \$\begingroup\$ I've edited the answer adding \$\approx\$ as required. \$\endgroup\$
    – StainlessSteelRat
    Commented Dec 1, 2018 at 18:12
0
\$\begingroup\$

@ John Cena

The derivative of the deltaV_diode / deltaI_diode (the AC resistance, the 're', what I call the 'reac', and 1/tranconductance) is:

26 ohms if the DC diode current is 1 milliamp

260 ohms if the DC diode current is 0.1 milliamp

2,600 ohms if 0.01mA (10uA)

\$\endgroup\$
1
  • \$\begingroup\$ The question uses \$r_e\$ = 25mV/\$I_E\$ in mA. \$\endgroup\$
    – StainlessSteelRat
    Commented Nov 30, 2018 at 17:17

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.