4
\$\begingroup\$

I am trying to design a minimalistic current source to drive an LED from a lithium polymer battery and with a small number of components to minimize board space usage. First I wanted to use the same type of current source shown in the datasheet of the LT3080 : LT3080 current source

then I noticed the LT3080-1 is the same but with a 25 mOhms resistor in the output of the functionnal diagram. So my question is : Can I use LT3080-1 as current source with only the integrated 25 mOhms as output resistor?

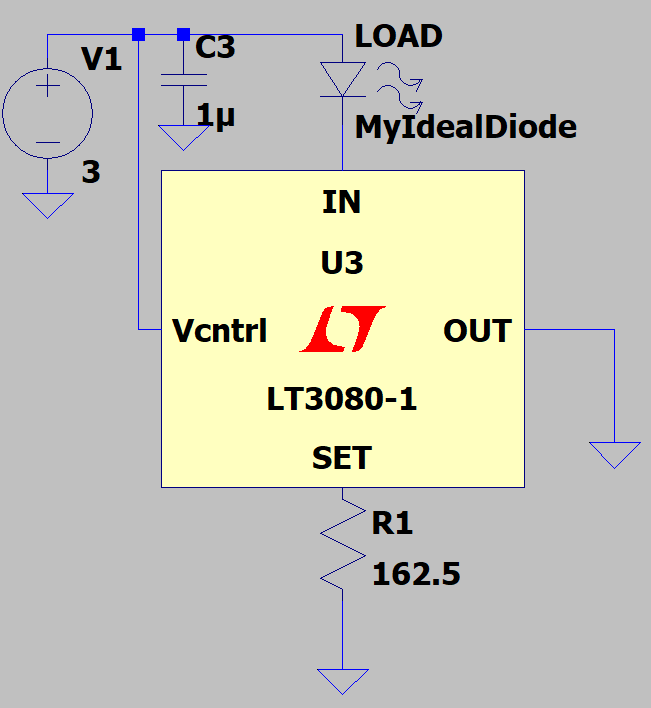
It seems to work in LTSPICE with the following schematic with a 3.2 voltage source (not with 3 as on the picture) for a 65 mA current source (although there is a slight offset, which is okay for my application and can be accounted for anyway) : LT3080-1 current source

But I am wondering if maybe there are imprecisions which will prevent repeatability of current accuracy in production? The current reference inside the component seems precise enough but I don't know the accuracy of the 25 mOhms resistor.

I want to use this component because of the low voltage drop (~0.3V) and the fact that I am driving an LED from a LIPO battery (4.2V max, 3.2V min) with a Vf= 2.9V. The 25 mOhm resistor also minimizes the voltage drop around the output resistor.

I don't want to use buck boost LED drivers because they are even more expensive and require more components.

\$\endgroup\$

1 Answer 1

Reset to default
1
\$\begingroup\$

According to the passage on p.10 of LT3080-1 datasheet,

As shown in Figure 4, the two devices have internal ballast resistors, which at full output current gives better than 90 percent equalized sharing of the current.

This seems to imply that the tolerance of internal 25-mOhm ballast resistors is about +-5%.

\$\endgroup\$
2
  • \$\begingroup\$ Thanks for the answer, can you please elaborate? \$\endgroup\$
    – Flabou
    Commented Dec 9, 2018 at 22:40
  • \$\begingroup\$ @Flabou, what to elaborate? It might depend on their interpretation of "90% sharing equilization". My interpretation is: If one part gives 50% and another 50%, it is 100% equalized. If one part gives 45% and another 55%, , it would be 80% in my books. Therefore the equalization must be something like 47.5% and 52.5%, or 2.5/50 = 5%. \$\endgroup\$
    – Ale..chenski
    Commented Dec 9, 2018 at 23:59

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.