5
\$\begingroup\$

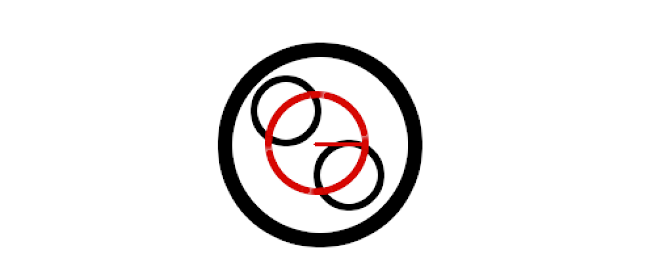
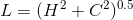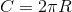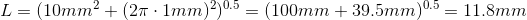Sometimes we might need to know the exact length of the wire pairs inside a cable. One can be interested in calculating voltage drop or some other calculation regarding the exact length.

Because the wires inside the cable are twisted, their actual(electrical) length is greater than the cable jacket length.

Is there any practical method to calculate the actual length?

I couldn't find a duplicate, but if there is I will delete this question.

\$\endgroup\$
7
  • \$\begingroup\$ If you want to determine volt drop then measure the loop resistance. \$\endgroup\$
    – Andy aka
    Commented Nov 11, 2019 at 12:39
  • \$\begingroup\$ loosely twisted wires will not be "as long" as tightly twisted pairs. I've seen this effect in using a drill to create tight twists, and during the drill's rotation the wire is continually CONTRACTING. \$\endgroup\$
    – analogsystemsrf
    Commented Nov 11, 2019 at 13:23
  • \$\begingroup\$ What method you chose should take into consideration why you need the answer. Don't forget obvious things like looking at building plans. \$\endgroup\$
    – Chris Stratton
    Commented Nov 11, 2019 at 18:49
  • 1
    \$\begingroup\$ @joribama I have submitted a geometric math themed answer that you might find interesting. \$\endgroup\$
    – Shadetheartist
    Commented Nov 13, 2019 at 22:58
  • 2
    \$\begingroup\$ @Shadetheartist - I had noticed (and up-voted) already. That's exactly what I had in mind :) \$\endgroup\$
    – joribama
    Commented Nov 14, 2019 at 5:02

4 Answers 4

Reset to default
2
\$\begingroup\$

One easy and obvious way is to connect the cable at the end and measure the loop resistance with an ohmmeter. Of course you need to know the resistance of the cable.

The better and very precise way is to use a time-domain reflectometer (TDR). This device sends an impulse into the cable which is reflected at the (open) end. The time of the reflected signal is measured and because of constant wave propagation, the length of the cable is calculated.

\$\endgroup\$
2
  • 4
    \$\begingroup\$ One limitation of using TDR is that you need to know the cable's relative permittivity in order to use the proper propagation speed used in the length calculation. Any inaccuracy on the permittivity will result in an inaccuracy in the length estimation, albeit to a smaller degree due to the fact that the propagation speed is inversely proportional to the the square root of the permittivity. \$\endgroup\$
    – joribama
    Commented Nov 12, 2019 at 6:07
  • \$\begingroup\$ "Of course you need to know the resistance of the cable." And you also need a very good meter. Cable conductors are usually rather low in resistance, so measuring takes considerable finesse. A Kelvin connection is usually called for. \$\endgroup\$
    – WhatRoughBeast
    Commented Nov 12, 2019 at 18:49
5
\$\begingroup\$

From a purely geometric perspective you could calculate the length using the helical length equation.

enter image description here

Where H is the length of the twisted wire and

enter image description here

Where R equals the radius of the turns in the wire. Basically from the center of the twisted pair assembly to the center of one of the wires.

enter image description here

So if the wire makes a complete rotation around the center in 10mm, and distance between the center of the twisted pair and the center of a wire is 1mm, then were you to untwist the wire and straighten it the length would be

enter image description here

\$\endgroup\$
3
\$\begingroup\$

Cut off a 1 meter piece, remove and straighten one of its conductors, and measure the actual length per meter.

Update:

Straighten out one of the conductors and measure it. Say it's 1.05m long, or 5% (made-up number - I've no idea whether it's realistic) longer than the cable it came out of. Apply that extra 5% to the length of your cable to get the length of the conductors inside.

\$\endgroup\$
1
  • \$\begingroup\$ Could you please expand the answer? \$\endgroup\$
    – Voltage Spike ♦
    Commented Nov 12, 2019 at 16:16
1
\$\begingroup\$

I used the 2 probes of an oscilloscope to measure the propagation delay and found 16ns for a 1.8m long (external) Ethernet cable (and 26ns for 3m). Either vp=1.1e8 m/s (not the expected 2/3 co=2e8 m/s https://en.wikipedia.org/wiki/Velocity_factor) or the cable are (2/1.1-1)*e100 = 80% longer. I was not expecting such a large difference.

\$\endgroup\$

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.