"the resonance frequency in an RLC circuit is defined as the condition where the capacitive and inductive reactances cancel each other, resulting in a purely resistive impedance."
Actually, resonant frequency is a phenomenon that comes from math and it has something to do with the solutions to differential equation. You mention a parallel RLC circuit, so let's have a look at that.
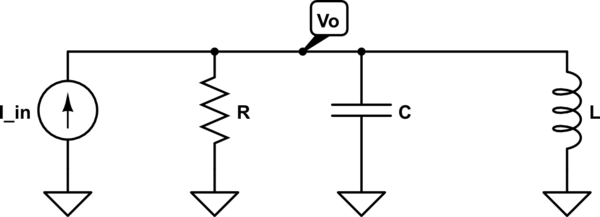
simulate this circuit – Schematic created using CircuitLab
From basic circuit theory, you should know the relationships: \$I_R = \frac{V}{R} \: \: I_C= C \frac{dV(t)}{dt} \: \: I_L=\frac{1}{L} \int V(t) \; dt \$.
Let's write up the relationship between the output voltage, \$V_o \$ and the input current \$I_{in} \$ in form of a node equation.
$$\frac{V_o(t)}{R} + C\frac{dV_o(t)}{dt} + \frac{1}{L}\int V_o(t) \; dt = I_{in}(t)$$
We don't want the integral in our equation, so we differentiate every term with respect to \$ t\$.
$$C \frac{d^2V_o(t)}{dt^2} + \frac{1}{R}\frac{dV_o(t)}{dt} +\frac{V_o(t)}{L} = \frac{dI_{in}(t)}{dt}$$
This is now a differential equation. We want it in standardform, so we divide with \$C \$ in every term.
$$ \frac{d^2V_o(t)}{dt^2} + \frac{1}{RC}\frac{dV_o(t)}{dt} +\frac{V_o(t)}{LC} = \frac{dI_{in}(t)}{dt}$$
And there is our final differential equation. Let's say we wished to find the natural response, that is, the solution to the differential equation when the right hand side is zero.
$$ \frac{d^2V_o(t)}{dt^2} + \frac{1}{RC}\frac{dV_o(t)}{dt} +\frac{V_o(t)}{LC} = 0$$
In order to find the solutions, we need the characteristic equation. Because this is a second order differential equation, with the coefficients \$\frac{1}{RC} \$ and \$ \frac{1}{LC} \$ the characteristic equation will look like this.
$$\lambda^2 + \frac{1}{RC}\lambda + \frac{1}{LC} = 0 $$
The roots of this equation as it is written right here are not so pretty
$$ \lambda = \frac{-L \pm \sqrt{-4R^2CL+L^2}}{2RLC} $$
However, look what happens if we define \$\alpha = \frac{1}{2RC} \$ and \$\omega_0 = \sqrt{\frac{1}{LC}} \$. The charateristic equation now becomes
$$\lambda^2 + 2\alpha \lambda + \omega_0 ^2 =0 $$
This equation has the solutions (roots)
$$ \lambda = -\alpha \pm \sqrt{\alpha^2 - \omega_0 ^2} $$
The roots are much nicer now. The term \$ \alpha\$ is called the damping coefficient, and \$\omega_0 \$ is called the undamped resonant frequency.
It turns out that if \$\alpha > \omega_0 \$ the solution to the homogenous differential equation is
$$V_o(t) = K_1 e^{\lambda_1 t} + K_2 e^{\lambda_2 t} $$
If \$\alpha = \omega_0 \$ the solution is
$$V_o(t) = K_1 e^{\lambda_1 t} + tK_2 e^{\lambda_2 t} $$
If \$\alpha < \omega_0 \$ the solution is
$$V_o(t) = K_1 e^{-\alpha t} \cos \bigg(\sqrt{\omega_0^2 - \alpha^2} \; t \bigg) + K_2 e^{-\alpha t} \sin \bigg(\sqrt{\omega_0^2 - \alpha^2} \; t \bigg) $$
If you have experience with solving 2nd order differential equations this will make sense to you.
As you can see, the resonant frequency \$\omega_0 \$ is saying a lot more than just "where the resulting impedance is purely resistive", and it is defined in a different way than what you initially thought.
It just so happens that in both a series and parallel RLC circuit (with only 1 of each component) at resonant frequency the equivalent impedance is purely resistive. Note, however, that this would not be the case for a, let's say, RRLC circuit, for example.