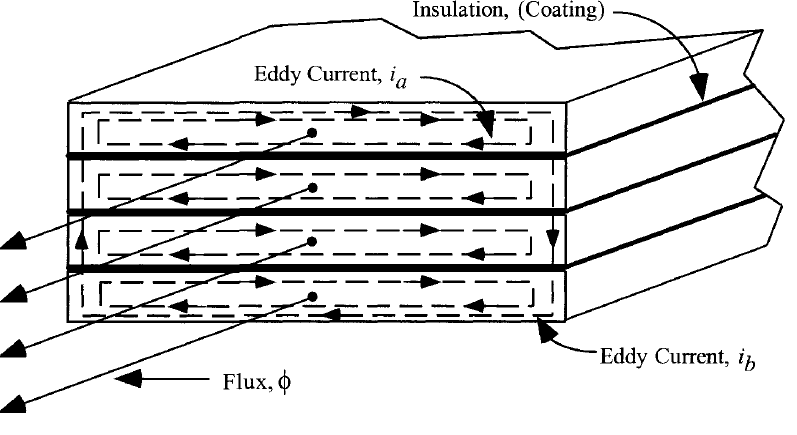
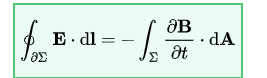Here is a transformer where we can see the eddy currents in the core:
I would like to estimate the voltage which induces the eddy currents. I know what voltage is applied to the primary and how much time this voltage is applied. The number of turns on the primary is 1,so I am able to determine what the variation of the flux is.
How can I estimate the voltage into the core as there is no winding, ie no turn?
-------------------------------EDIT--------------------------------