I hope this accurately reflects the problem:
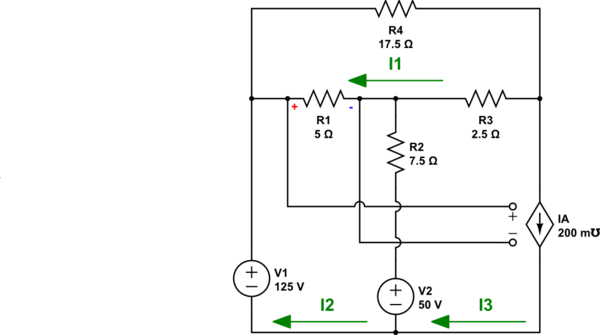
simulate this circuit – Schematic created using CircuitLab
Here's the initial mesh work straight from head to paper as fast as I can write it out:
$$\begin{align*}
0\:\text{V} -R_4\cdot I_1-R_3\cdot\left(I_1-I_3\right)-R_1\cdot\left(I_1-I_2\right) &= 0\:\text{V}
\\\\
0\:\text{V} +V_1 -R_1\cdot \left(I_2-I_1\right)-R_2\cdot\left(I_2-I_3\right)-V_2 &= 0\:\text{V}
\\\\
0\:\text{V} +V_2 -R_2\cdot \left(I_3-I_2\right)-R_3\cdot\left(I_3-I_1\right)-V_{IA} &= 0\:\text{V}
\\\\
I_3&=0.2\cdot R_1\cdot\left(I_2-I_1\right)
\end{align*}$$
That's four equations and four unknowns. (Since you know that \$R_1=5\:\Omega\$, you could just go around the first three equations replacing \$I_3\$ with \$I_2-I_1\$ and reduce it to three equations and three unknowns.)
These solve out in the following way (using a free tool called sympy):
var( 'r1 r2 r3 r4 i1 i2 i3 via v1 v2' )
eq1 = Eq( 0 - r4*i1 - r3*(i1-i3) - r1*(i1-i2), 0 )
eq2 = Eq( 0 + v1 - r1*(i2-i1) - r2*(i2-i3) - v2, 0 )
eq3 = Eq( 0 + v2 - r2*(i3-i2) - r3*(i3-i1) - via, 0 )
eq4 = Eq( i3, 0.2*r1*(i2-i1) )
ans = solve( [eq1, eq2, eq3, eq4], [i1, i2, i3, via] )
for v in ans: v, ans[v].subs( {r1:5, r2:7.5, r3:2.5, r4:17.5, v1:125, v2:50} )
(i1, 3.60000000000000)
(i2, 13.2000000000000)
(i3, 9.60000000000000)
(via, 62.0000000000000)
So, given the direction of \$I_2\$ and \$V_2\$'s polarity, it's pretty clear that the power dissipated by \$V_1\$ is \$-V_1\cdot I_2=-125\:\text{V}\cdot 13.2\:\text{A}=-1650\:\text{W}\$. It's supplying power to the rest of the circuit. And the number matches up with the book value (at least in magnitude, if not sign.)
Just as a double-check, if the current \$V_2\$ is taken as exiting its positive terminal, then \$I_{V_2}=I_3-I_2=-3.6\:\text{A}\$. So the power is positive and therefore \$V_2\$ is dissipating. So you do not need to add it in.
Likewise, the current in \$I_A=I_3=9.6\:\text{A}\$ and voltage across it is \$62\:\text{V}\$. Given the polarity and direction this is also a dissipating element.
So there really is only one circuit element powering things, \$V_1\$. And you have the answer.
Please feel free to go through what I wrote, and what you did, and find your own error. It was less work for me to write this up than to parse your red script.


