2
\$\begingroup\$

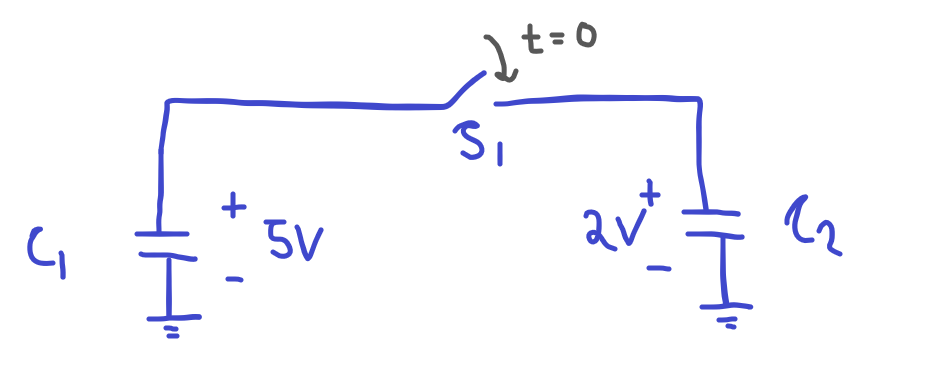
I have a confusion about this circuit:

enter image description here

Assume C1, C2 and S1 (in particular) is ideal. Assume S1 has an ideal 0 on resistance.

Capacitor C1 is charged to 5V and C2 is charged to 2V. At time t=0, the switch is closed and current flows from C1 to C2 in a transient phase. A steady state condition is reached where the voltage across both caps will settle to a value based on the capacitor ratios and their initial charge.

My question is about exactly at time t = 0. As soon as the switch is closed, the top plates of C1 and C2 are basically the same node/net (ignore any resistances, I know there will be resistances of the wires/tracks and the switch), yet current is only starting to flow, so what exactly is the voltage on this net at this time t = 0, is it 5V or 2V?

\$\endgroup\$
3
  • 1
    \$\begingroup\$ Does this answer your question? What will happen when two capacitors C1 and C2 are added in series instantly by a switching mechanism, How the charge sharing will take place? \$\endgroup\$
    – D.A.S.
    Commented Feb 10, 2022 at 1:36
  • \$\begingroup\$ @TonyStewartEE75 That other question is asking about capacitors in series and is unclear about whether it's asking about real or ideal circuits. This question is asking about capacitors in parallel and is unambiguously asking about ideal circuits. \$\endgroup\$
    – Sophie Swett
    Commented Feb 10, 2022 at 2:23
  • \$\begingroup\$ @Super, your question presumes a single node exists at the instant the ideal switch connects and is based on a simplification. A physics answer will be quite different and accurate. But the simplified (cartoon) models used throughout the more commonly applied electronics analysis tools fail here. \$\endgroup\$
    – jonk
    Commented Feb 10, 2022 at 7:19

1 Answer 1

Reset to default
2
\$\begingroup\$

Presuming you are asking about the voltages at the instant just after the switch closes (conventinally called t0+).

At that time, in an ideal circuit, the voltage cannot be determined as there is an indeterminate condition -- the capacitor voltages are equal which implies that they changed instantaneously. This implies an infinite current.

Normal circuit analysis is not possible with an infinite current.

If you assumed that there is some additional inductance or resistance in the switch, the instantaneous infinite current would not happen and the voltages could be analyzed normally -- there would be an exponential approach to the final (equal) capacitor voltages.

\$\endgroup\$
2
  • 1
    \$\begingroup\$ correct. Or as my professor used to say: voltages of a capacitor can not jump (not change in an infinitesimal short time step) - and the same applies to inductors and currents. Caps have a phase of -90°. This means first there must be a current transporting charge for a voltage to change. So connecting 2 Caps with different voltages and no resistance is simply impossible, as voltages at t(0) can not change without a current at t(-x) prior to closing the switch. Also given no resistance, a node can not have two different voltages at the same time \$\endgroup\$
    – schnedan
    Commented Feb 10, 2022 at 9:05
  • \$\begingroup\$ Understood. Thanks! \$\endgroup\$
    – SuperConductor47
    Commented Feb 10, 2022 at 11:41

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.