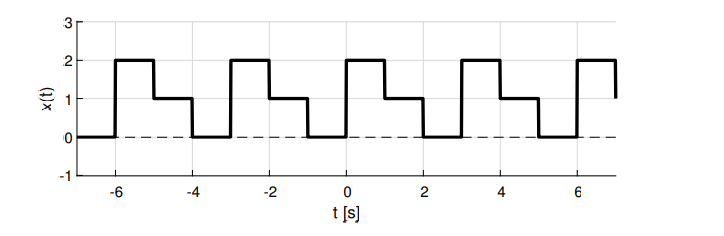
I am trying to find the power spectral density, autocorrelation function, and power content of a periodic signal. In order to do this I need to find the Fourier series coefficients.
I am trying to solve the problem analytically, but when I find the closed form of the signal x(t), I found j dependency in the signal. Whatever I do, I cannot get rid of the complex part. Can a real signal have j dependency? How can I find x(t) for this signal analytically from its Fourier series?