The follow-up question...
but what I don't understand is why the flow of electrons that are physical entities result in emission of these EM waves
Why does "radiation" occur?
Let's look at this specifically, because it is a common (and excellent) concern.
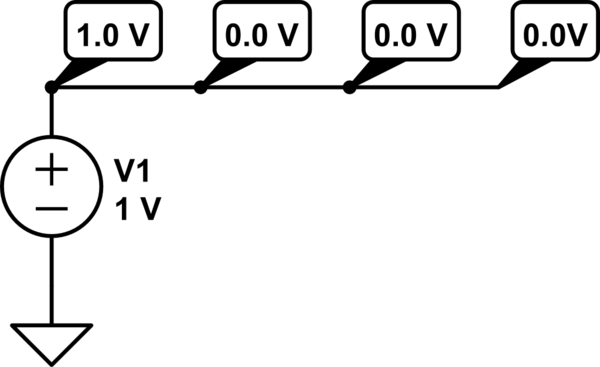
Here is a simple wire, instantly connected to a voltage source:
simulate this circuit – Schematic created using CircuitLab
At this moment in time, the potential difference between the left-end of the wire (adjacent to the source) and ground is 1 volt.
The extreme other end of the wire is still at ground (0 difference) because the electromotive force (voltage) of the source has not yet propagated to the other end of the wire.
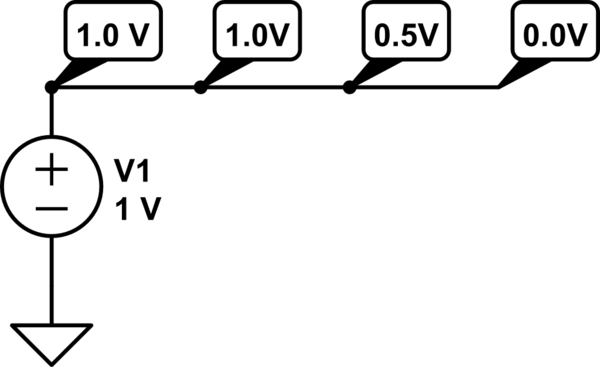
As time goes on, the voltage down the wire increases:
simulate this circuit
The electrons in the conductor are being accelerated by the electric field (the potential energy of the source being converted into kinetic energy in the electrons).
When the electrons reach the end*, they can't physically continue -- there's no more conductor to propagate along!
...but these charges have momentum in the direction of the wire (e.g. there is kinetic energy).
When the charges come to an abrupt stop at the end of the wire, the conservation of energy law requires that this energy must "go somewhere" -- it can't just disappear!
The answer is radiation. The energy leaves the end of the wire in the form of an electro-magnetic wave.
*It should be noted that the same electrons that start moving at one end of the wire are not necessarily the same electrons that reach the other end of the wire, but this isn't material to our discussion.
The fallout
A lot of neat things fall out of this. For example, you could think of the wire in our example as being composed of infinitely many smaller wires. For each of these, the same behavior would hold true (which is why radiation occurs down the entire length).
You can also see why radiation results from a change in the electro-magnetic field (e.g. from a change in current).
You can understand how linear antennas work. In our example, now imagine that just at the moment when the voltage peaks at the far end, we switch the source back to 0.0V. You would now have the identical picture but flipped over (1.0V on the right, 0.0V on the left) and the process would begin again.
Keep repeating this process and the electrons would be endlessly running back and forth (over the whole wire length) from one end to the other. That's a perfect linear antenna ("radiator").
If the wire were too short, there would be less movement and if too long, there would be too much. The voltage would keep increasing further down the wire as you reduce the voltage in the nearby section (interference results, which is hard to visualize with just these simple figures).
Now you can intuit trace behavior...
What I understand is that the board trace is essentially starting to behave as an antenna in this case but I do not know the reason.
At low frequencies (really, low edge-rates in "digital" circuits), the electrons have time to reach the end of the wire before the source is switched around and the electrons are asked to come back. This is called a "lumped element."
The voltage at each end of the wire is basically always the same. This is the behavior we teach to introductory electronics students (a wire is an equipotential surface = same voltage everywhere).
As the frequency increases, they have less and less time to make the trip and the voltage at each end of the wire can no longer be guaranteed to always be the same as shown in the previous figures.
In circuit board design, you don't need to worry much about radiation from lumped elements. A simple approximation is:
- Find the fastest rise-time (1/edge-rate) in your signaling = Tr
- Find the maximum frequency contained in this edge = f
- Keep tracks an order-of-magnitude shorter than the corresponding wavelength
That is:
$$f = \frac{1}{2T_r}$$
$$\lambda = \frac{c_m}{f}$$
$$l_{track} < \frac{\lambda}{10} = \frac{T_r c_m}{5}$$
where c_m is the speed of light in the medium (typically for a copper over FR-4 PCB c_m is approximately 1.5e8).