I got to know that in signal processing we use j\$j\$ to represent a phase of π/2\$\pi/2\$ in between two real signals which are being transmitted. This also conforms to the fact that to find signal energy we find the magnitude first and then square it to time integrate.
But, in transmission lines when we represent a wave as a complex function in time and space, for power delivered to the load we take only the real part of VI^∗ (V*I conjugate)\$VI^∗\$ and half it . So my question is what is the significance of the complex part in the wave and how is it different from signals?
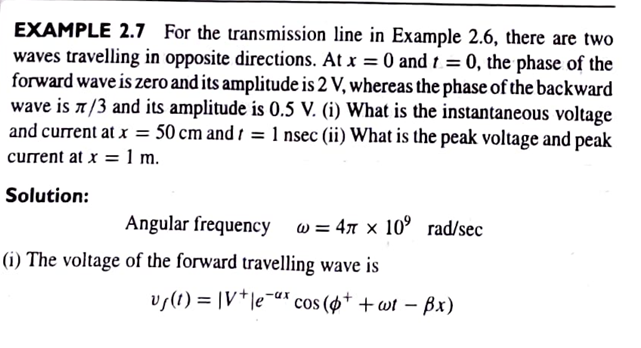
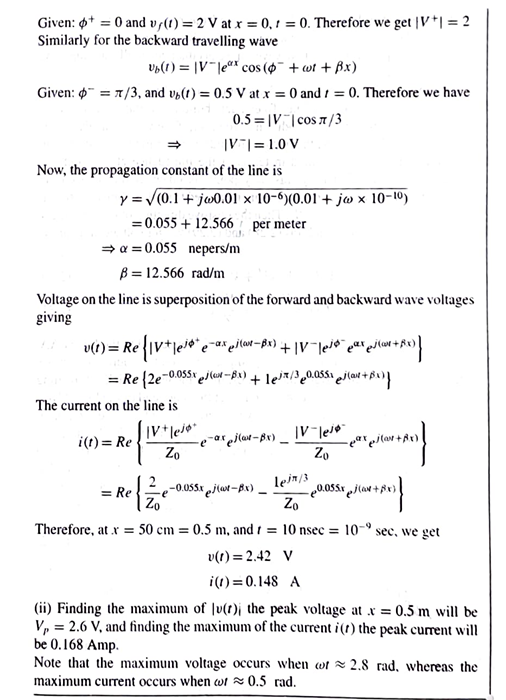
Above is a attached screenshot of a question from a book(Electromagnetic Waves - By R K Shevgaonkar, published by Tata McGraw-Hill, fourth reprint 2010, pg 18-19) which further clarifies my question as we see that only real part of the general wave equation is taken even for calculating voltage and current at a certain instant of time and position in space. So why do we not consider the complex part?