0
\$\begingroup\$

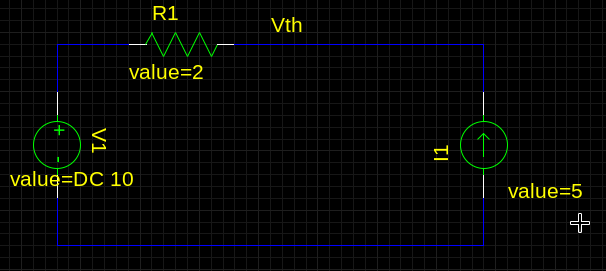
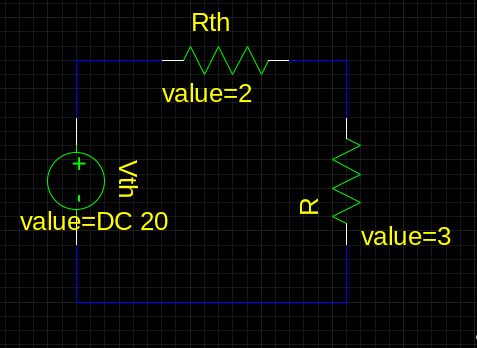
The question wants me to calculate the \$V_3\$ value,and the solution is as below.

Solution:

\$V_3=(\frac{10}{2}+5)(2//3)=12V\$

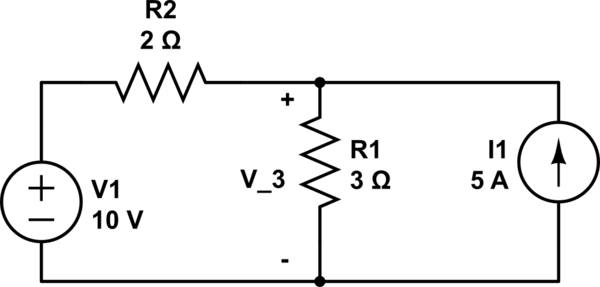
schematic

simulate this circuit – Schematic created using CircuitLab

However, here is my thinking. My answer is not the same as solution's, but I don't know where am I wrong. Can anyone tell me where am I wrong?

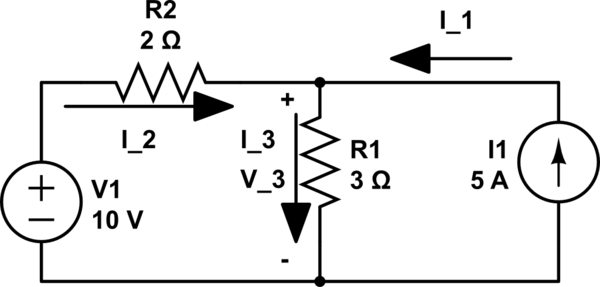
schematic

simulate this circuit

\$V_3=I_3\times R_1\$,and \$I_3=I_1+I_2=5+(\frac{10}{R_1+R_2})=5+(\frac{10}{2+3})=5+2=7\$,

so \$V_3=I_3\times R_1=7 \times 3=21V\$

Where am I wrong? I think my calculation should be correct, but the my answer is wrong.

\$\endgroup\$
7
  • 2
    \$\begingroup\$ If you converted I1 and R1 into a voltage source, the solution is clearly seen without putting pen to paper even. \$\endgroup\$
    – Andy aka
    Commented Apr 24, 2020 at 15:26
  • 2
    \$\begingroup\$ Did you learn about the Thevenin and Norton equivalent circuits yet? What Andy is talking about is converting I1 and R1 into the Thevenin eqivalent voltage source. You can also convert V1 and R2 into the Norton equivalent current source. Either way will make the problem much easier. \$\endgroup\$
    – user57037
    Commented Apr 24, 2020 at 17:31
  • \$\begingroup\$ But if you haven't learned that yet then you need to solve equations, I guess. \$\endgroup\$
    – user57037
    Commented Apr 24, 2020 at 17:32
  • \$\begingroup\$ shineele, please use better titles. Also, your previous questions got lots of comments and answers with knowledge that you're not applying here \$\endgroup\$
    – Marcus Müller
    Commented Apr 24, 2020 at 21:24
  • 1
    \$\begingroup\$ The one lesson to take from this is to never declare your reasoning as right when the results are wrong. That thinking leads to vanity, and vanity is blindness. Doubting yourself is not a sign of weakness, it's a process of learning. Since doubts will never fully be gone, since we keep on learning until we die, don't be afraid to doubt yourself -- it leads towards improvement. \$\endgroup\$
    – a concerned citizen
    Commented Apr 25, 2020 at 5:55

5 Answers 5

Reset to default
2
\$\begingroup\$

You’ve made a mistake in your nodal analysis equation. \$I_2\$ is not simply \$\frac{V_1}{R_1 +R_2}\$ as it’s not just those two resistors in the circuit. I’ll point out that \$I_2=\frac{V1-V3}{R_2}\$ because this is ohms law. I’ll leave it up to you to determine \$I_3\$ and \$V_3\$.

\$\endgroup\$
1
  • \$\begingroup\$ thanks a lot ,now i really know where am i wrong!! you got a point! \$\endgroup\$
    – shineele
    Commented Apr 25, 2020 at 1:43
2
\$\begingroup\$

You assumed that the current through R2 was equal to

\$\frac{10}{R1+R2}\$

That is incorrect.

\$\endgroup\$
0
1
\$\begingroup\$

You assumed that source I1 doesn't at all affect I2. That's totally wrong. I2=(V1-V3)/R2 and V3 depends on I1.

You should write an equation which is based on circuit laws, for example assume V3 is unknown and write the currents I1 and I2 together are equal with V3/R1.

The given right solution in the question converts V1 to current source and that's combined with I1.

\$\endgroup\$
2
  • \$\begingroup\$ so you mean that \$I_1+I_3=I_2\$.not \$I_1+I_2=I_3\$ ? \$\endgroup\$
    – shineele
    Commented Apr 25, 2020 at 1:27
  • \$\begingroup\$ NO! I1 and I2 together =I1+I2 and V3/R1=I3. That's not my innovation, Ohm and Kirchoff invented it. The equation I suggested is I1+(V1-V3)/R2=V3/R1. BTW you have already accepted an answer which contain the same as my answer but not all of it. – user287001 9 mins ago \$\endgroup\$
    – user136077
    Commented Apr 25, 2020 at 7:34
0
\$\begingroup\$

You can solve it using the Thevenin theorem.

  1. First remove your target resistance and calculate the thevenin voltage, which results in Vth = 20v

enter image description here

  1. Then calculate the thevenin resistance, which ends up being simply: Rth = 2 ohm. You must remember that in order to do this you have to shortcircuit the voltage source and open the current source.

enter image description here

  1. Finally your circuit will look like the image below and you will have to get V3 just like you would do with a voltage divider.

V3 = Vth ( Rth / R + Rth ) = 20 ( 3 / 3 + 2 ) = 12v

enter image description here

With some practice you will be able to perform this process only thinking, no need to write equations.

\$\endgroup\$
3
  • 1
    \$\begingroup\$ The OP asked where he'd gone wrong not how to solve it using a different method -1 \$\endgroup\$
    – Andy aka
    Commented Apr 24, 2020 at 18:13
  • \$\begingroup\$ Since the wrong part was already pointed, I just proposed a solving method that fits better to the problem. \$\endgroup\$
    – Marcelo Coronel
    Commented Apr 24, 2020 at 18:25
  • 1
    \$\begingroup\$ Best left as a comment then (as I did 3 hours ago). \$\endgroup\$
    – Andy aka
    Commented Apr 24, 2020 at 18:35
-2
\$\begingroup\$

Let's solve this using superposition:

Turn off I1 (leaves an open circuit) -> this leaves you with a resistive divider circuit where the V_R1_Part1 is 6V

Turn on I1 again and turn off V1 (leaves a short circuit) -> leaves you with the two resistors in paralell to each other paralell to the current source. Combined resistance of the two is 6/5 Ohms. --> Voltage over both resistors is 6V (V_R1_Part2 = 6V)

Add the two parts of your solution. V_R1_part1 + V_R1_part2 = 6V+6V=12V

\$\endgroup\$
1
  • 3
    \$\begingroup\$ Please do not turn this into a homework solution site. This is not Chegg. We expect the OP to do a significant amount of their own work. \$\endgroup\$
    – Elliot Alderson
    Commented Apr 24, 2020 at 15:38

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.