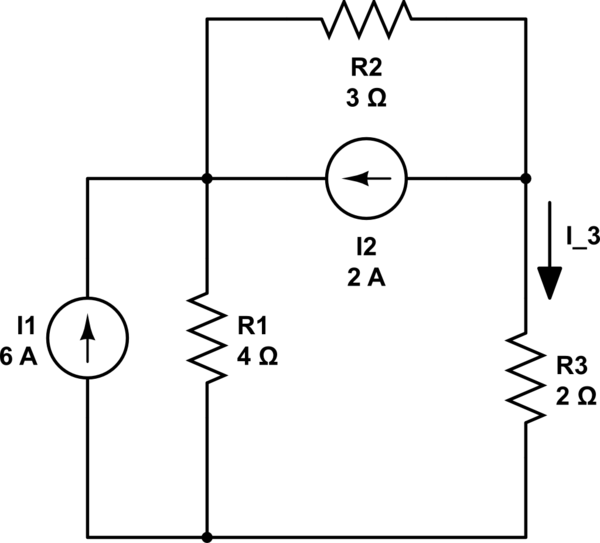
You are right, in a way. There is \$8\:\text{A}\$ arriving into the shared node of \$I_1\$ and \$I_2\$. And if you imagine that the sum of the currents in \$R_1\$ and \$R_2\$ must also be \$8\:\text{A}\$ then you'd be right about that, too. However, you write, "...is divided across two parallel resistors." And this is, I think, at least one point where you make an error. \$R_1\$ and \$R_2\$ are not in parallel.
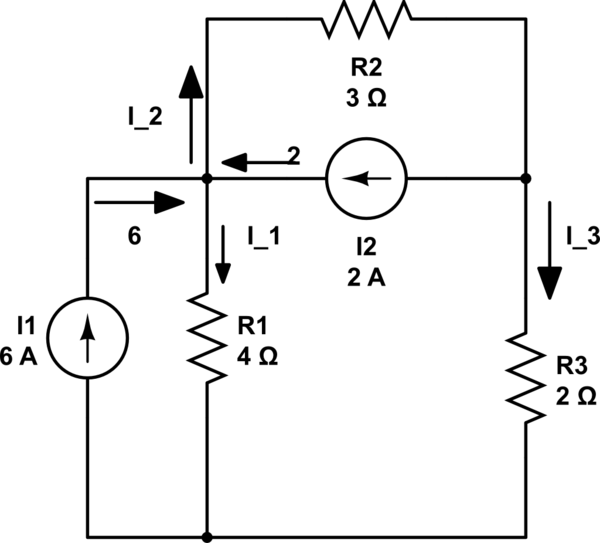
Before I continue, let's note first that you are always allowed to designate exactly one node as \$0\:\text{V}\$ (or "ground.") So I want to redraw the schematic:

simulate this circuit – Schematic created using CircuitLab
That said, you can observe that the sum of the currents in \$R_1\$ and \$R_3\$ (you know the direction, I assume) must also be the same as \$I_1\$ or \$6\:\text{A}\$. From simple inspection, we can say:
$$\begin{align*}
I_{\text{R}_1}+I_{\text{R}_2}&=8\:\text{A}\\
6\:\text{A}&=I_{\text{R}_1}+I_{\text{R}_3}\\
2\:\text{A}+I_{\text{R}_3}&=I_{\text{R}_2}\\
I_{\text{R}_2}\cdot R_2 &= I_{\text{R}_1}\cdot R_1-I_{\text{R}_3}\cdot R_3
\end{align*}$$
That's a little "over-specified" with four equations when you only need two of the first three plus the last one. Regardless, you could wrestle with the above and get the answers you want for all three currents, now.
Or use just two equations and two unknowns using KCL:
$$\begin{align*}
\frac{V_1}{R_1}+\frac{V_1}{R_2}&=I_1+I_2+\frac{V_2}{R_2}\\\\
\frac{V_2}{R_2}+\frac{V_2}{R_3}+I_2&=\frac{V_1}{R_2}
\end{align*}$$
When done, you know the current in \$R_3\$ is \$I_3=I_{\text{R}_3}=\frac{V_2}{R_3}\$.