How can we drive an 8 ohm speaker if it can only support around 1.5-2 watts for an Audio Amplifier Circuit?

Question

Goal of the circuit:

• Must be able to deliver 1.5 W to the speaker (8 Ω) at 1 kHz.

First Problem

1.) how can we drive an 8 ohm speaker if it can only support around 1.5-2 watts? Because when we input 8ohms as the load the Vout decreases.

• Coupling capacitor formula we used is 1/2pi(lowest frequency)(resistance seen by capacitance) 20Hz was the lowest frequency I used.

Referencing to the image above. If we add a common emitter by our understanding it should increase the power but even with coupling capacitor, it won't shift down to AC.

The next step we did was thinking of adding a darlington pair, it did increase the gain, but it still doesn't want to make the AC waveform shift down to its negative half cycles for a safer speaker usage.

above is the darlington w/ ce and ef (1s)

above is without the darlington.

Summary

Our goal is to get the output that is the same in the photo below. note The RL (R6) is 100K ohms but we want that output if our desired Load is an 8ohms 2W Speaker, that can ultimately support 1.5-2W power for the speaker. What changes can we implement in our design to achieve this?

Answer 1

Its okay not to know what to do right away. But my advice is your darlington approach is okay however if you check LT spice your power is incredibly low from what your target 2W. I may resuggest unawriter advice to directly put push and pull amplifier. I would recommend a class AB see here for more info about this.

I would suggest to put it directly on your output. And try to play around with your values use your knowledge about capacitor coupling and biasing for this.

May I also suggest to check this post I have recently read it and it quite match your question.

Answer 2

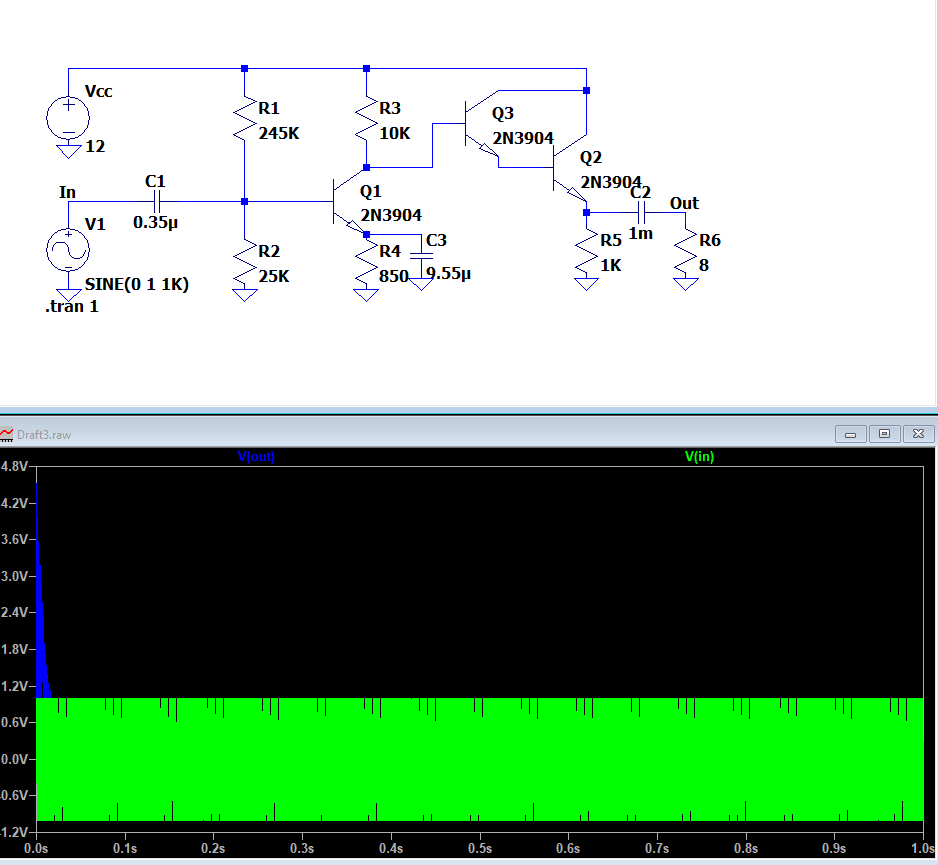
I sincerely wish you'd give up on the 2nd stage -- the power output stage -- that you keep insisting on using. It's useful if and only if you want lots of heat and/or fire and/or a rapid unplanned/unscheduled disassembly.

The 2nd stage is not salvageable. So I'm not wasting more time on it. You can read something I wrote, recently, here.

It's just wrong. Abandon it.

On the first stage, the main problem is that it is highly distorting, due to a widely variable voltage gain that depends upon the applied signal, itself.

Let's first look at the DC bias operating point (quiescent operation):

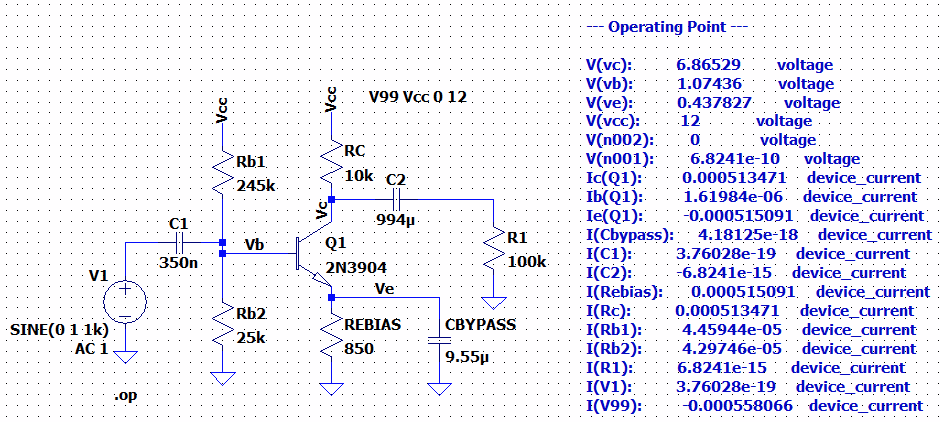
From that, and the emitter bypass capacitor arrangement, I can estimate the small signal voltage gain as about \$45\:\text{dB}\$ (for the case where the applied input signal is extremely tiny -- negligibly so.)

(I'm going to ignore the base biasing pair as they look stiff enough to me, upon first glance.)

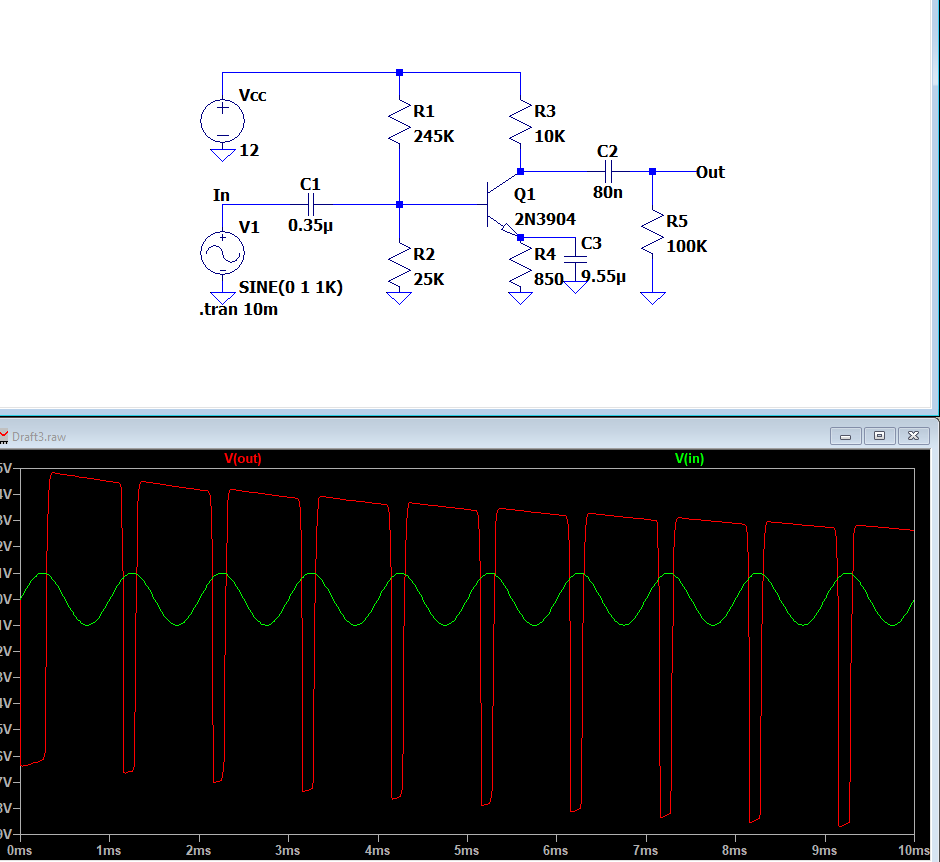
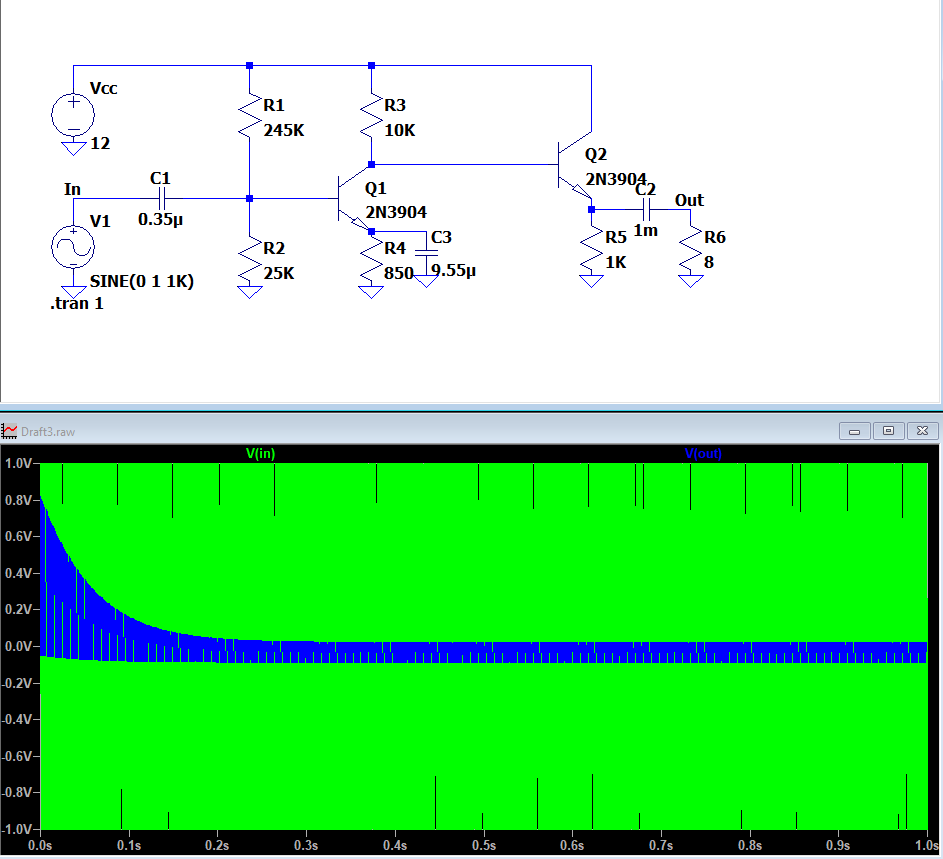
Based upon two facts observed from the DC quiescent bias point of \$V_{_\text{C}}\approx 6.9\:\text{V}\$ and \$V_{_\text{E}}\approx 440\:\text{mV}\$, and keeping in mind that the minimum \$V_{_\text{CE}}\approx 1\:\text{V}\$, I'd estimate that the maximum input signal magnitude can be no more than \$30\:\text{mV}\$ peak. Yet you are applying a test value of \$1000\:\text{mV}\$ peak! Right off the bat, that's not going to work.

Let's test my assertion:

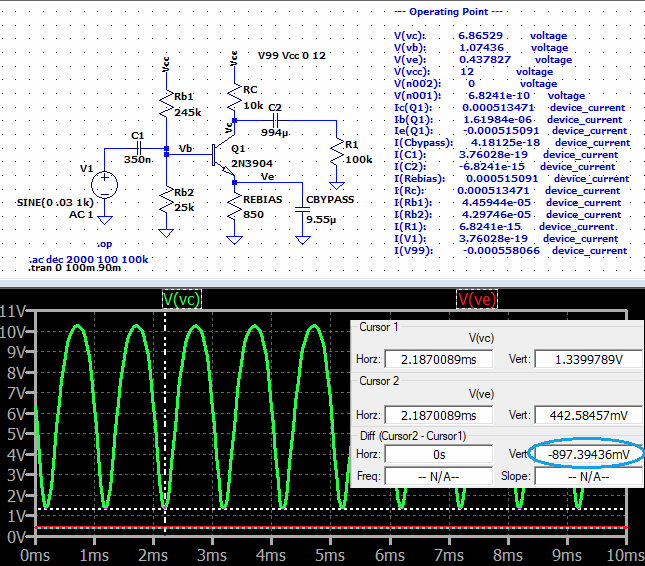
First off, yes. With an input of \$30\:\text{mV}\$ peak, we just break through my \$V_{_\text{CE}}\approx 1\:\text{V}\$ (to avoid the onset of saturation.) Close enough so that my approach is validated.

But second, notice the terrible distortion in the green curves? That's what this kind of stage does to an input signal when pushing it to its limits. This is because the voltage gain is highly variable with input signal, if the input signal deviates very far from its small signal assumption. And a \$30\:\text{mV}\$ peak obviously falsifies that assumption.

Earlier, I said the voltage gain is \$45\:\text{dB}\$. This would be truer for a \$1\:\mu\text{V}\$ input peak. But with the collector dropping to \$1.4\:\text{V}\$, the change in collector current is substantial (more than double) and this changes the voltage gain by the same factor, to almost \$53\:\text{dB}\$. Similarly, at the other end of the cycle the collector current drops by about a factor of 4 now and therefore the voltage gain drops to about \$33\:\text{dB}\$.

Those kinds of voltage gain changes mean distortion on an epic scale!

You just can't do that.

There are fixes. One fix is to dramatically reduce the input signal (I'll get to that, shortly.) Another would be to add global NFB (with another transistor added.) And another would be to place an AC emitter degeneration resistor in series with the emitter bypass capacitor. That will reduce the gain. But that's the price that has to be paid to keep this topology.

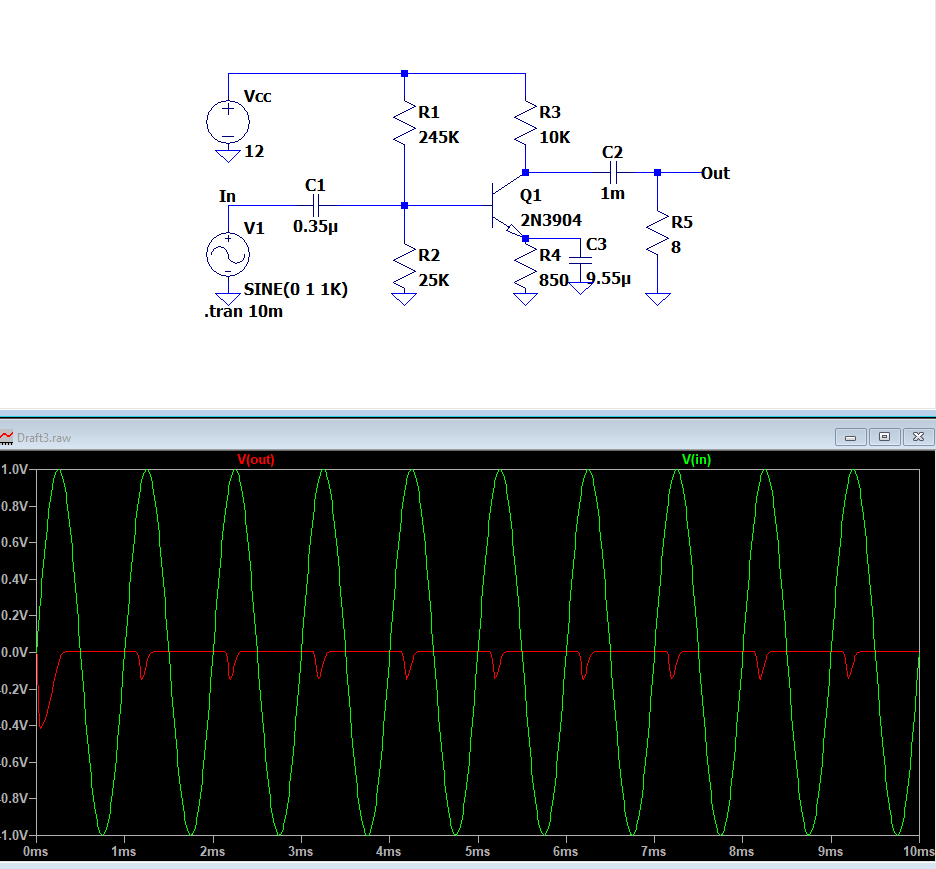
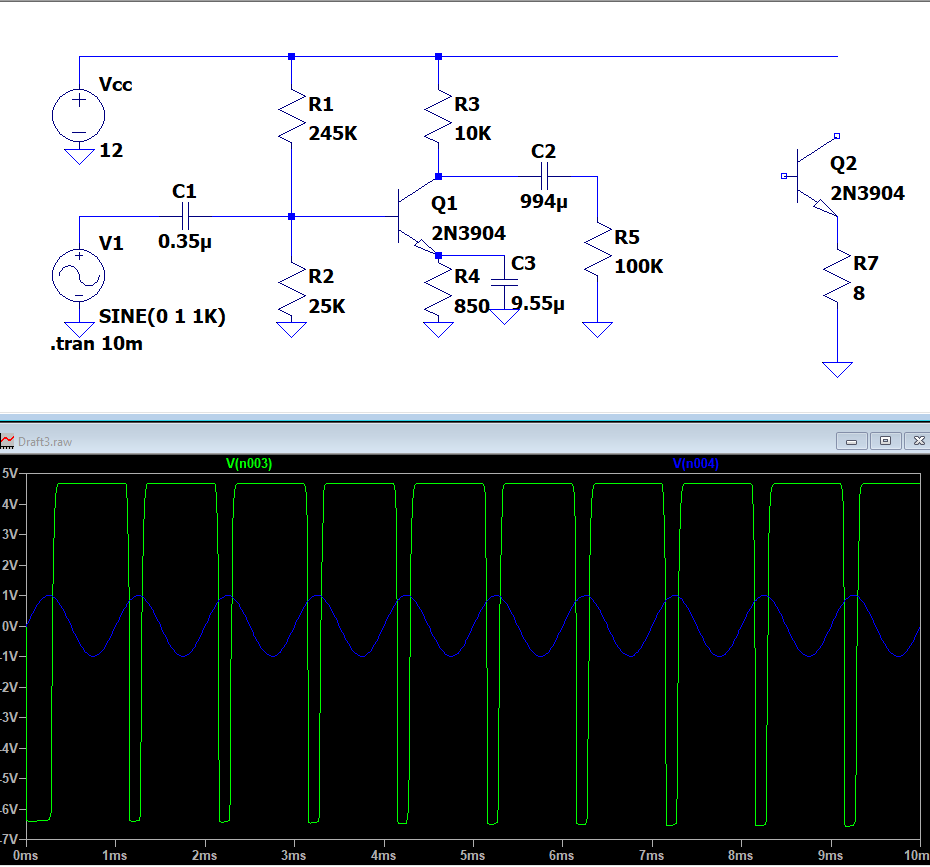
What signal level would be acceptable? That depends on how much distortion you are willing to accept. But let's say a gain variation of \$\pm 3\:\text{dB}\$ is acceptable (a factor of \$\sqrt{2}\$ in collector current, one way or the other.) Then the maximum input signal would be about \$9\:\text{mV}\$ peak!

Let's see:

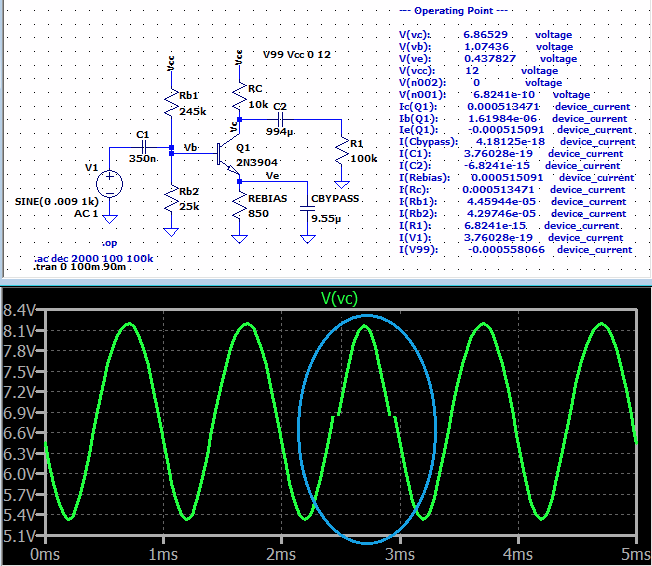
And that looks a lot better. But as you can see, where I flipped one half-cycle around in the center, it's still distorting the input signal enough to notice here.

So you have the above issues with respect to the 1st stage, which need solutions. And the 2nd stage is simply wrong and needs to be abandoned, entirely.

Answer 3

There are several problems with the base design, not the least of which is that a 2N3904 cannot supply the current needed without burning up (IRL).

In each of the schematic with an output emitter follower (not an output common emitter), the emitter resistor R5 is 1K. During positive half-cycles of the signal, the output current is supplied by the Q2 or Q2-Q3. During negative half-cycles, the current path is though R5 only. Thus there is a 1K resistor in series with the 8 ohm load.

If you reduce R5 to 1 ohm, 8 ohm load will have a much more symmetrical current path for the entire cycle. The circuit probably will simulate much better, although it will not work in real life.

Please add figure numbers to each image so we can be sure which schematic is being discussed.