Source voltage in discharging capacitor equation

Question

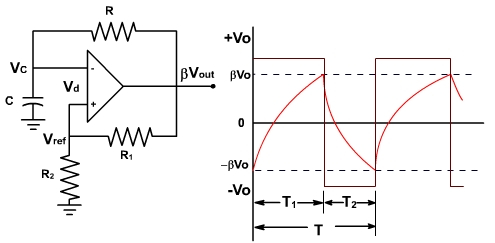
The voltage across a discharging capacitor in an RC network is

$$V_c = V_s (e^{-\frac{t}{RC}})$$

Rearranging this equation for t gives

$$t = -RC \ln \left( \frac{V_c}{V_s} \right)$$

Say I have a capacitor charged such that the voltage across it is 3.6V and I want to find the time it takes to reach 0.9V. I remove the voltage source and the capacitor begins to discharge. Since I have removed the source, what is the value of \$V_s\$ in this equation, and how do I use it to find the time it takes the capacitor voltage to reach 0.9V?

Answer 1

The more general form of the first equation is

$$v_C(t) = v_C(0)\cdot e^{\frac{-t}{RC}}$$

This must be so since, for \$t=0\$

$$e^{\frac{-0}{RC}} = 1$$

So, assuming the capacitor has charged up to the source voltage before you disconnect the source at \$t=0\$, we have

$$v_C(0) = V_S$$

and then

$$t(v_C) = - RC \ln \frac{v_C}{V_S} = RC \ln \frac{V_S}{v_C} $$

Answer 2

Vs is best described in this equation as "the voltage at time zero" not "the source voltage." Considering that V_c is "the voltage across the capacitor" it's actually a time dependent variable, so expressing it as such might help you understand it.

$$V_c(t) = V_c(0) * e^{-\frac{t}{RC}}$$

Answer 3

"However, using the oscillator period equation T = 0.5RC, I get 5 seconds. What's the right way?"

This depends on the oscillator design. You need to know the threshold voltages at which it flips over and use these values in your equation. In the design below this is set by R1,R2. Different designs have different threshold settings though.