Voltage Controlled Butterworth Hi-Pass Filter using a Transconductance Op Amp?

Question

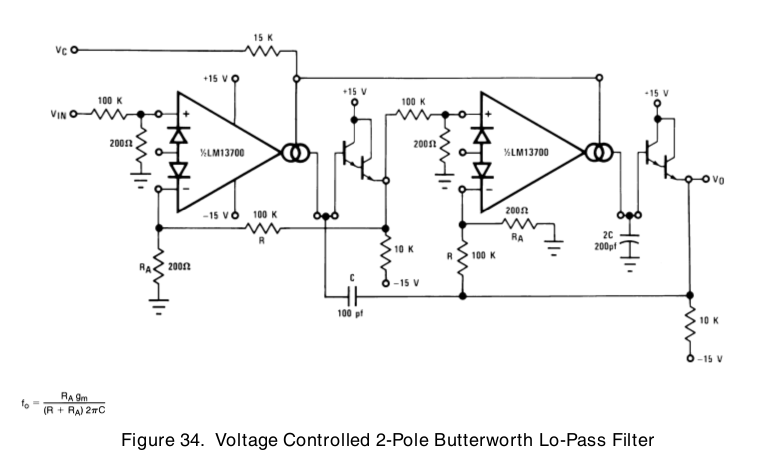
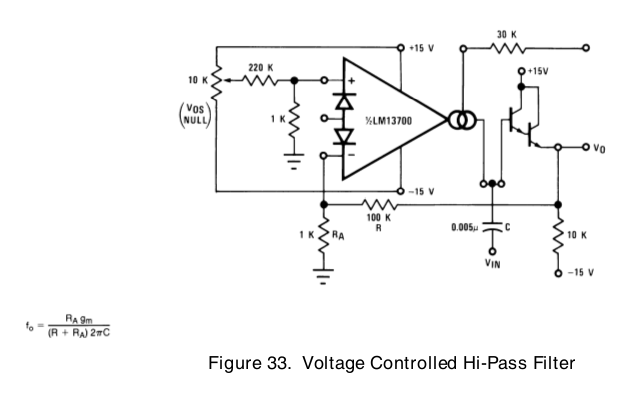
In the Texas Instruments datasheets for the LM13700 Transconductance Op Amp (as well as the earlier National Semiconductor versions) there's been an application note showing a voltage-controlled 2 Pole Butterworth low pass filter (in the TI datasheet this is shown in Figure 34 on page 15):

I'm using that filter but would like a symmetrical high pass filter (likely using the same RC values as the low pass). I basically need a balanced pair of voltage-controlled low and high pass filters.

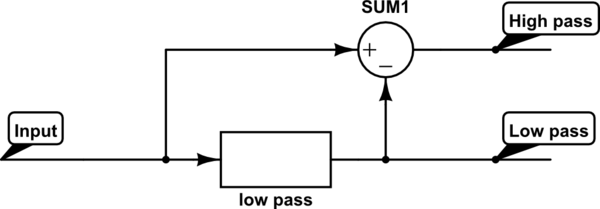
Does anyone know either of an existing schematic (in a book or online) or otherwise how I'd design an equivalent voltage-controlled 2-Pole Butterworth high pass filter? I've tried diagramming it and can't figure out, apart from the obvious bit of connecting the output of the first stage to the second, where the feedback is between stages. This is complicated by the fact that the high pass filter (Figure 33) uses the juncture between the output and buffer input (pins 5 and 7) as the source input:

I suppose the easiest way to approach this would be to ask: what changes would I make to the existing low-pass filter (Figure 34) to make it high-pass? This would seem straightforward were it not for my own limitations. [Oh, and apologies by way of explanation: I'm not an engineer but likewise not exactly new to analog circuitry, but I am to use of transconductance op amps.]

If it's any help, this is for audio range frequencies with a +-9VDC supply.

Thanks very much for any assistance.

Answer 1

I assume that you are not forced to use the structure as shown in your diagram (for lowpass filters)? In the following, I give you the link to one of the classical inventory articles on OTA filters:

http://class.ece.iastate.edu/vlsi2/docs/Linked%20Publications/1985-03-ICADM-RG.pdf

With reference to Fig. 7 in this paper, I recommemd to choose on of the structures which can be used for lowpass or highpass second-order functions. Formulas how to control the various parameters are also given.

Answer 2

You can use filters subtractively....

Take your original signal and produce the low pass output version using the circuit in your question. Then, take your original signal and subtract the low pass output from it. What you are left with (as a new signal) is the high pass version.

So what you get is two outputs; one is low-pass with a cut-off frequency at (say) 1kHz and the other is a high pass with cut-off frequency also locked-in to 1kHz - if you lower the cut-off frequency of the LP filter then the HP also lowers its cut-off.

^{simulate this circuit – Schematic created using CircuitLab}