Is there a way to mathematically express transformers in series? I'm curious if I can use the transformer equation to express this, but I'm not sure how to handle it. Would it be as simple as, say, treating the n transformers in series as just one transformer like: $$\frac{V_{P0}+...+V_{Pn}}{V_{S0}+...+V_{Sn}}=\frac{T_{P0}+...+T_{Pn}}{T_{S0}+...+T_{Sn}}$$
Where V is voltage, the subscripts p and s are primary and secondary, and T are turns of a winding.
This question is highly related to this one, raised by the same person and my answer is virtually the same so, I expect one or the other to be marked as duplicate.
The main problem with putting transformer primaries in series is that even with supposedly identical units, you cannot expect the magnetization inductance to be the same. This means, that on light or no-loads, the primary voltages could be 50% different when wired in series. You have to treat the transformer as it's equivalent circuit demands: -
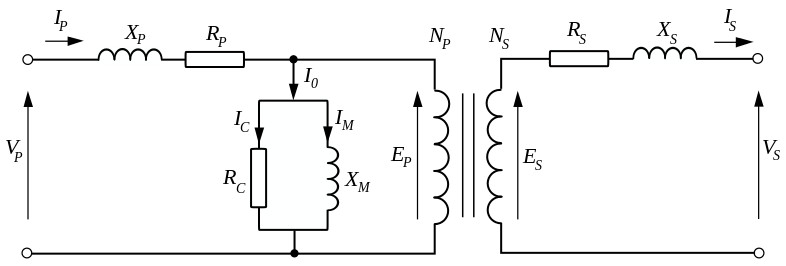
Forget the secondary for now. In ignoring the secondary you can ignore the perfect transformer symbol at the heart of the equivalent circuit. This means you are left with Xp, Rp, Rc and Xm. Because Xp and Rp are insignificant under no-load conditions you are left with the primary of the transformer looking like Xm in parallel with Rc.
These are the magnetization inductance and core losses respectively and you: -
CANNOT EXPECT THESE TO BE IDENTICAL for any two transformers that otherwise have the same model number.
This basically means the formulas in the question are too simple to merit further consideration. You can only use formulas like this when both transformer windings share the same core but, that then becomes a single transformer scenario.
