3 phase AC Voltage controller question

Question

Hello I have a question regarding the operating characteristics of 3 phase AC voltage controllers, the images describe the relation in between Van and (VAC,VAB,VBC)

The last image shows the resulting waveform when the respective thyristors are conducting (on), however i am confused as to why there is a voltage of zero when 2,3 and 5,6 are on.

I drew in green what i would expect the waveform to look like at that stage.

If anybody with experience in power electronics could explain this, it would be greatly appreciated.

thanks

Answer 1

The A to neutral voltage is zero when neither T1 nor T4 is conducting. When T2 & T3 or T5 and T6 are conducting, neither T1 nor T4 is conducting. It might help to diagram all three of the phase voltages and all of the conduction paths. The T4, T5, T6 gate sequence repeats after the third period of conduction that is numbered in the diagrams.

Answer 2

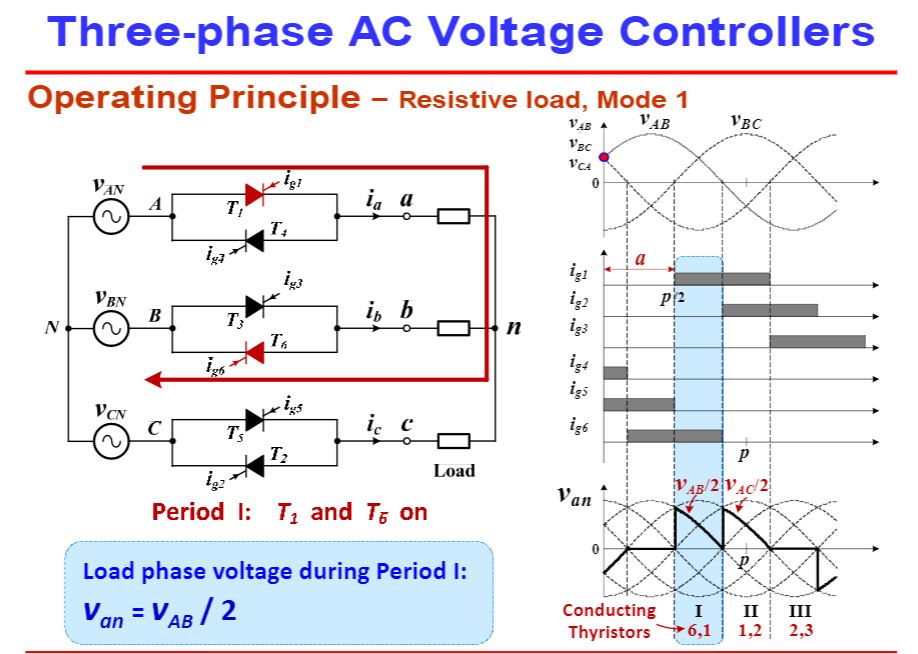
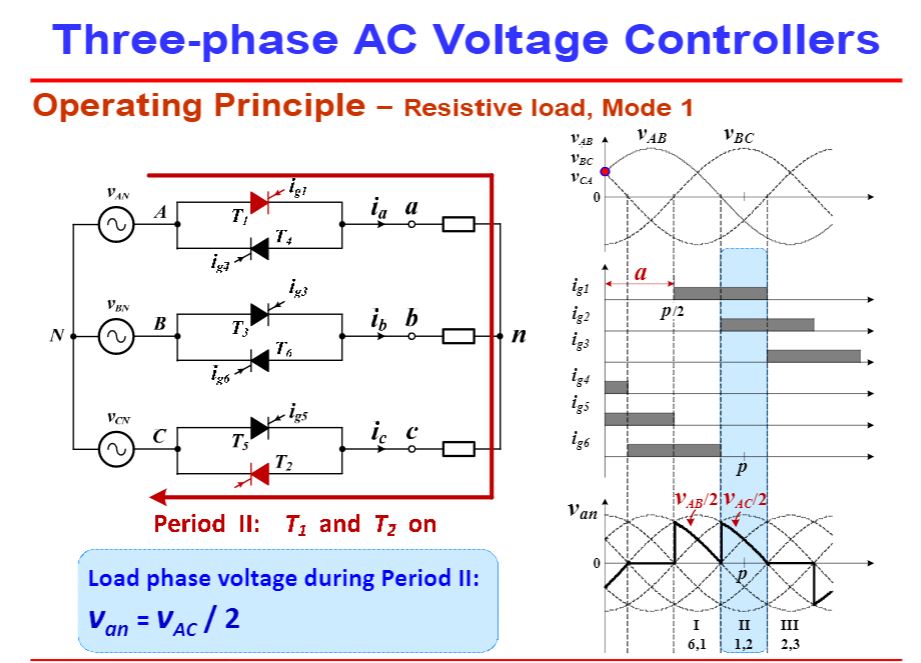
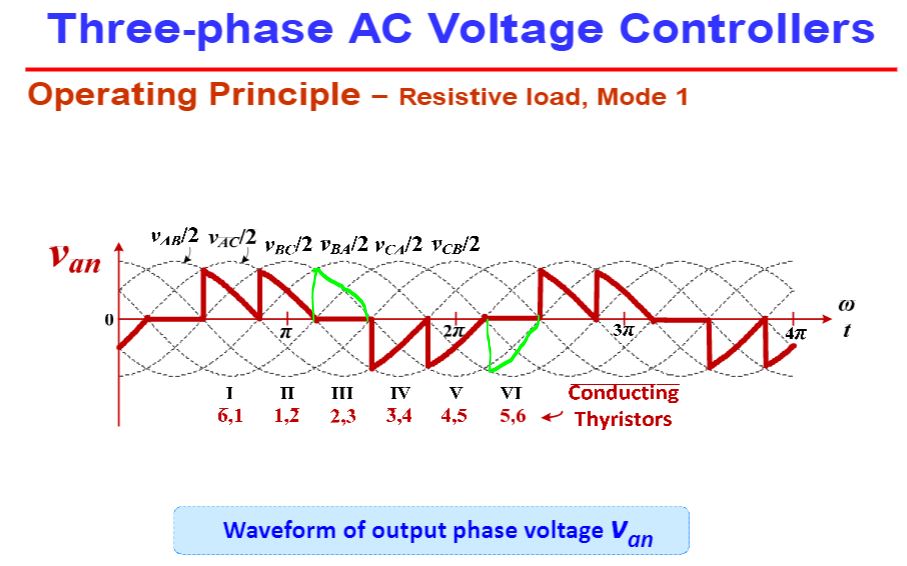
First, realize these observations:

1. Applying Ohm's law to the resistor in phase a: \$ v_\text{an} = R \, i_\text{a} \$. So, if \$ i_\text{a} = 0 \$ at some instant, then \$ v_\text{an} = 0 \, R = 0 \$ too. And if \$ i_\text{a} \ne 0 \$, then \$ v_\text{an} \ne 0 \$.
2. Only one SCR of each parallel group (1 and 4; 3 and 6; 5 and 2) can conduct at a given instant. So \$S_1\$ and \$S_4\$ can't be ON simultaneously.
3. The resistor in phase a is in series with the parallel group of SCR's 1 and 4. So, if at some instant those SCR's are both OFF, there won't be current in the conductor of phase a nor in that resistor. Then, by Ohm's law, \$ v_\text{an} = 0 \$.

Thus, if \$ S_1 = S_4 = \text{OFF} \$ then \$ i_\text{a} = 0 \implies v_\text{an} = 0 \$ for some instant. Also, if \$ S_1 = \text{ON} \implies S_4 = \text{OFF} \$ then \$ i_\text{a} \ne 0 \implies v_\text{an} \ne 0\$; if \$ S_1 = \text{ON} \implies S_4 = \text{OFF} \$ then \$ i_\text{a} \ne 0 \implies v_\text{an} \ne 0\$.

Going back to the last image you posted, in the intervals I, II, IV, V, the voltage \$ v_\text{an} \$ is not zero because either \$S_1\$ or \$S_4\$ are conducting. But for the intervals III, VI, which is where you have the doubt, neither \$S_1\$ nor \$S_4\$ are conducting, so \$ v_\text{an} \$ is zero.