Line voltage and phase voltage question

Question

​

In the question, why couldn't I just find Line current from Line Voltage?

Is not Line Current=Line Voltage/Impedance?

I know line voltage and line current aren't in phase, that the angle between line voltage and line current is 30 + theta, where theta is the angle current is lagging by.

But

1. How do I find theta?
2. How do I utilise it once I've found it out?

I'm really only lookin for an alternative solution to the question

Answer 1

I'm really only lookin for an alternative solution to the question

Unfortunately, you are looking for an easier solution where none exist.

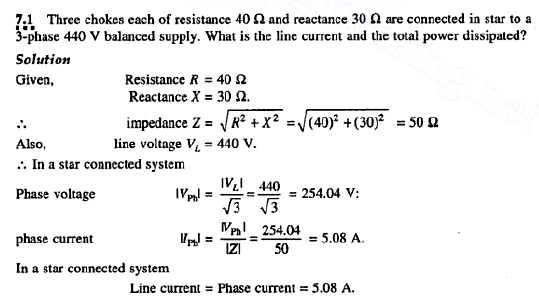
In the question, why couldn't I just find Line current from Line Voltage?

Is not Line Current=Line Voltage/Impedance?

Each of the three line voltages appears across two limbs of the 3 phase load and the impedance that the 2 limbs presents to the line is not simply 2(R + jwL); it's more complex than that because the junction of the 2 limbs of the 3 phase load is neutral for a balanced load/supply.

Hence, if you think about it a bit more it's a lot more sensible to calculate the phase voltage. The question delivers the hints; the supply is balanced and so is the load therefore, phase voltage is precisely line voltage divided by \$\sqrt3\$.

That's not too hard is it?

And, each individual phase voltage appears across each limb of the load hence, current is phase voltage divided by limb impedance. And that current can be called phase current or line current because the load is star-connected. If it were delta connected then it's a different matter.

So, your worked example calculates phase voltage (250 volts) and phase (limb) impedance and derives a value of (phase or line) current of 5.08 amps.

How do I find theta?

You don't need to find theta to calculate power; for each limb (phase) it is \$5.08^2\$ X 40 watts. Then, because there are three loads (each with the same power dissipation) you multiply the single phase power by 3 to get total star power.