0
\$\begingroup\$

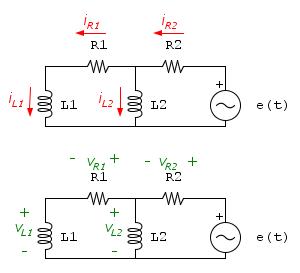
I have a problem with this circuit

enter image description here

I can't write the status equation because the system that characterizes the circuit is coupled respect to derivatives of the difference of rings currents.

Let $$i_1:=i_{R_1}=i_{L_1}$$ $$i_2:=i_{R_2}$$ so, applying counterclockwise the KVLs to the two rings we obtain

$$\begin{cases} L_1\frac{\text{d}}{\text{d}t}i_1-L_2\frac{\text{d}}{\text{d}t}[i_2-i_1]+R_1 i_1=0 \\ L_2\frac{\text{d}}{\text{d}t}[i_2-i_1]-e+R_2 i_2=0 \\ \end{cases}$$

or, equivalently

$$\begin{cases} \frac{\text{d}}{\text{d}t}i_1=-\frac{R_1}{L_1+L_2} i_1+\frac{L_2}{L_1+L_2}\frac{\text{d}}{\text{d}t}i_2\\ \frac{\text{d}}{\text{d}t}i_2=-\frac{R_2}{L_2}i_2+\frac{\text{d}}{\text{d}t}i_1+e \\ \end{cases}$$

but now I don't know how to proceed to determinate the vectorial status equation $$\dot{x}=Ax+Bu$$

\$\endgroup\$
4
  • \$\begingroup\$ Just use substitution (i.e. replace \$di_2/dt\$ from the first equation with the second equation). You will be able to represent the currents derivatives in terms of currents and \$e\$. \$\endgroup\$
    – Vicente Cunha
    Commented May 2, 2016 at 12:23
  • \$\begingroup\$ @Vicente Cunha, that will still leave \$i_2\$ in the equation. \$\endgroup\$
    – Chu
    Commented May 2, 2016 at 12:27
  • \$\begingroup\$ @Chu \$x\$ is a vertical vector with both \$i_1\$ and \$i_2\$; should I expand these comments to an answer? \$\endgroup\$
    – Vicente Cunha
    Commented May 2, 2016 at 12:45
  • \$\begingroup\$ @Vicente Cunha, state space form would be a very useful answer \$\endgroup\$
    – Chu
    Commented May 2, 2016 at 13:14

1 Answer 1

Reset to default
1
\$\begingroup\$

Starting from your equations:

$$\begin{cases} \frac{\text{d}}{\text{d}t}i_1=-\frac{R_1}{L_1+L_2} i_1+\frac{L_2}{L_1+L_2}\frac{\text{d}}{\text{d}t}i_2\\ \frac{\text{d}}{\text{d}t}i_2=-\frac{R_2}{L_2}i_2+\frac{\text{d}}{\text{d}t}i_1+e \\ \end{cases}$$

Substitution of second equation into first equation, and of first equation into second:

$$\begin{cases} \frac{\text{d}}{\text{d}t}i_1=-\frac{R_1}{L_1+L_2} i_1+\frac{L_2}{L_1+L_2}\left(-\frac{R_2}{L_2}i_2+\frac{\text{d}}{\text{d}t}i_1+e\right) \\ \frac{\text{d}}{\text{d}t}i_2=-\frac{R_2}{L_2}i_2+\left(-\frac{R_1}{L_1+L_2} i_1+\frac{L_2}{L_1+L_2}\frac{\text{d}}{\text{d}t}i_2\right)+e \\ \end{cases}$$

Rewriting equations:

$$\begin{cases} \frac{\text{d}}{\text{d}t}i_1\times\left(1-\frac{L_2}{L_1+L_2}\right)= -\frac{R_1}{L_1+L_2}i_1-\frac{R_2}{L_1+L_2}i_2+\frac{L_2}{L_1+L_2}e\\ \frac{\text{d}}{\text{d}t}i_2\times\left(1-\frac{L_2}{L_1+L_2}\right)= -\frac{R_1}{L_1+L_2}i_1 -\frac{R_2}{L_2}i_2 + e\\ \end{cases}$$

$$\begin{cases} \frac{\text{d}}{\text{d}t}i_1\times L_1= -R_1i_1-R_2i_2+L_2e\\ \frac{\text{d}}{\text{d}t}i_2\times L_1= -R_1i_1 -\frac{(L_1+L_2)R_2}{L_2}i_2 + (L_1+L_2)e\\ \end{cases}$$

Matrix representation:

$$ A = \frac{1}{L_1}\left( \begin{array}{cc} -R_1 & -R_2 \\ -R_1 & -\frac{(L_1+L_2)R_2}{L_2} \end{array} \right) $$

$$ B = \frac{1}{L_1}\left( \begin{array}{c} L_2 \\ L_1+L_2 \end{array} \right) $$

\$\endgroup\$
0

Your Answer

By clicking “Post Your Answer”, you agree to our terms of service and acknowledge you have read our privacy policy.

Not the answer you're looking for? Browse other questions tagged or ask your own question.