Explanation of the Node-Voltage Method equation in specific circuit

Question

I'm looking at this circuit in my textbook: 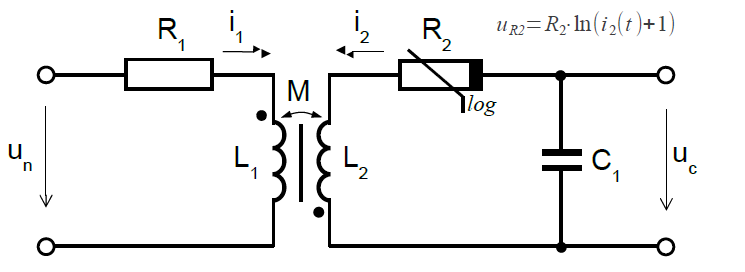There are also equations for this circuit:

\$ u_n(t) = R1\cdot i_1(t) + L_1\frac{di_1(t)}{dt}-M\frac{di_2(t)}{dt} \$

\$u_C(t) = -R_2\ln(i_2(t)+1)-L_2\frac{di_2(t)}{dt}+M\frac{di_1(t)}{dt}\$

I totaly agree with the first equation. But I don't understand, why the second equation looks like this. I would wrote it as: \$u_C(t) = R_2\ln(i_2(t)+1)+L_2\frac{di_2(t)}{dt}-M\frac{di_1(t)}{dt}\$

I see the current \$i_2\$ comming in the opposite direction than voltage \$u_C\$ same as the current \$i_1\$ is comming in the opposite direction than voltage \$u_n\$. So what is the differnce? Is it something about the \$u_n\$ being the source and \$u_C\$ consumed voltage? Could anybody explain?

Answer 1

The only difference between the given equation for \$u_C(t)\$ and your equation is the right hand side is multiplied by -1. Since the dot of the primary side of transformer is at the top and the dot for the secondary side is at the bottom, the polarity of the secondary side will be opposite that of the primary side.

http://en.wikipedia.org/wiki/Polarity_(mutual_inductance)

Had both the dots been at the top, your equation would be right.

Answer 2

Here is simple way to understand.

Dot indicates that the instantaneous voltages increase or decrease together at those points.

So if both dots are on top, the polarity is same both sides or else its opposite.Polarity in AC domain means imagine how the polarity of voltage is at a particular instant of time.

Now the way you write equation depends -

• on directions assumed in diagram of current
• and polarity assumed of voltages obtained using dot conventions of induced voltages.