Art Of Electronics - 2nd Edition - Absolute Value of a Complex Number - Math

Question

In this section, I am struggling with the line

Abs(V) * Abs(I) = V0^2 / [blah blah]

I am not seeing how the math simplifies to that term. After looking up the absolute value of a complex number, and seeing that it is basically Pythagoras theorem on the real and imaginary axis. I just don't see the simplification for the line above power faster =

Can someone help? I assume it is correct?

Thanks

Answer 1

It's not quite "absolute value" -- it's "magnitude", and is defined to be the square root of the number times it's complex conjugate -- \$ \sqrt{ X \cdot X^*}\$. This is what Matlab does when it takes the absolute value of a complex number.

To take the complex conjugate, just replace the \$j\$ with \$-j\$.

When you do the multiplication, you'll see that all of the complex terms (i.e., odd powers of j) will end up cancelled out through subtraction, and of course even powers of j are real.

If you do this, and develop a little more facility with complex numbers, along with remembering that \$j^2= -1\$ you won't have to remember any tricks or formulas or theorems. It will work all the time.

So, Let's look at the magnitude of Z

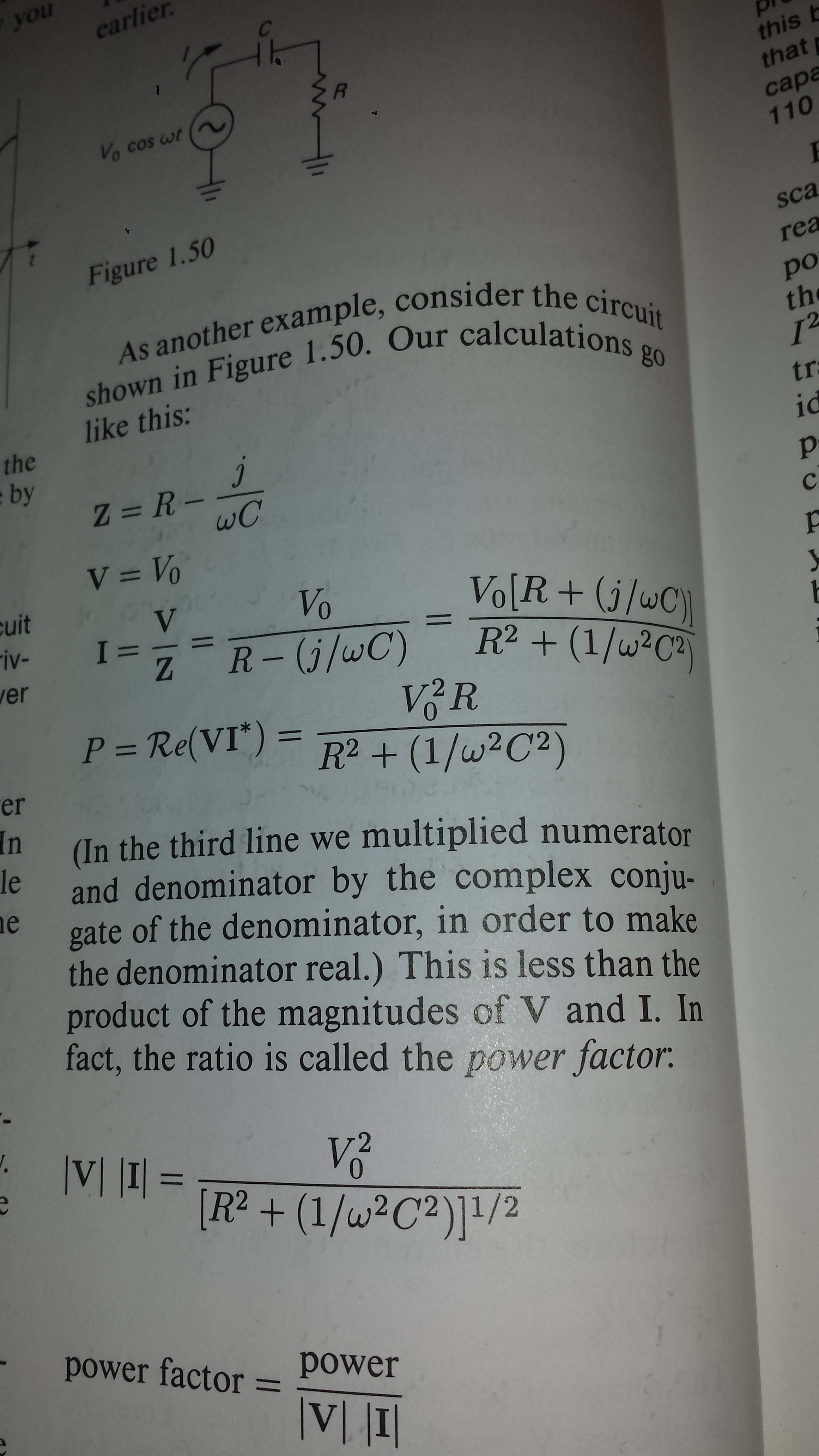
$$ Z= R- \frac{j}{\omega C}$$ $$ Z^*= R + \frac{j}{\omega C}$$ $$|Z|=\sqrt{ZZ^*}= \sqrt{R^2 - \frac{jR}{\omega C}+\frac{jR}{\omega C}+ \frac{1}{\omega^2C^2}}= \sqrt{R^2+ \frac{1}{\omega^2C^2}}$$

Now, lets look at your example

$$ I = \frac{V_o}{Z}= \frac{V_o}{R-\frac{j}{\omega C}}$$

Multiply the numerator and denominator by the complex conjugate of the denominator (i.e., multiply by 1, which is always allowed): $$\frac{V_o}{Z}= \frac{V_o}{R-\frac{j}{\omega C}}\cdot \frac{R+\frac{j}{\omega C}}{R+\frac{j}{\omega C}} =\frac{ V_o \left[ R+\frac{j}{\omega C} \right]}{R^2 + \frac{1}{\omega^2 C^2}}\text{,}$$

which is your middle equation. Note that the denominator is a real number, so you can just factor that out if you need to go on and figure out the magnitude of the numerator.

So all of this, combined with the understanding that all of this complex math will yield a vector in the complex plane, and that the magnitude is the length of the vector, and the angle is the angle of clockwise rotation (i.e., \$\tan^{-1}\left( \frac{\mathbb{I}\text{m}}{\mathbb{R}\text{e}}\right) \$) will answer all of your series of recent questions on the manipulation of these sorts.

Answer 2

As you discovered, you use the Pythagorean theorem to get the absolute value (aka modulus aka magnitude). Do this to the I= line and then it's just algebra to get the result:

$$ |I| = \left| {\frac {V_0} { R - (j/\omega C)}} \right| = \frac { V_0} { |R - (j/\omega C)|} = \frac {V_0} {\sqrt{ R^2 + 1/\omega^2 C^2}} $$

Multiply by |V|=V0 to get the result |V||I| = ... that you're looking for.

Answer 3

Yes it's correct.

Here's what I think you're missing : capacitors and inductors are energy storage devices; the power you put into the capacitor is reclaimed later.

But the power you put into the resistor is dissipated as heat.

So the real power is the component of the VI product lying along the real (R) axis, while the "VA rating" is the hypotenuse; and the power factor is the ratio of the two - or the cosine of the phase angle between them.