LC circuit impulse response

Question

I have a trouble solving an assignment in the online course I'm following. The assignment asks some questions with respect to this circuit:

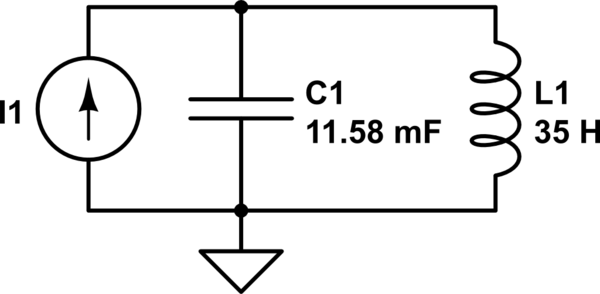
^{simulate this circuit – Schematic created using CircuitLab}

where the current source puts out an impulse of area \$A = \frac{2}{\pi}\$ Coulomb at time \$t=9s\$ The differential equation for this circuit is given already solved and it's

$$\frac{d^2i_L(t)}{dt^2} + \frac{1}{LC}i_L(t) = \frac{A}{LC}\delta(t - 9.0)$$

I've already answered some questions (like \$i_L\$ and \$v_C\$ just before the impulse and \$i_L\$ just after the impulse) and the answers are correct. In particular, the system accepted my \$-54.9768V\$ as the answer for the \$v_C\$ just before the impulse.

But for the \$v_C\$ just after the impulse the system doesn't accept my answer which is (rounded to four decimal places) \$0.0318V\$. I got this answer using two ways (both times the same result).

The first is: supposing that for a current impulse the capacitor should behave like a short circuit, all the current should go through the capacitor and deposit all the charge on it. The deposited charge should add to the voltage already on the capacitor an increment \$\Delta V = \frac{A}{C}\$.

The second way is: find the response to a current pulse of width \$\Delta t\$ and height \$\frac{A}{\Delta t}\$, which happens at the \$t=9\$. From this response we find then \$v_C\$ at time \$t=9 + \Delta t\$ and then we find limit of this value when \$\Delta t \to 0\$. The result is the \$v_C\$ just after the impulse.

So, as mentioned above, both ways give me the same answer which seems to be wrong and I don't see where is my error. Please, help me with this.

Thanks

Answer 1

It appears to me that you were given a 1A DC current source at t=0 ( but did't tell us) and LC oscillates with a 4 second cycle. Then an impulse is applied at 9s.

I get ΔV=54.9758 (2/π/11.58mF) which give a net result of -1mV.

But I would call it zero.

It is a 4 second LC cycle so t=9s is the same as t=1s, 5s.