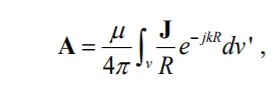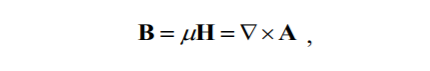Especially in antenna theory books, they employ magnetic vector potential. Why is the vector potential concept used?
For example the magnetic vector potential is written as:
And the magnetic field in terms of this potential:
But what is the motivation or need to introduce the vector potential concept here? And is the magnetic vector potential derived from Maxwell equations?
The magnetic vector potential is defined to be analogous to the electric scalar potential for a point charge at distance R which is written,
\begin{equation} V = \frac{1}{4\pi\epsilon } \frac{Q}{R} \end{equation}
This simplifies many problems that would otherwise require using the electric field which is a vector. Similarly, the magnetic vector potential is defined
\begin{equation} \mathbf{A} = \frac{\mu}{4\pi} \frac{Q\mathbf{v}}{R} \end{equation}
This has to be a vector as magnetic fields are produced by moving charge, but this is still simpler than dealing with the magnetic field as that involves taking the cross product of the velocity vector with a position vector.
Once these potentials have been found for a given situation, the electric and magnetic fields can be found by a differential operator, curl in the case of the magnetic field. This ends up being much easier than directly solving for the fields.
