Magnetic Monopoles and Magnetic Current Densities

Question

I see that in electronic engineering the Maxwell equations are written with also some terms which represent the magnetic charge and the magnetic current densities. I was told they are inserted only to get symmetrical equations since they physically do not exist. enter link description here

But, how can this operation be correct, since it is and arbitrary addition of not physical terms? It is like modifying a correct physical law by adding arbitrary terms to one member: it is a correct operation if and only if these terms are 0.

Answer 1

Math

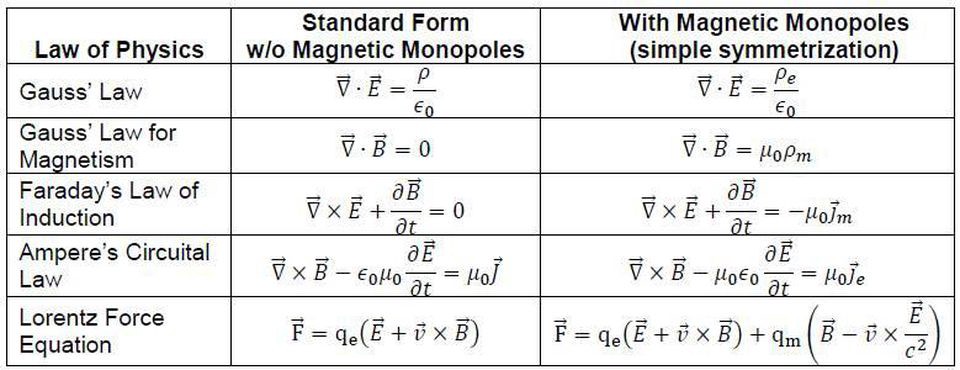
As to why this is allowed: They follow from some of the math. When you solve the equations (you can actually get both of Gauss' laws from Faraday's and Ampere's laws), you end up with a constant, which is the charge. Think of it this way: Gauss' laws state that the total amount flow of field into/out a sphere equals the amount of 'sinking/sourcing power' inside of that sphere. The left part of the equation, the \$ \overrightarrow{\nabla} \cdot \overrightarrow{E}\$ and \$\overrightarrow{\nabla} \cdot \overrightarrow{B}\$ are that 'total flow into/out of the sphere'. The \$ \frac{\rho_e}{e_0} \$ and \$ \mu_0\rho_m \$ are the 'sink/source power'.

So from this mathematical perspective, it makes sense to write them. Just because we have not found them (and thus, the \$\rho_m\$ will always be zero), doesn't mean it is wrong to write them. If they do exist, it would make a lot of sense for the 'full' equation to be correct.

Duality

In addition to this, there is another reason we might want to write them down: There is a concept in electromagnetism, which is called 'Duality' (link to wikipedia page). I won't list all of the 'properties' here, but in short, this concepts states that you can exchange 'magnetic' and 'electric' concepts, and still keep valid equations (only if you exchange all the concepts - you can't choose just a few).

But, there is a problem with the electric charge - in the standard set of Maxwell equations, there is nothing to exchange with electric charge. This is where magnetic monopoles come in - we can exchange them with the electric charge and use this duality.

What is the use of this? It can be very effective for the design of new structures. Take for example the simple electrical dipole antenna we know and love. If we apply the duality principle to this antenna, we know we would have an antenna that performs the same, but would be a magnetic loop instead. And this is exactly what a loop antenna is - the magnetic dual to the electric dipole. The same applies to making more complicated structures.